If y=ekt then dydt will be (A) ekt(B)ektk(C)tekt(D)kekt
Step by step video & image solution for If y=e^(kt) then dy/dt will be (A) e^(kt) (B) e^(kt)/ k (C) te^(kt) (D) ke^(kt) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If y=Ae−ktcos(pt+c), then prove that d2ydt2+2kdydx+n2y=0 , where n2=p2+k2
Ap2−k2Bp2Ck2Dp2+k2A particle moves in the x-y plane under is influence of a force such that its linear momentum is →P(t)=A[ˆicos(kt)−ˆjsin(kt)], where A and k are constants Angle between the force and the momentum is:
A0∘B30∘C45∘D90∘In the Wilhelmey equation of a first order reaction ct=c0e−kt. If the initial concentration c0 is increased m times, then
AThe value of k will increase m timesBThe value of k will decrease m timesCThe value of k will remain unchangedDNone of these
The total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
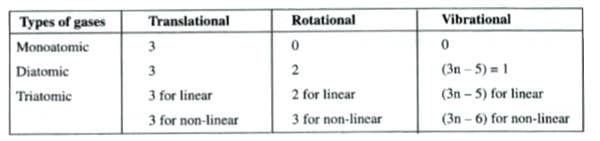
Where 'n' is atomicity of gas.
How many total degrees of freedom are present in H2 molecules in all types of motions ?
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
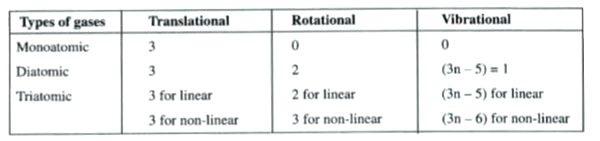
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
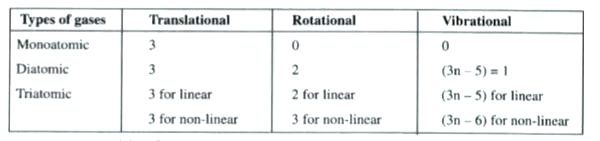
Where 'n' is atomicity of gas.
The rotational kinetic energy of H20 molecule is equal to
View SolutionConsider the two statements Statement-l: y=sin kt satisfies the differential equation y''+9y=0. Statement 2 : y= ekt satisfy the differential equation y''+y'−6y=0. The value of k for which both the statements are correct is (A)−3 (B)0 (C) 2 (D)3
View SolutionIn the Wilhelmey equation of a first order reaction ct=c0e−kt. If the initial concentration c0 is increased m times, then
View Solution
