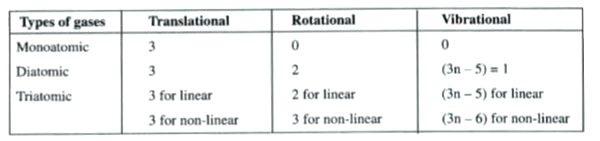
The total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
![The total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are E(K(x)), E(K(y)), E(K(z)) Total K.e =E(K(x)) + E(K(y)) + E(K(z)) Since the motion of molecule is equally probable in all the three directions, therefore E(K(x)) = E(K(y)) = E(K(z)) =1/3 E(K) =1/3 xx 3/2 kT = 1/2 kT, where k =R/N(A) = Botzman constant. K.E. = 1/2 kT per molecule or =1/2 RT per mole. In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom. Vibration energy =2 xx 1/2kT =2 xx 1/2RT [therefore two degrees of freedom per mole] If the gas molecules have n(1) translational degrees of freedom, n2 rotational degrees of freedom and n(3) vibrational degrees of freedom, that total energy = n(1)[(kT)/2] + n(2) [(kT)/2] + n(3) [(kT)/2] xx 2 Where 'n' is atomicity of gas. The rotational kinetic energy of H20 molecule is equal to](https://d10lpgp6xz60nq.cloudfront.net/physics_images/AKS_AO_CHE_V01_P2_C02_E02_087_Q01.png)
Where 'n' is atomicity of gas.
The rotational kinetic energy of H20 molecule is equal to
32kT
12kT
kT
2kT
The correct Answer is:A
Step by step video & image solution for The total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are E_(K_(x)), E_(K_(y)), E_(K_(z)) Total K.e =E_(K_(x)) + E_(K_(y)) + E_(K_(z)) Since the motion of molecule is equally probable in all the three directions, therefore E_(K_(x)) = E_(K_(y)) = E_(K_(z)) =1/3 E_(K) =1/3 xx 3/2 kT = 1/2 kT, where k =R/N_(A) = Botzman constant. K.E. = 1/2 kT per molecule or =1/2 RT per mole. In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom. Vibration energy =2 xx 1/2kT =2 xx 1/2RT [therefore two degrees of freedom per mole] If the gas molecules have n_(1) translational degrees of freedom, n_2 rotational degrees of freedom and n_(3) vibrational degrees of freedom, that total energy = n_(1)[(kT)/2] + n_(2) [(kT)/2] + n_(3) [(kT)/2] xx 2 Where 'n' is atomicity of gas. The rotational kinetic energy of H20 molecule is equal to by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
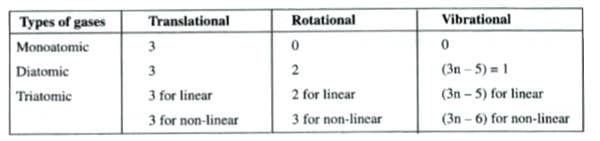
The total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
Where 'n' is atomicity of gas.
How many total degrees of freedom are present in H2 molecules in all types of motions ?
View SolutionDerive an expression for the mean K.E. of a gas molecule.
View SolutionTotal kinetic energy of molecules of a gas sample varies K.E.=74nRT. This sample contains
View SolutionThe magnitude of binding energy of the satellite is E and kinetic energy is K. The ratio E/K is
View SolutionWhich of the following is correct if E0x+x>E0y+y>E0z+z
View SolutionThe Threshold energy is given as E0 and radiation of energy E falls on metal then K.E is given as
View SolutionThe ratio of average translational K.E. and average rotational K.E. of a linear polyatomic molecule at temperature T is:
View SolutionIf EpandEk represent potential energy and kinetic energy respectively of an orbital electron, then, according to Bohr.s theory :
View SolutionIf the area bounded by y=x2andy=21+x2 is (K1π−K23) sq. units (where K1,K2∈Z), then the value of (K1+K2) is equal to
View Solution
