A block of mass m = 2 kg is attached to a spring whose spring constant is k = 8 Nm−1. The block slides on an incline for which μk=18 and θ = 37°. If the block starts at rest with spring unextended, what is its speed, in ms−1, when it has moved a distance d = 0.5 m down the incline ?
Step by step video & image solution for A block of mass m = 2 kg is attached to a spring whose spring constant is k = 8 Nm^(-1). The block slides on an incline for which mu_(k)=(1)/(8) and theta = 37°. If the block starts at rest with spring unextended, what is its speed, in ms^(-1), when it has moved a distance d = 0.5 m down the incline ? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
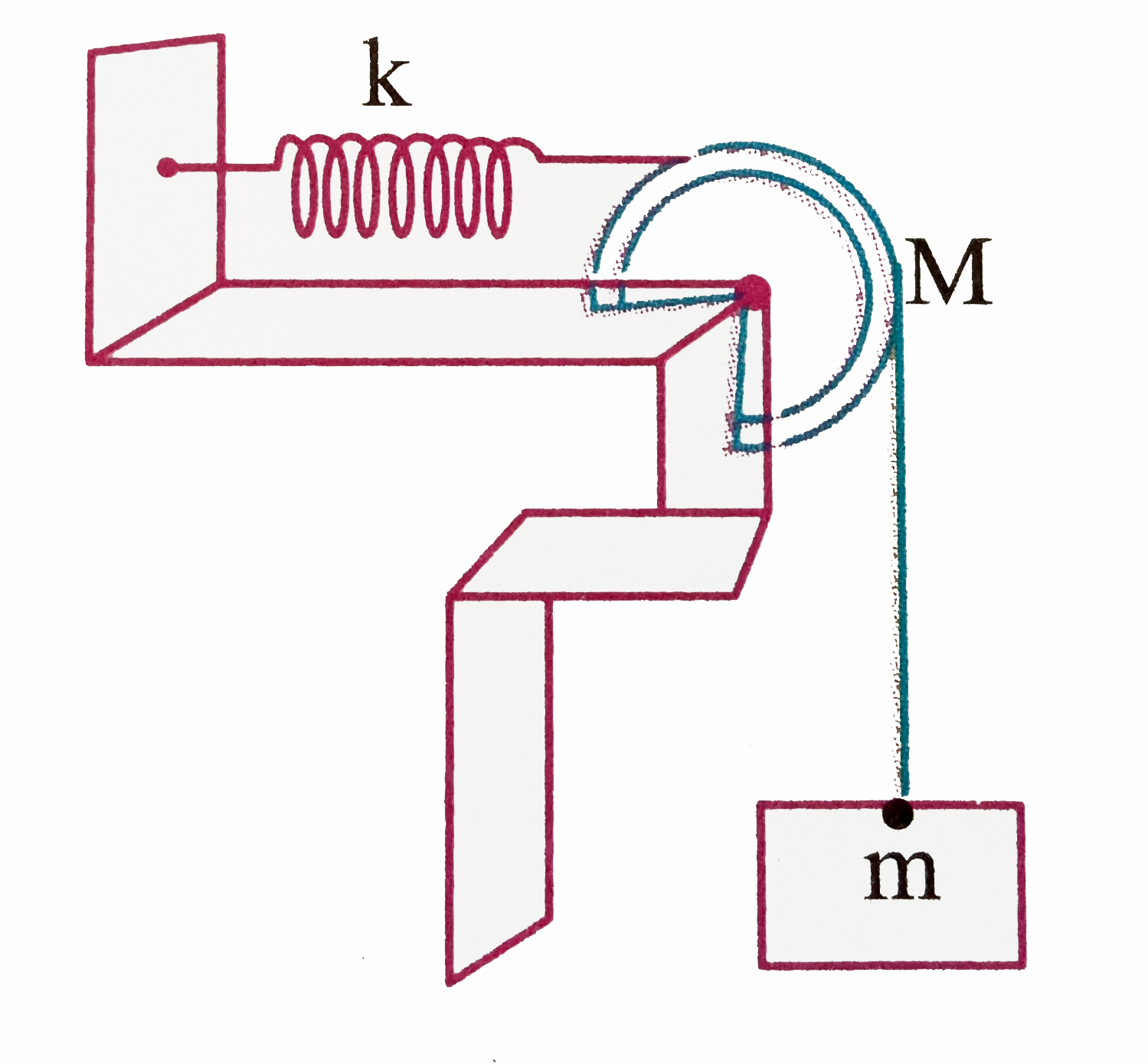
A block of mass m=4kg is attached to a spring of spring constant (k=32Nm−1) by a rope that hangs over a pulley of mass M=8kg If the system starts from rest with the spring unstretched, find the speed of the block after it falls 1m. Treat the pulley as a disc, so I=12MR2
 View Solution
View Solution
Consider a block of mass 6 kg is attached to a spring having constant 16 Nm−1 . If the block is at rest initially and then stretched by a constant force of 20 N on frictionless surface as shown in figure . Caclculate the speed of the block when it has moved through a distance of 0.2 m from its initial position . dView Solution
Knowledge Check
- Question 1 - Select One
A block of mass 'm' is attached to a spring in natural length of spring constant 'k'. The other end A of the spring is moved with a constat velocity v away from the block . Find the maximum extension in the spring.
 A14√mv2kB√mv2kC12√mv2kD2√mv2k
A14√mv2kB√mv2kC12√mv2kD2√mv2k - Question 2 - Select One

A block of mass m is attached to two unstretched springs of spring constant k, each as shown. The block is displaced towards right through a distance x and is released The speed of the block as it passes through the mean position will beAx√m2kBx√2kmCxmkDx2km - Question 3 - Select One
A block of mass m, attacted to a string of spring constant k, oscillates on a smooth horizontal table. The other end of the spring is fixed to a wall. The block has a speed v when the spring is at its natural length. Before coming to an instantaneous rest. If the block moves a distance x from the mean position, then
Ax=√mkBa=1v√mkCx=v√mkDx=√mvk
The block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.
 View Solution
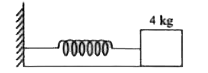
View SolutionA block of mass 4 kg while at rest is attached to an unstretched spring. The force constant of spring is 24Nm−1. If a constant horizontal force of 10 N is applied on the block, the spring gets compressed by 0.5m. What is the speed of the block at this point?
 View Solution
View SolutionA block whose mass is 2 kg is fastened on a spring whose spring constant is 100 Nm−1 . It is pulled to a distance of 0.1 m from over a frictionless surface and is released at t=0. Calculate the kinetic eneryg of the block when it is 0.05 m away from its mean position.
View SolutionIn the figure shown below , a block of mass 4 kg is attached to a spring constant 24 N/m . The coefficient og friction μ=0.5 . If the system is initially at rest and a constant horizontal force of 50 N is applied on the block , then calculate its speed when it is moved through a distance of 0.6 m .
 View Solution
View SolutionA block of mass m is attached with a massless spring of force constant k. The block is placed over a fixed rought inclined surface for which the coefficient of friction is μ=34 . The block of mass m is initially at rest . The block is mass M is released from rest with spring in unstretched state. The minimum value of M required to move the block up the plane is ( neglect mass of string and pulley and friction in pulley )
 View Solution
View Solution
