K represents the rate constant of a reaction when log K is p lotted again st 1/T (T=temperature) the graph obtained is a
curve
a straight line with a constant positive slope
a straight line with constant negative slope
a straight line with no slope
The correct Answer is:C
To solve the problem, we need to analyze the relationship between the rate constant (K) and temperature (T) using the Arrhenius equation. Here’s a step-by-step solution:
Step 1: Understand the Arrhenius Equation
The Arrhenius equation is given by:
K=Ae−EaRT
where:
- K is the rate constant,
- A is the pre-exponential factor,
- Ea is the activation energy,
- R is the universal gas constant,
- T is the absolute temperature.
Step 2: Take the Logarithm of Both Sides
To analyze the relationship in terms of logarithms, we take the logarithm of both sides:
logK=logA−Ea2.303RT
This can be rearranged to:
logK=−Ea2.303R⋅1T+logA
Step 3: Identify the Linear Form
The equation now resembles the linear form y=mx+c:
- Let y=logK
- Let x=1T
- The slope m=−Ea2.303R
- The y-intercept c=logA
Step 4: Analyze the Graph
From the equation, we can conclude:
- The graph of logK versus 1T is a straight line.
- The slope of the line is negative because −Ea2.303R is negative (since Ea and R are positive).
- The intercept is logA, which means the line does not pass through the origin.
Conclusion
The graph obtained by plotting logK against 1T is a straight line with a constant negative slope.
Final Answer
The correct nature of the graph is: A straight line with a constant negative slope.
---
The Arrhenius equation is given by:
K=Ae−EaRT
where:
- K is the rate constant,
- A is the pre-exponential factor,
- Ea is the activation energy,
- R is the universal gas constant,
- T is the absolute temperature.
To analyze the relationship in terms of logarithms, we take the logarithm of both sides:
logK=logA−Ea2.303RT
This can be rearranged to:
logK=−Ea2.303R⋅1T+logA
The equation now resembles the linear form y=mx+c:
- Let y=logK
- Let x=1T
- The slope m=−Ea2.303R
- The y-intercept c=logA
From the equation, we can conclude:
- The graph of logK versus 1T is a straight line.
- The slope of the line is negative because −Ea2.303R is negative (since Ea and R are positive).
- The intercept is logA, which means the line does not pass through the origin.
The graph obtained by plotting logK against 1T is a straight line with a constant negative slope.
The correct nature of the graph is: A straight line with a constant negative slope.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - EXERCISE -1 (C.W) (RATE OF REACTION & FACTORS)14videos
CHEMICAL KINETICS
- BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - EXERCISE -1 (C.W) (ORDER OF REACTION)14videos
CHEMICAL KINETICS
- BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - CHECK YOUR GRASP14videos
CHEMICAL KINETICS
- BOOK - NARAYNACHAPTER - CARBOXYLIC ACIDEXERCISE - All Questions288videos
CARBOXYLIC ACID
- BOOK - NARAYNACHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - ASSERTION & REASON TYPE QUESTIONS10videos
CHEMISTRY IN EVERYDAY LIFE
Similar Questions
The rate of a gaseous reaction is given by the expression k [A] [B]. If the volume of the reaction vessel is suddenly reduced to 1/4th of the initial volume, the reaction rate relating to original rate will be
View Solutionfor a reaction 2A+3B→Products, the rate law expression is given by rate = K(A)1(B)2 . The order of the reaction with respect to A,B and over all order of reaction ar
03:31View SolutionWhat is the instantaneous rate of a reaction.
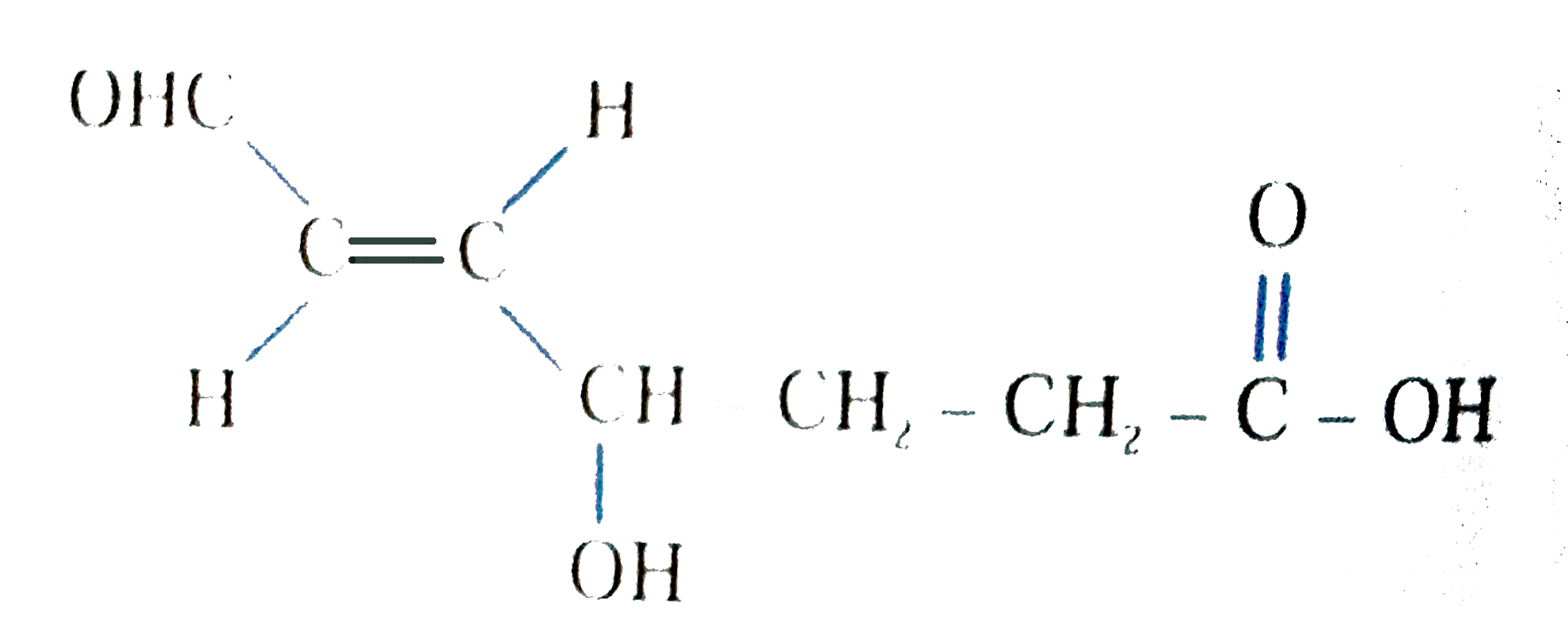
View SolutionProvide IUPAC name of the compound given below.
 06:07View Solution
06:07View Solution(A) Analgesics bring down body temperature during high fever.
(R ) Tetracyclin is a tranquillizer.03:31View SolutionGraph between log k and 1//T [where K is rate constant in s^(-1) and T is the temperature (in K) is a straight line with Hence, E_(a) will be
View SolutionThe rate constant 'k'. For a reaction varies with temperature 'T' according to the question. logk=logA−Ea2.303R(1T)
Where Ea is the activation energy. When a graph is plotted for logkvs1/T, a straight line with a slope of −4250 K is obtained. Calcualte Ea for this reaction.03:19View SolutionThe graph between log k and 1/T[K is rate constant (sec−1) and T the temperature (K)] is a straight line with OX=5andθ=tan−1(−12.303).Calculate the value of Ea is cal. ?
 03:51View Solution
03:51View SolutionRate constant k of a reaction varies with temperature according to the equation
logk = constant −Ea2.303R(1T)
where Ea is the energy of activation for the reaction . When a graph is plotted for log k versus 1T , a straight line with a slope - 6670 K is obtained. Calculate the energy of activation for this reaction02:17View SolutionThe rate constant 'k'. For a reaction varies with temperature 'T' according to the question. logk=logA−Ea2.303R(1T)
Where Ea is the activation energy. When a graph is plotted for logkvs1/T, a straight line with a slope of −4250 K is obtained. Calcualte Ea for this reaction.03:51View SolutionRate constant k of a reaction varies with temperature T according to the following Arrhenius equation.
logklogAEa2.303R(1T) where Ea is the activation energy . When a graph is plotted is or logk Vs 1T a straight line with a slope of −400K is obtained . Calculate the activation energy .02:04View SolutionGraph between logk and 1/T [k rate constant (s−1) and T and the temperature (K)] is a straight line with OX=5,θ=tan−1(1/2.303). Hence −Ea will be
 01:35View Solution
01:35View Solutionदर स्थिरांक k का तापमान T(R) के साथ विक्षेपण निम्न समीकरण द्वारा दर्शाया जाता है
logk=logA−Ea2.303RT
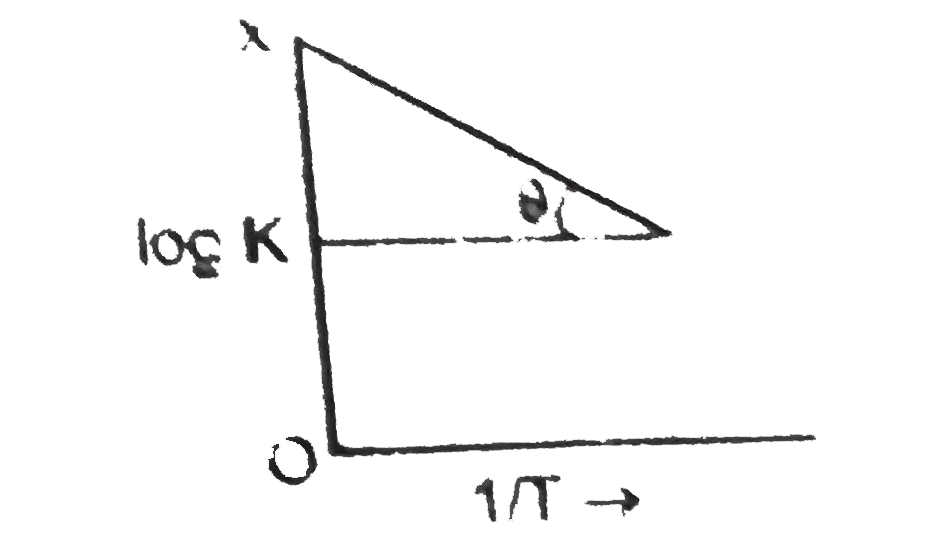
logk और 1T के बीच खींचा गया ग्राफ एक सीधी रेखा है, अतः कहा जा सकता है05:30View SolutionThe graph of log K versus 1/T is given below. Here x is equal to [Where K=rate constant and T=absolute temperature]
02:28View SolutionFor a class reaction, T is the rate constant at temperature, lim_(T to oo) The value of log K ____ is - (where A is the constant constant).
02:28View Solution

