Two triangles QPR and QSR, right angled at P and S respectively are drawn on the same base QR and on the same side of QR. If PR and SQ intersect at T, prove that PT×TR=ST×TQ.Solution in Tamil
Step by step video solution for Two triangles QPR and QSR, right angled at P and S respectively are drawn on the same base QR and on the same side of QR. If PR and SQ intersect at T, prove that PT xx TR= ST xx TQ. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - EXERCISE 4.2
17 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - EXERCISE 4.3
8 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - THINKING CORNER
2 Videos
- DEPARTMENTAL MODEL PAPER BOOK - FULL MARKSCHAPTER - DEPARTMENTAL MODEL PAPER EXERCISE - PART-IV
3 Videos
- MENSURATION BOOK - FULL MARKSCHAPTER - MENSURATION EXERCISE - ADDITIONAL QUESTIONS SOLVED (iii)
8 Videos
Similar Questions
In ΔABC, D and E are points on the sides AB and AC respectively such that DE∣∣BC
(i) If ADDB=34 and AC=15 cm find AE.
(ii) If AD=8x-7, DB=5x-3, AE= 4x-3 and EC=3x - 1, find the value of x.
View SolutionA man goes 18 m due east and then 24 m due north. Find the distance of his current position from the starting point ?
View SolutionCan we draw two tangents parallel to each other on a circle ?
View SolutionPQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T . Find the length TP .
View SolutionA vessel is in the form of a hemispherical bowl mounted by a hollow cylinder. The diameter is 14 cm and the height of the vessel is 13 cm. Find the capacity of the vessel.
View SolutionIn figure, PQR is a triangle in which PQ=PR and S is any point on the side PQ . Through S, a line is drawn parallel to QR and intersecting PR at T. Prove that PS=PT.
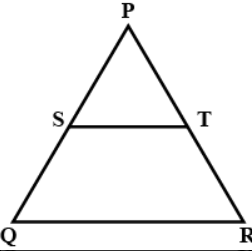
View SolutionPQR is a triangle is which PQ=PR and S is any point on the side PQ . Through S, a line is drawn parallel to QR and intersecting PR at T. Prove that PS=PT
In△PQR,wehavePQ=PR
View SolutionIn ΔPQR,Pq=PR and S is the mid-point of PQ. A line drawn from S parallel to QR, intersects the line PR at T. Prove that PS = PT.
View SolutionIn the adjoining figure, S and T are points on the sides PQ and PR respectively of ΔPQR such that PT=2cm,TR=4cmandST is parallel to QR. Find the ratio of the ares ofΔPSTandΔPQR.
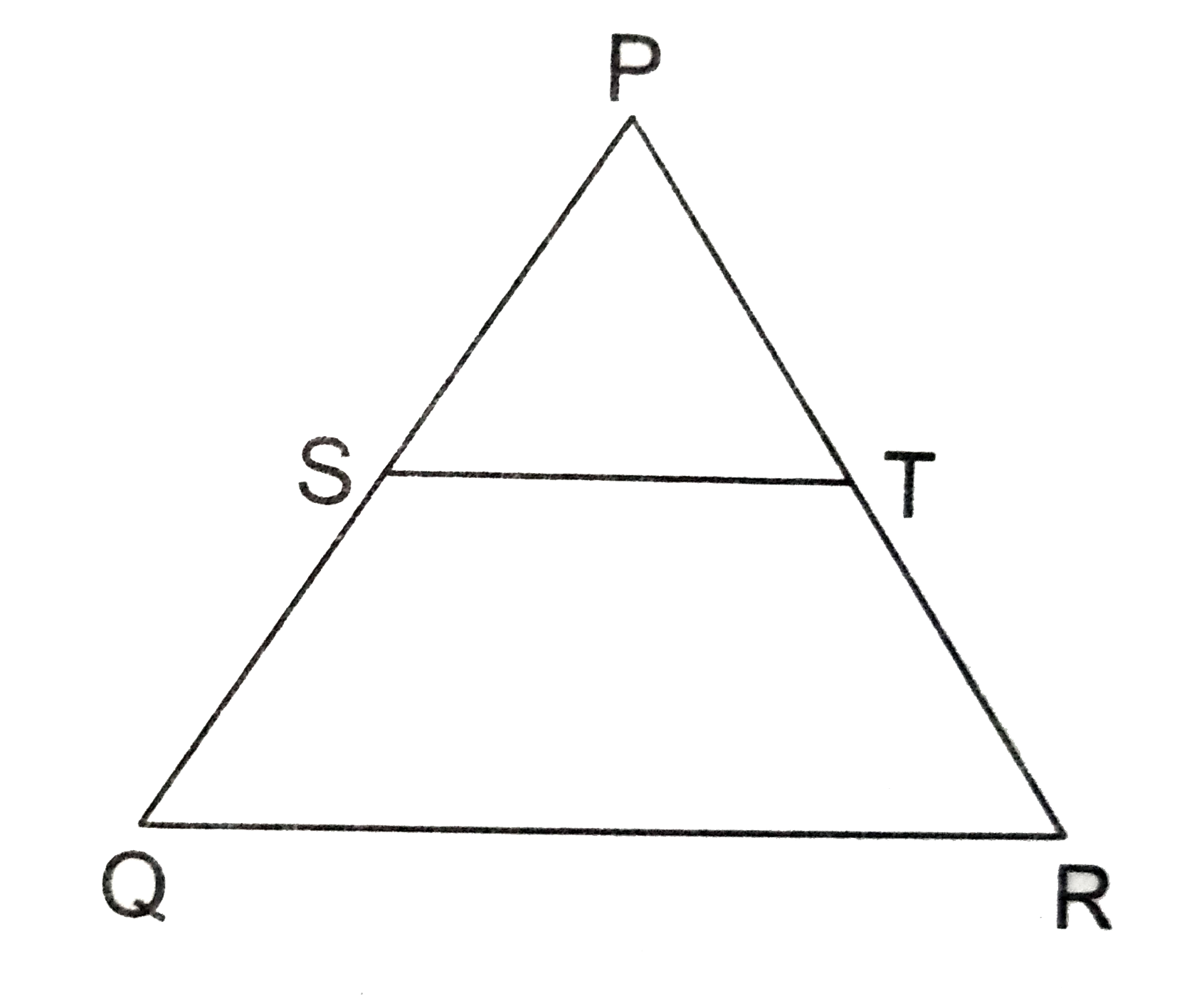
View Solutionचित्र में, बिंदु S तथा T , ΔPQR की भुजाओं PQ तथा PR पर क्रमशः इस प्रकार स्थित है, की PT =2 सेमि., TR = 4 सेमि तथा ST और QR समांतर है| ΔPST तथा ΔPQR के क्षेत्रफलों का अनुपात ज्ञात कीजिए|
(##RDSHINMATX_C04E08019_Q01##)
View SolutionTwo triangles ΔPQR and ΔSQR are drawn on the same base QR and on the same side of QR and their areas are equal. If F and G are two centroids of two triangles, then prove that FG∣∣QR.
View SolutionTwo triangles QPR and QSR, right angled at P and S respectively are drawn on the same base QR and on the same side of QR. If PR and SQ intersect at T, prove that PT×TR=ST×TQ.
View SolutionDiagonal PR and QS of quadrilateral PQRS intersects at T such that PT= TR and PS= QR, show that ar (ΔPTS)= ar (ΔRTQ)
View SolutionQR ஐ அடிப்பக்கமாகக் கொண்ட இரு முக்கோணங்கள் QPR மற்றும் QSR -ன் புள்ளிகள் P மற்றும் S -ல் செங்கோணங்களாக அமைந்துள்ளது .இரு முக்கோணங்களும் QR -யின் ஒரே பக்கத்தில் அமைந்துள்ளது .PR மற்றும் SQ என்ற பக்கங்கள் T என்ற புள்ளியில் சந்திக்கின்றன எனில் ,PTXTR=STXTQ என நிறுவுக ?
View SolutionS and T are points on the sides PQ and PR, respectively of triangle PQR, such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ∆PST and ∆PQR
View SolutionS and T are points on the sides PQ and PR, respectively of ΔPQR, such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ΔPST and ΔPQR.
View Solution
