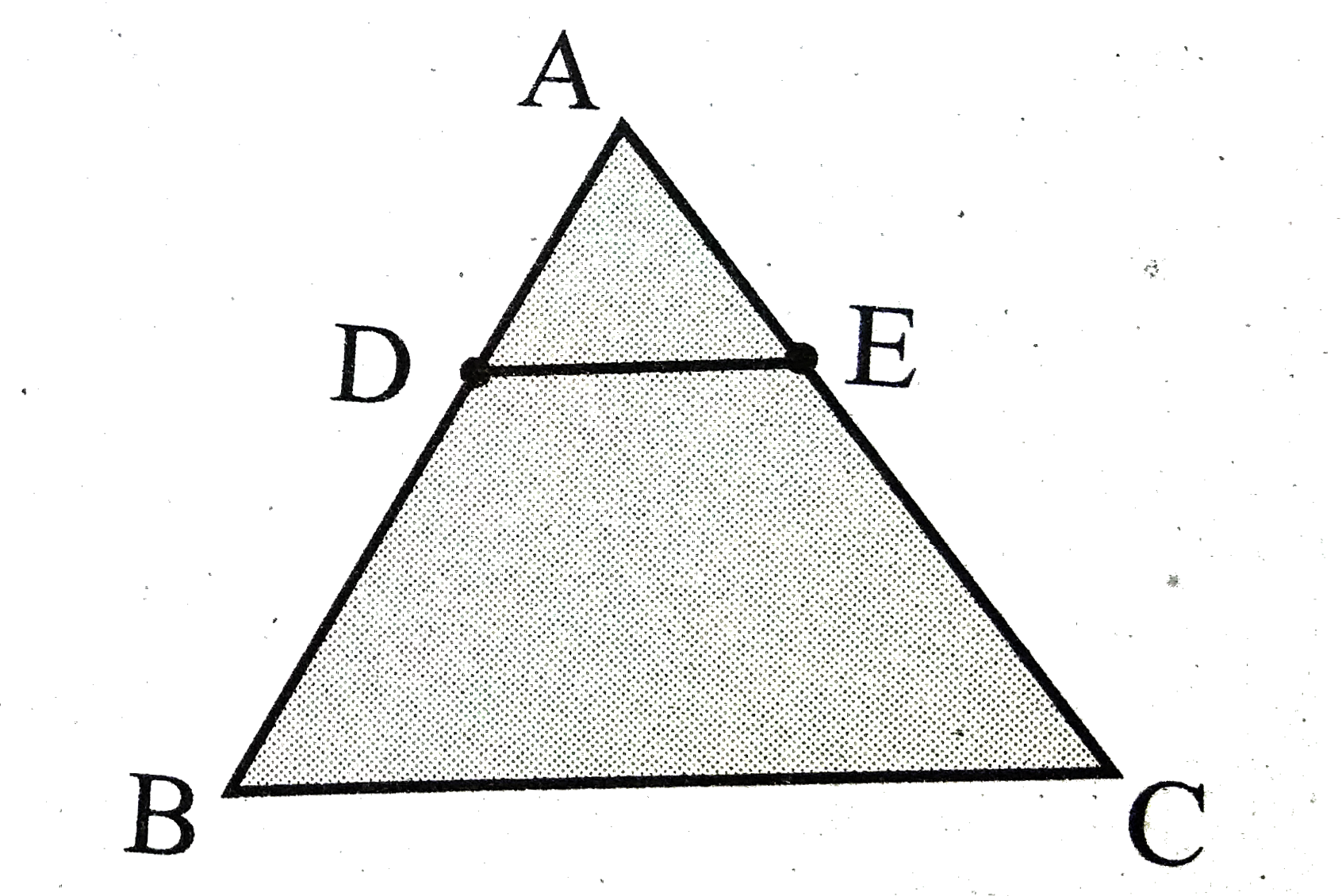
In the figure, ABC is a triangle in which AB= AC. Points D and E are points on the side AB and AC respectively such that AD=AE. Show that points B, C, E and D lie on a same circle.
 Solution in Tamil
Solution in Tamil
Step by step video & image solution for In the figure, ABC is a triangle in which AB= AC. Points D and E are points on the side AB and AC respectively such that AD=AE. Show that points B, C, E and D lie on a same circle. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - ADDITIONAL QUESTIONS SOLVED (MULTIPLE CHOICE QUESTIONS)23videos
GEOMETRY
- BOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - ADDITIONAL QUESTIONS SOLVED (ANSWER THE FOLLOWING QUESTIONS)24videos
GEOMETRY
- BOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - EXERCISE 4.5 (MULTIPLE CHOICE QUESTIONS)15videos
GEOMETRY
- BOOK - FULL MARKSCHAPTER - DEPARTMENTAL MODEL PAPER EXERCISE - PART-IV3videos
DEPARTMENTAL MODEL PAPER
- BOOK - FULL MARKSCHAPTER - MENSURATION EXERCISE - ADDITIONAL QUESTIONS SOLVED (iii)8videos
MENSURATION
Similar Questions
If a straight line intersects the sides AB and AC of a △ABC at D and E respectively and is parallel to BC , then AEAC= . ………..
04:07View SolutionThe image of man height 1.8m , is of length 1.5 cm on the film of a camera . If the film is 3 cm from the camera, how far is the man from the camera ?
03:50View SolutionIf in triangles ABC and EDF, ABDE=BCFD then they will be similar, when
03:42View SolutionPQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T . Find the length TP .
02:38View SolutionA vessel is in the form of a hemispherical bowl mounted by a hollow cylinder. The diameter is 14 cm and the height of the vessel is 13 cm. Find the capacity of the vessel.
03:26View SolutionABC is an isosceles triangle in which AB=AC. If DandE are the mid-points of ABandAC respectively, prove that the points B,C,DandE are concylic.
02:11View SolutionIn Figure, ABC is a triangle in which AB=AC. Point DandE are points onthe sides ABandAC respectively such that AD=AE. Show that the points B,C,EandD are concyclic.
02:46View SolutionABC is a triangle in which AB=AC.DandE are points on the sides ABandAC respectively, such that AD=AE. Show that the points B<C<EandD are concyclie.
05:41View SolutionABC is an isosceles triangle in which AB=AC. If D and E are the mid-points of AB and AC respectively, prove that the points B, C, D and E are concyclic.
01:13View SolutionIn the given figure (not to scale), O is the centre of the circle. A,B,C and D are concyclic and AB=CD . If /_MON =120^(@) , then find /_ OPN .
05:01View SolutionDetermine the point of symmetry of a regular hexagon.
05:01View SolutionIn the figure above (not to scale), ¯¯¯¯¯¯AB⊥¯¯¯¯¯¯CDandAD is the bisector of ∠BAE.AB=3cm and AC=5cm. Find CD.
07:41View SolutionMatch the following Column A to Column B
 03:20View Solution
03:20View SolutionIn the figure (not to scale) AB = AC and BD = CD. Find ∠ADB
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C15_E05_003_Q01.png" width="80%"gt03:09View SolutionIn the figure, ABC is a triangle in which AB= AC. Points D and E are points on the side AB and AC respectively such that AD=AE. Show that points B, C, E and D lie on a same circle.
 02:15View Solution
02:15View SolutionIn the figure, ABC is a triangle in which AB= AC. Points D and E are points on the side AB and AC respectively such that AD=AE. Show that points B, C, E and D lie on a same circle.
 05:25View Solution
05:25View Solution

