Doubtnut Promotions Sticky

Let R be a relation defined on S, the set of squares on a chess board such that xRy iff x and y share a common side. Then, which of the following is false for R?

A

Reflexive only

B

Symmetric

C

Transitive

D

All the above.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

(i) Check by taking examples.
(ii) Consider the three squares of the board as follows.
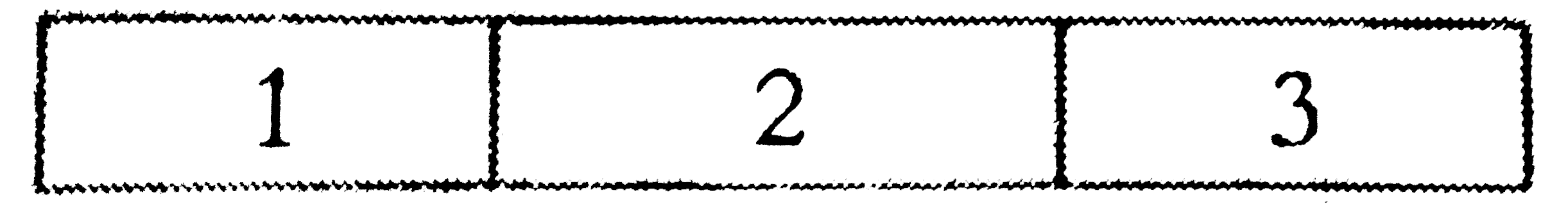
ocr_image
Squares 1 and 2 share a common side and squares 2 and 3 share a common side, but squares 1 and 3 does not share 4 common side.

|
Updated on:21/07/2023
Class 9MATHSSETS AND RELATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Let R be the relation defined on the set N of natural numbers by the rule xRy iff x + 2y = 8, then domain of R is

    A{2, 4, 8}
    B{2, 4, 6}
    C{2, 4, 6, 8}
    D{1, 2, 3, 4}
  • Question 2 - Select One

    Let A be a set of compartments in a train. Then the relation R defined on A as aRb iff ''a and b have the link between them'', then which of the following is true for R?

    Areflexive
    Bsymmetric
    Ctransitive
    Dequivalence
  • Question 3 - Select One

    Let R be the relation on the set R of all real numbers, defined by a→b if |a−b|≤1. Then, R is

    AReflexive and symmetric only
    BReflexive and transitive only
    CEquivalence
    DNone of the above