A magnet is dropped vertically downwards along the axis of the Aluminium loop of wire lying horizontally. Will there be any difference in the time of fall if the wire is at room temperature and heated to below its melting point ? If so, explain. (Hint: Resistance of a conductor is directly proportional to its temperature).
(i) Change in the magnetic field
(ii) Dependence of induced current on resistance of the coil
(iii) Dependence of resistance on temperature
(iv) Force between induced current and magnet.
(v) Dependence of fall in amount of current induced.
Topper's Solved these Questions
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ELECTROMAGNETISMEXERCISE - TEST YOUR CONCEPS ( VERY SHORT ANSWER TYPE QUESTIONS)32videos
ELECTROMAGNETISM
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ELECTROMAGNETISMEXERCISE - TEST YOUR CONCEPS ( SHORT ANSWER TYPE QUESTIONS)15videos
ELECTROMAGNETISM
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ELECTROMAGNETISMEXERCISE - Concept Application (Level 2)14videos
ELECTROMAGNETISM
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ELECTRICITYEXERCISE - CONCEPT APPLICATION (Level 3)10videos
ELECTRICITY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - FORCE AND PRESSUREEXERCISE - COMPETITION CORNER37videos
FORCE AND PRESSURE
Similar Questions
Answer questio numbers (a)-(d) on the basis of your understanding of the following paragraph and the related studied concepts:
We know that current flowing through a conductor depends upon its resistance and potential difference across its ends. The resistance of the conductor depends of (i) its length (ii) its area of cross section and (iii)on the nature of its material. PRecise measurements show that resistance of a uniform metallic conductor is directly proportional to its length (l) and inversely proportional to the area of cross section (A). thus, we can write
R=ρlA where p is a constant whose value depends on the material of the conductor.
WHo studied the relation between current flowing through a conductor and potential difference across its ends?
(b) State the relationship between current, resistance and potential difference in a mathematical form.
(c) Name the constant p whose value depends on the material of the conductor and give its SI unit.
(d) How does value of p governs your choice of material to be used as live wire for electrical fittings in your house? Name two suitable materials.View SolutionDefine resistance of a conductor and its cause. Explain the factors on which its value depends. How will the resistance of a wire of metal be affected if its temperature is changed?
View Solution
Knowledge Check
- Question 1 - Select One
Assertion (A): When a resistance wire is stretched so as to make its length double, its resistance becomes 4 times of its original value.
Reason (R ): Resistance of a conductor is directly proportional to its length and inversely proportional to its cross section area.ABoth (A) and (R ) are true and (R ) is correct explanation of the assertionBBoth (A ) and ( R) are true but (R ) is not the correct explanation of the assertion.C(A ) is true but (R ) is falseD(A) is false but (R ) is true - Question 2 - Select One
Assertion: When we increase the temperature of a metal wire then its electrical resistance increases.
Reason: When we increase the temperature of a conductor then its relaxation time also increases.AIf both assertion and reason are correct and reason is a correct explanation of the assertionBIf both assertion and reason are correct but reason is not the correct explanation of the assertionCIf assertion is correct but reason is incorrectDIf assertion is incorrect but reason is correct - Question 3 - Select One
A copper ring having a cut such as not to from a complete loop is held horizontally and a bar magnet is dropped through the ring with its length along the axis of the ring. The acceleration of the falling magnet is
AgB<gC>gDnone
Newton’s law of cooling says that the rate of cooling of a body is proportional to the temperature difference between the body and its surrounding when the difference in temperature is small.
(a) Will it be reasonable to assume that the rate of heating of a body is proportional to temperature difference between the surrounding and the body (for small difference in temperature) when the body is placed in a surrounding having higher temperature than the body?
(b) Assuming that our assumption made in (a) is correct estimate the time required for a cup of cold coffee to gain temperature from 10∘C to 15∘C when it is kept in a room having temperature 25∘C. It was observed that the temperature of the cup increases from 5∘C to 10∘C in 4 minView SolutionIf all the energy lost from joule heating stays in a wire , and the temperature increases as a result the resistivity also increase. The current will therefore change as a function of time of time, the joule heating will change and so forth. If the wire material has constant heat capacity , the rate of energy loss in wire will be proportional to rate of change of temperature . Assuming that potential stays constant set up a differential equation that describes the rate of change of temperature .If this equation is solved, how can the current he found as a function of time ?
(Given m is the mass of the wire , V is the potential difference across the wire, C is the specfic heat capacity of wire , α is the thermal coefficient of resistance, R0 is the resistance of wire at initial temperature T0).View SolutionAssertion : A wire of uniform cross-section and uniform resistivity is connected across an ideal cell. Now the length or wire is doubled keeping volume of wire constant. The drift velocity of electrons after stretching the wire becomes one fourth of what it was before streching the wire.
Reason: If a wire (or uniform resistivity and uniform cross-section) of length l0 is stretched by a factor n, then its resistance becomes n2 times the one before stretching the wire (the volume of wire is kept constant in stretching process.) Fruther at constant potential difference, current is inversely proportional to resistance. Drift velocity of free electron is directly proportional to current and inversely proportional to cross-sectional area of current carrying wire.View SolutionA special metal S conducts electricity without any resistance. A closed wire loop made of S does not allow any change in flux through itself by inducting a suitable current to generate a compensating flux. This current gives rise to a magnetic moment which in turn repels the source of magnetic field or flux. Consider such a loop, of radius a, with its center at the origin. A magnetic dipole of moment m is brought along the axis of this loop from infinity to a point at distance r(>> a) from the center of the loop with its north pole always facing the loop, as shown in the figure below. The magnitude of magnetic field of a dipole m at a point on its axis at distance r is μ0m2πr3. The magnitude of the force between two magnetic dipoles with moments m1 and m2 separated by a distance r on common axis with their north pole facing each other is km1m2r4 where k is a constant of appropriate dimensions. The direction of this force is along line joining two dipoles.
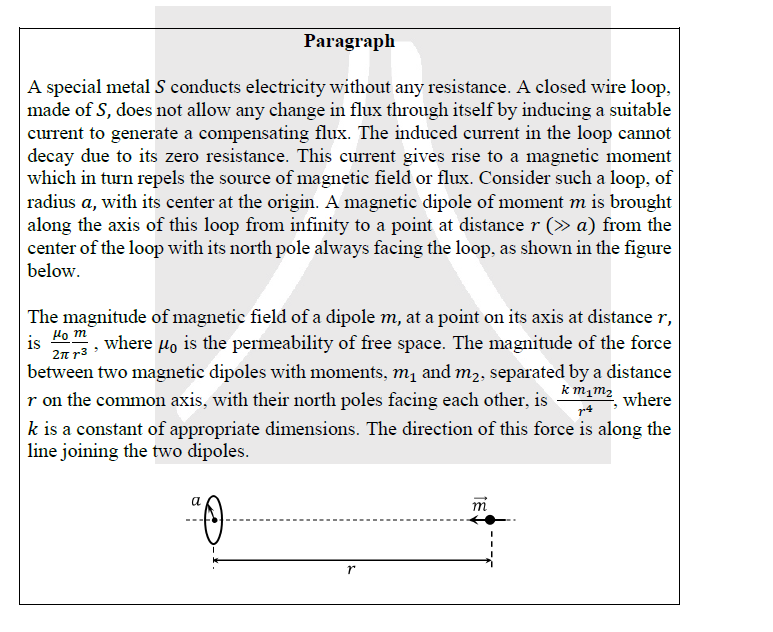When the dipole m is placed at a distance r from center of the loop the current induced in the loop will be proportional to View SolutionStatement I: A wire of uniform cross-section and uniform resistivity is connected across an ideal cell. Now the length of the wire is doubled keeping volume of the wire constant. The drift velocity of electrons after stretching the wire becomes one-fouth of what it was before stretching the wire.
Statement II: If a wire (of uniform resistivity and uniform cross section) of length l0 is stretched to length nl0, then its resistance becomes n2 times of what it was before stretching the wire (the volume of wire is kept constant in stretching process). Further at constant potential difference, current is inversely proportional to resistance. Finally, drift velocity of free electron is directly proportional to current and inversely proportional to cross-sectional area of current carrying wire.View Solution