In a radioactive disintegration, the ratio of initial number oif atoms to the number of atoms present at an instant of tme equal to its mean life is Solution in Kannada
1/e2
1/e
e
e2
The correct Answer is:C
Let the initial number of atoms at time t=0 be N0.
Let the number of atoms at any instant of time t be N.
According to radioactive decay law N=N0e−λt
Mean life τ=1/λ, where λ is the decay constant.
here t=τ (given)
∴N=N0e−1τ×τ or N=N0e−1 or N0N=e
Topper's Solved these Questions
Similar Questions
If μ0 is permeability of free space and ε0 is permittivity of free space , the speed of light in vacuum is given by
View SolutionAll capacitors used in the diagram are identical and each is capacitance C .Then the effective capacitance between the points A and B is
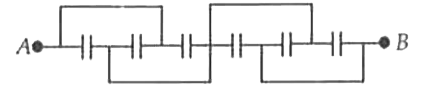
View SolutionThe rate of disintegration of a radioactive substance is …………………………… to the number of atoms present at that time .
View Solution1g of a radioactive substance disintegrates at the rate of 3.7xx10^(10) disintegrations per second. The atomic massof the substance is 226. Calculate its mean life.
View SolutionIn a radioactive disintegration, the ratio of initial number of atoms to the number of atoms present at an instant of time equal to its mean life is
View SolutionThe rate of disintegration at a given instant, is directly proportional to the number of atoms present at that instant. This is the statement of
View SolutionThe radioactive sources A and B of half lives of 2hr and 4hr respectively, initially contain the same number of radioactive atoms. At the end of 2 hours, their rates of disintegration are in the ratio:-
View Solutionकिसी रेडियोएक्टिव पदार्थ की अर्ध्द - आयु में उसमें उपस्थित परमाणुओं की संख्या कितनी रह जायेगी ?
View SolutionThe half-life period of a radioactive element A is same as the mean life time of another radioactive element B. Initially both have the same number of atoms. Then
View SolutionThe half-life of a radioactive sample A is same as the mean life of sample B. The number of atoms present initially in both the samples is same.
View SolutionThe half-line period of a radioactive element A is same as the mean life time of another radioactive element B. Initially both have the same number of atoms. Then
View SolutionFind the probability that a certain radioactive atom would get disintegrated in a time equal to the mean life of the radioactive sample.
View SolutionFind the probability that a certain radioactive atom would get disintegrated in a equal to the mean life of the radioactive sample.
View Solution1g of a radioactive substance disintegrates at the rate of 3.7×1010 disintegrations per second. The atomic massof the substance is 226. Calculate its mean life.
View SolutionThe rate of disintegration of a radioactive substance is …………………………… to the number of atoms present at that time .
View Solution
