In a parallelogram ABCD, AB = 10 cm and AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.
∠ADE is a isosceles triangle
AD=DE=6cm
EC=DC-DE
=10-6=4cm
∠EFC~∠AFB
ABEC=BFCF
104=6CF+1
CF=4cm.
Similar Questions
In a parallelogram ABCD, AB = 10 cm and AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.
View SolutionIn a parallelogram ABCD, AB = 10 cm and AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.
View Solution
Knowledge Check
In a parallelogram ABCD, the bisector of anl≥A also bisects BC at E, find the AD.
AABB2ABC3ABD12ABA parallelogram ABCD has sides AB = 24 cm and AD = 16 cm. The distance between the sides AB and DC is 10 cm. Find the distance between the sides AD and BC.
A16 cmB18 cmC15 cmD26 cm
In a parallelogram ABCD, AB= 20 cm and AD= 12cm. The bisector of angle A meets DC at E and BC produced at F. Find the length of CF.
View SolutionIn parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that :
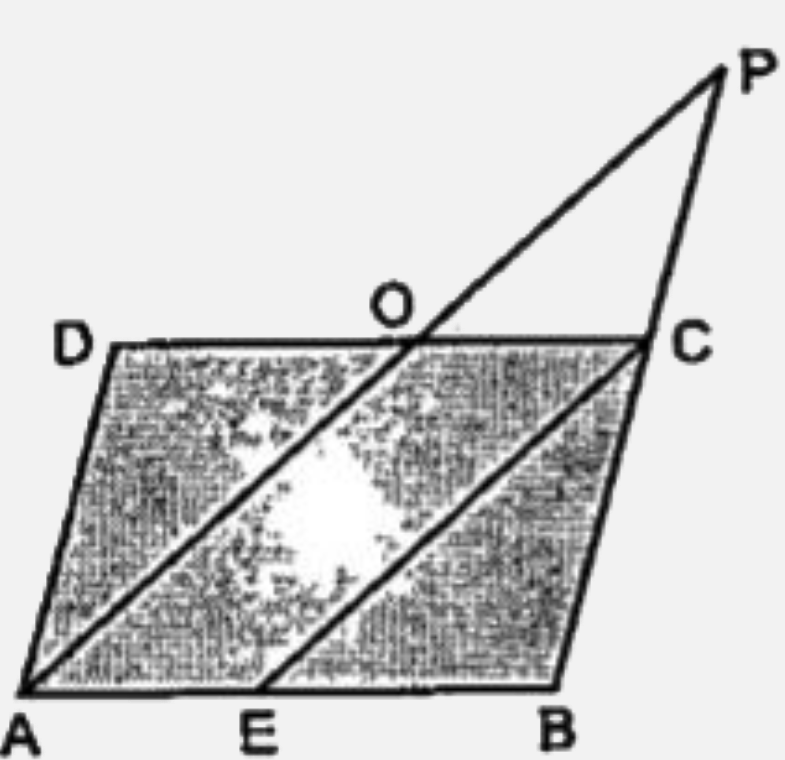
BP=2AD
View SolutionIn parallelogram ABCD, the bisector of angle A meets DC at P and AB= 2AD. Prove that:
BP bisects angle B
View SolutionIn parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that:
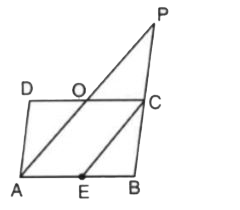
PB=2AD
View SolutionIn parallelogram ABCD, the bisector of angle A meets DC at P and AB= 2AD. Prove that:
Angle APB= 90∘
View SolutionUsing ruler and compasses only, construct a parallelogram ABCD, using the following data: AB=6cm,AD=3cmand∠DAB=45∘. If the bisector of ∠DAB meets DC at P, prove that ∠APB is a right angle.
View Solution
