Prove that :
adj.O=O
To prove that the adjoint of the null matrix O is the null matrix itself, we can follow these steps:
Step 1: Define the Null Matrix
Let O be an n×n null matrix, which means all its elements are zero:
O=⎛⎜
⎜
⎜
⎜
⎜⎝00⋯000⋯0⋮⋮⋱⋮00⋯0⎞⎟
⎟
⎟
⎟
⎟⎠
Step 2: Recall the Definition of Adjoint
The adjoint of a matrix A, denoted as adj(A), is defined as the transpose of the cofactor matrix of A.
Step 3: Calculate the Determinant of the Null Matrix
The determinant of the null matrix O is:
det(O)=0
Step 4: Use the Formula for Inverse
The inverse of a matrix A is given by:
A−1=adj(A)det(A)
For the null matrix O:
O−1=adj(O)det(O)=adj(O)0
Since division by zero is undefined, we cannot find the inverse of the null matrix.
Step 5: Analyze the Adjoint of the Null Matrix
From the properties of matrices, we know that:
O−1 is undefined⟹adj(O) must also be such that it results in a null matrix when multiplied by 0.
Thus, we can express:
adj(O)=O−1⋅det(O)=O−1⋅0=O
Step 6: Conclusion
Thus, we conclude that:
adj(O)=O
This proves that the adjoint of the null matrix is the null matrix itself.
Let O be an n×n null matrix, which means all its elements are zero:
O=⎛⎜
⎜
⎜
⎜
⎜⎝00⋯000⋯0⋮⋮⋱⋮00⋯0⎞⎟
⎟
⎟
⎟
⎟⎠
The adjoint of a matrix A, denoted as adj(A), is defined as the transpose of the cofactor matrix of A.
The determinant of the null matrix O is:
det(O)=0
The inverse of a matrix A is given by:
A−1=adj(A)det(A)
For the null matrix O:
O−1=adj(O)det(O)=adj(O)0
Since division by zero is undefined, we cannot find the inverse of the null matrix.
From the properties of matrices, we know that:
O−1 is undefined⟹adj(O) must also be such that it results in a null matrix when multiplied by 0.
Thus, we can express:
adj(O)=O−1⋅det(O)=O−1⋅0=O
Thus, we conclude that:
adj(O)=O
This proves that the adjoint of the null matrix is the null matrix itself.
Topper's Solved these Questions
- DETERMINANTSBOOK - MODERN PUBLICATIONCHAPTER - DETERMINANTSEXERCISE - Check your understanding
10 Videos
- DETERMINANTSBOOK - MODERN PUBLICATIONCHAPTER - DETERMINANTSEXERCISE - Competition file
14 Videos
- DETERMINANTSBOOK - MODERN PUBLICATIONCHAPTER - DETERMINANTSEXERCISE - Exercise
4 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - MODERN PUBLICATIONCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - CHAPTER TEST
12 Videos
- DIFFERENTIAL EQUATIONSBOOK - MODERN PUBLICATIONCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - CHAPTER TEST (9)
12 Videos
Similar Questions
Prove that :
adj.In=In
View SolutionProve that : (i) adj I=I (ii) adj O=O (iii) I−1=I.
View Solution(i) Prove that (adj adj A) =|A|n–2A
(ii) Find the value of |adj adj adj A| in terms of |A|
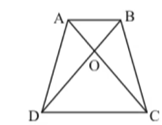
View SolutionIn Figure, ABCD is a trapezium in which AB∣∣CD . Prove that: ar(AOD)=ar(BOC).
View SolutionProve that (adj. A)−1=(adj. A−1).
View Solution(i)Prove that (adj adjA)-|A|n−2A
(ii) Find the value of |adj adj adj A| in terms of |A|
View SolutionIf A is a square matrix of order n, prove that |AadjA|=|A|n
View SolutionABCD IS A PARALLELOGRAM AND O is any point in its interior. Prove that: ar (AOB)+ ar (COD)= ar (BOC)+ ar(AOD) ar (AOB)+ar (COD)=12 ar(^(gm)ABCD) Given: A parallelogram ABCD and O is a point in its interior. To Prove: ar ( AOB)+ ar(COD)=ar (BOC)+ar(AOD)
View SolutionO is any point on the diagonal BD of the parallelogram ABCD. Prove that ar(OAB)=ar(OBC)
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD)=ar ( BOC). Prove that ABCD is a trapezium.
View Solution