Three point masses m1, m2, m3 are placed at the corners of an equilateral triangle of side 2 m. Locate the centre of mass of the system.
m1=2kg,m2=3kg,m3=4kg
To locate the center of mass of the system of three point masses m1,m2,m3 placed at the corners of an equilateral triangle, we will follow these steps:
Step 1: Define the coordinates of the triangle's vertices
We have three masses located at the corners of an equilateral triangle with a side length of 2 m. We can place the triangle in the coordinate system as follows:
- Mass m1=2kg at A(0,0)
- Mass m2=3kg at B(2,0)
- Mass m3=4kg at C(1,√3) (using the height of the triangle, which is √3 when the side is 2 m)
Step 2: Calculate the total mass
The total mass M of the system is given by:
M=m1+m2+m3=2+3+4=9kg
Step 3: Calculate the x-coordinate of the center of mass
The x-coordinate of the center of mass xcm is calculated using the formula:
xcm=m1x1+m2x2+m3x3M
Substituting the values:
xcm=(2⋅0)+(3⋅2)+(4⋅1)9=0+6+49=109≈1.11m
Step 4: Calculate the y-coordinate of the center of mass
The y-coordinate of the center of mass ycm is calculated using the formula:
ycm=m1y1+m2y2+m3y3M
Substituting the values:
ycm=(2⋅0)+(3⋅0)+(4⋅√3)9=0+0+4√39=4√39≈0.77m
Step 5: Final coordinates of the center of mass
Thus, the center of mass of the system is located at:
(xcm,ycm)≈(1.11m,0.77m)
Summary
The center of mass of the system of three point masses located at the corners of an equilateral triangle is approximately at the coordinates (1.11m,0.77m).
---
We have three masses located at the corners of an equilateral triangle with a side length of 2 m. We can place the triangle in the coordinate system as follows:
- Mass m1=2kg at A(0,0)
- Mass m2=3kg at B(2,0)
- Mass m3=4kg at C(1,√3) (using the height of the triangle, which is √3 when the side is 2 m)
The total mass M of the system is given by:
M=m1+m2+m3=2+3+4=9kg
The x-coordinate of the center of mass xcm is calculated using the formula:
xcm=m1x1+m2x2+m3x3M
Substituting the values:
xcm=(2⋅0)+(3⋅2)+(4⋅1)9=0+6+49=109≈1.11m
The y-coordinate of the center of mass ycm is calculated using the formula:
ycm=m1y1+m2y2+m3y3M
Substituting the values:
ycm=(2⋅0)+(3⋅0)+(4⋅√3)9=0+0+4√39=4√39≈0.77m
Thus, the center of mass of the system is located at:
(xcm,ycm)≈(1.11m,0.77m)
The center of mass of the system of three point masses located at the corners of an equilateral triangle is approximately at the coordinates (1.11m,0.77m).
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS (MULTIPLE CHOICE QUESTIONS) (MULTIPLE CHOICE QUESTIONS WITH ONLY ONE CORRECT ANSWER) )
67 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (JEE (Main) & Other State Boards for Engineering Entrance) )
48 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Long Answer Questions Carrying 5 marks)
8 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Knowledge Check
Three point masses m1,m2 and m3 are located at the vertices of an equilateral triangle of side α. What is the moment of inertia of the system about an axis along the altitude of the triangle passing through m1?
A(m1+m2)a24B(m2+m3)a24C(m1+m3)a24D(m1+m2+m3)a24Three point masses, each of mass 2kg are placed at the corners of an equilateral triangle of side 2m. What is moment of inertia of this system about an axis along one side of a triangle?
A2kgm2B4kgm2C6kgm2D8kgm2
Four particles of masses m, m, 2m and 2m are placed at the four corners of square of side a. Find the centre of mass of the system.
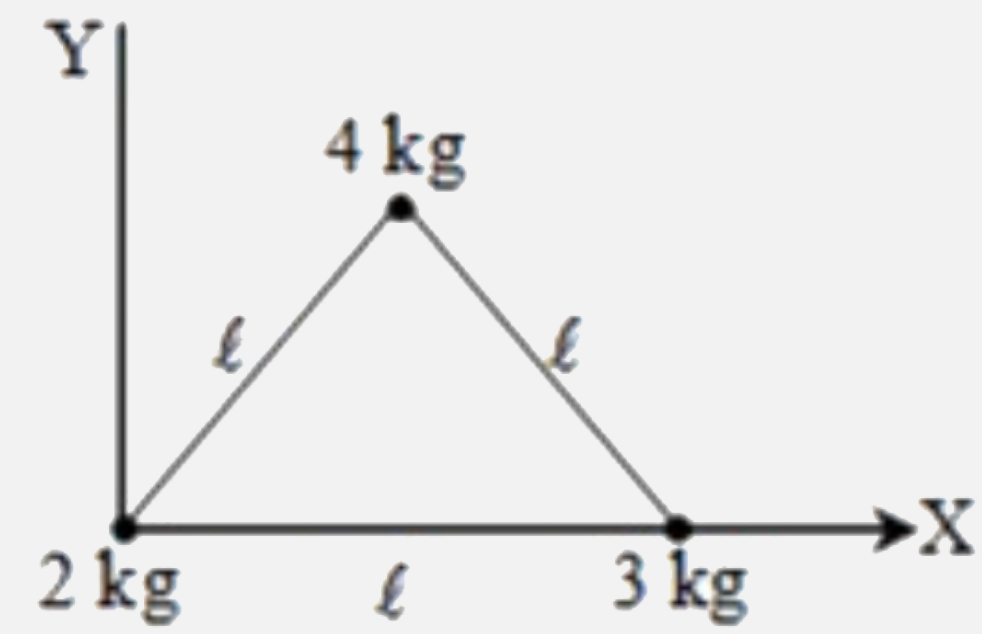
View SolutionThree masses 2 kg , 3kg and 4kg are lying at the corners of an equilateral triangle of side I. The X coordinate of center of mass is
View SolutionFour particles of masses m, m, 2m and 2m are placed at the corners of a square of side a, as shown in fig. Locate the centre of mass.
View SolutionThree point masses each of mass m are placed at the corners of an equilateral triangle of side 'a' . Then the moment of inertia of this system about an axis passing along one side of the triangle is
View SolutionThree particles of msses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre ofmass of the system.
View SolutionThree balls of masses 1kg,2kgand3kg are arranged at the corners of an equilateral triangle of side 1m. What will be the moment of inertia of the system and perpendicular to the plane of the triangle.
View Solution
