From a solid sphere of M and radius R a cube of maximum possible volume is cut. Moment of inertia of cube about an axis passing through its centre and perpendiular to one of its faces is:

A

$\frac{M R^{2}}{16 \sqrt{2} π}$

B

$\frac{4 M R^{2}}{9 \sqrt{2} π}$

C

$\frac{4 M R^{2}}{3 \sqrt{2} π}$

D

$\frac{4 M R^{2}}{32 \sqrt{2} π}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

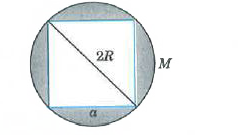
The denisty of the sphere is $ρ = \frac{M}{V} = \frac{M}{\frac{4 π}{3} R^{3}}$
$= \frac{3 M}{4 π R^{3}}$
a = length of the side of the cube of maximum volume $= \frac{2 R}{\sqrt{3}}$
Mass of the cube is $m = ρ a^{3} = (\frac{3 M}{4 π R^{3}}) (\frac{8 R^{2}}{3 \sqrt{3}}) = \frac{2 M}{\sqrt{3} π}$
The moment of inertia of the cube is
$I = \frac{m a^{2}}{6} = \frac{2 M}{\sqrt{3} π} \times \frac{1}{6} \times {(\frac{2 R}{\sqrt{3}})}^{2}$
$= \frac{8 M R^{2}}{18 \sqrt{3} π} = \frac{4 M R^{2}}{9 \sqrt{3} π}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
From a solid sphere of M and radius R a cube of maximum possible volume is cut. Moment of inertia of cube about an axis passing through its centre and perpendiular to one of its faces is:
A $\frac{4 M R^{2}}{3 \sqrt{3} π}$
B $\frac{M R^{2}}{32 \sqrt{2} π}$
C $\frac{M R^{2}}{16 \sqrt{2} π}$
D $\frac{4 M R^{2}}{9 \sqrt{3} π}$
Question 2 - Select One
The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
Aincreases if its temperature is increased
Bchanges if its axis of rotation is changed
Cincreases if its angular velocity is increased
Dboth (a) and (b) are correct
Question 3 - Select One
The moment of inertia of a sphere of radius R about an axis passing through its centre is proportional to-
A $R^{2}$
B $R^{3}$
C $R^{4}$
D $R^{5}$

MODERN PUBLICATION-SYSTEMS OF PARTICLES AND ROTATIONAL MOTION-COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (JEE (Main) & Other State Boards for Engineering Entrance) )

Home
Ask Doubt
Profile