Calculate the electric field intensity at point P due to a charged thin rod AB as shown in the given figure. Charge is distributed uniformly over the length of the rod . The length of rod is l. The distance of point P from end B of the rod is a.Charge per unit length of the rod is λ .Also , find the electric field intensity when the length of rod is infinite .


Refer given figure Uniform positively charged thin rod AB shown in the figure . The length of rod is l and charge per unit length of rod is λ. Electric field intensity at point P is to be calculated. The distance of P from one end of rod is a as shown.
A segment of length dx is selected at a distance x from P. Charge on segment can be written as follows :
dq=λdx
The charge on segment is written by multiplying the charge per unit length (λ) with the length of segment (dx) .
The selected segment can be considered a positive point charge. Electric field due to segment (dE) is directed away from segment along line joining P and segment , as shown in the figure. Using formula of electric field for a point charge we get the following :
dE=14πε0λ(dx)x2
In this case, the direction of electric field intensity , due to all possible segments on rod, remains along the same direction. Hence, dE can be integrated from x=a to x=a+l, for the total electric field intensity due to the rod at point P.
E=∫dE=∫a+la14πε0λ(dx)x2
E=λ4πε0∫a+lax−2dx
E=λ4πε0[−1x]a+la
Now substituting limits we get the following :
E=λ4πε0[(−1a+l)−(−1a)]=λ4πε0[1a−1a+l]
E=λl4πε0a(a+l)
Note that the direction of net electric field intensity is parallel to the rod . If charge is positive then electric field intensity will be directed away from B and in case of negative charge , it will be towards the point B.
The above relation can be easily modified to get electric field intensity when the length of rod is very long. Dividing numerator and denominator by l, we get the following :
E=λl4πε0a(a+l)=λ4πε0a(al+1)
We can reglect a/l in comparison to 1 for large length l of rod. hence , we get the following relation for rod of infinite length :
E=λ4πε0a
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Practice Problems
88 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Conceptual Questions
51 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
14 Videos
Similar Questions
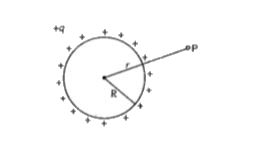
Electric field intensity at point P due to charge distributed over a sphere is:
View SolutionFind the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View Solution
Knowledge Check
Electric field intensity at point P due to charge distributed over a sphere is:
 AE=14πε0qrBE=σR2ε0r2CE=14πε0qrDE=0
AE=14πε0qrBE=σR2ε0r2CE=14πε0qrDE=0
Find the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View SolutionFind the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The lengthof the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View SolutionCalculate the potential due to a thin charged rod of length L at the points along and perpendicular to the length. Charge per unit length on the rod is λ.
View SolutionCalculate the potential due to a thin charged rod of length L at the points along and perpendicular to the length. Charge per unit length on the rod is λ.

View SolutionCalculate electric field intensity at point P due to a thin positive uniformly charged rod . Point P is at perpendicular distance d from the rod . The lower and upper ends of the rod make the angles α and β as shown below .
View SolutionWhat is the electric field intensity at any point on the axis of a charged rod of length 'L' and linear charge density λ? The point is separated from the nearer end by a.
View SolutionWhat is the electric field intensity at any point on the axis of a charged rod of length 'L' and linear charge density λ? The point is separated from the nearer end by a.
View Solution
