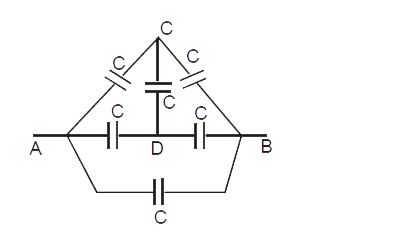
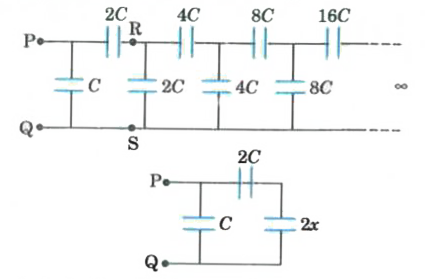
Consider the following network of capacitors extended up to infinity. Find equivalent capacitance between P and Q.
Video Solution
Text Solution
Let x be effective capacitance between P and Q then 2x must be the equivalent capacitance between R and S, as the capacitors, are doubled.
We can write the following equation for the above diagram:
x=C+4Cx2C+2x⇒x=2C2+2Cx+4Cx2C+2x
⇒2Cx+2x2=2C2+2Cx+4Cx
⇒x2−2Cx−C2=0
⇒x=2C±√4C2+4C22=C±C√2
Selecting positive value for the answer we get equivalent capacitance C(√2+1).
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Very Short Answer Questions)56videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Additional Questions)10videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - NCERT FILE Solved (Exemplar Problems) (Short Answer Type Questions)5videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST14videos
ELECTROMAGNETIC WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION16videos
MAGNETISM AND MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
Find equivalent capacitance between A and B
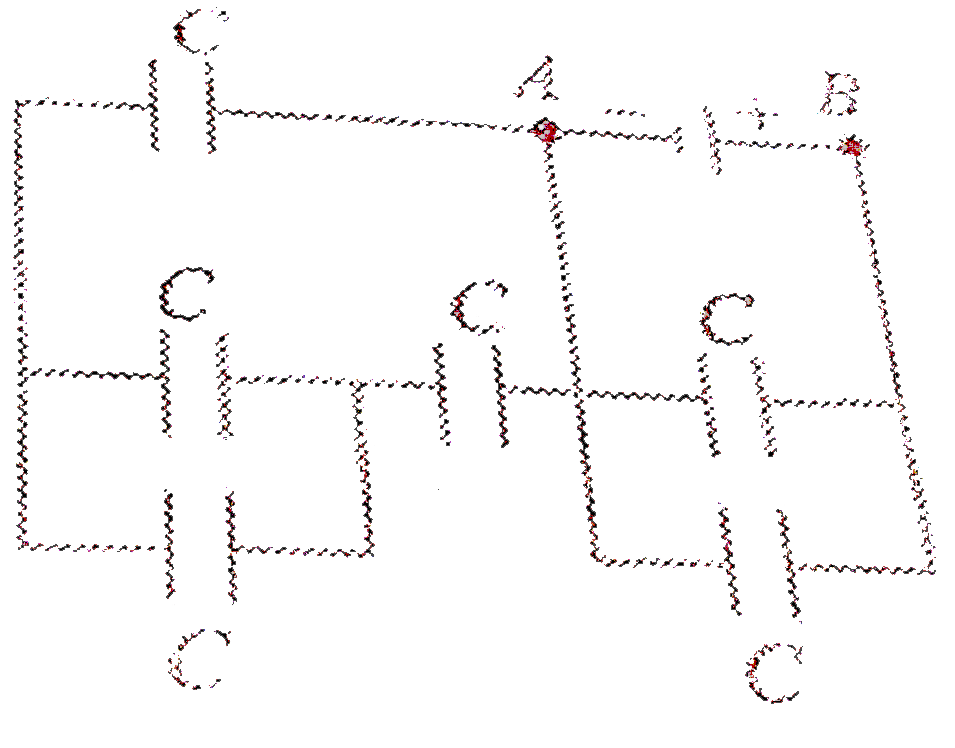 A6CB5CC3CD2C
A6CB5CC3CD2C - Question 2 - Select One
Find equivalent capacitance between A & B
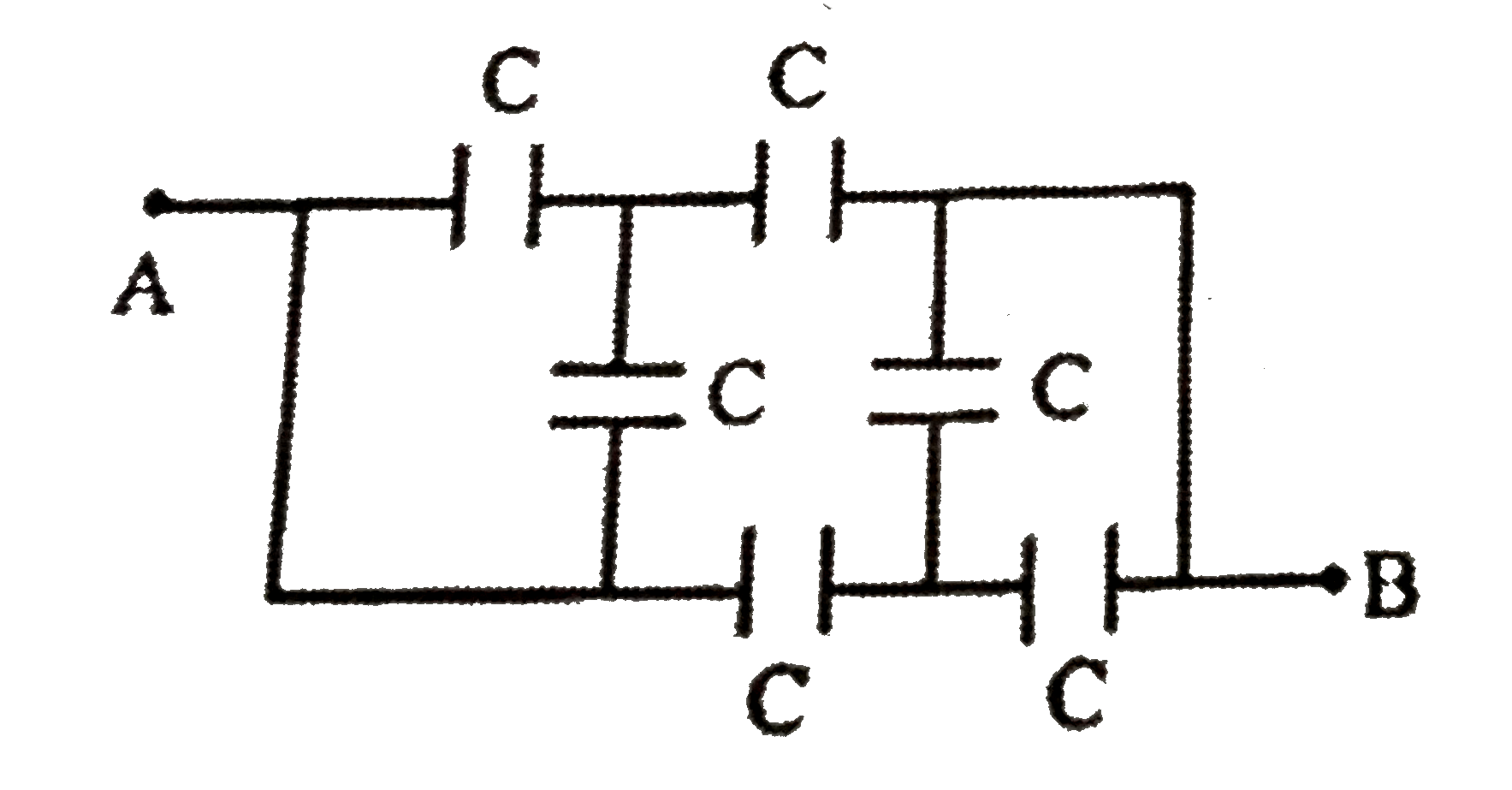 AC4B3G4C3G2D4G3
AC4B3G4C3G2D4G3 - Question 3 - Select One
The equivalent capacitance between P and Q is.
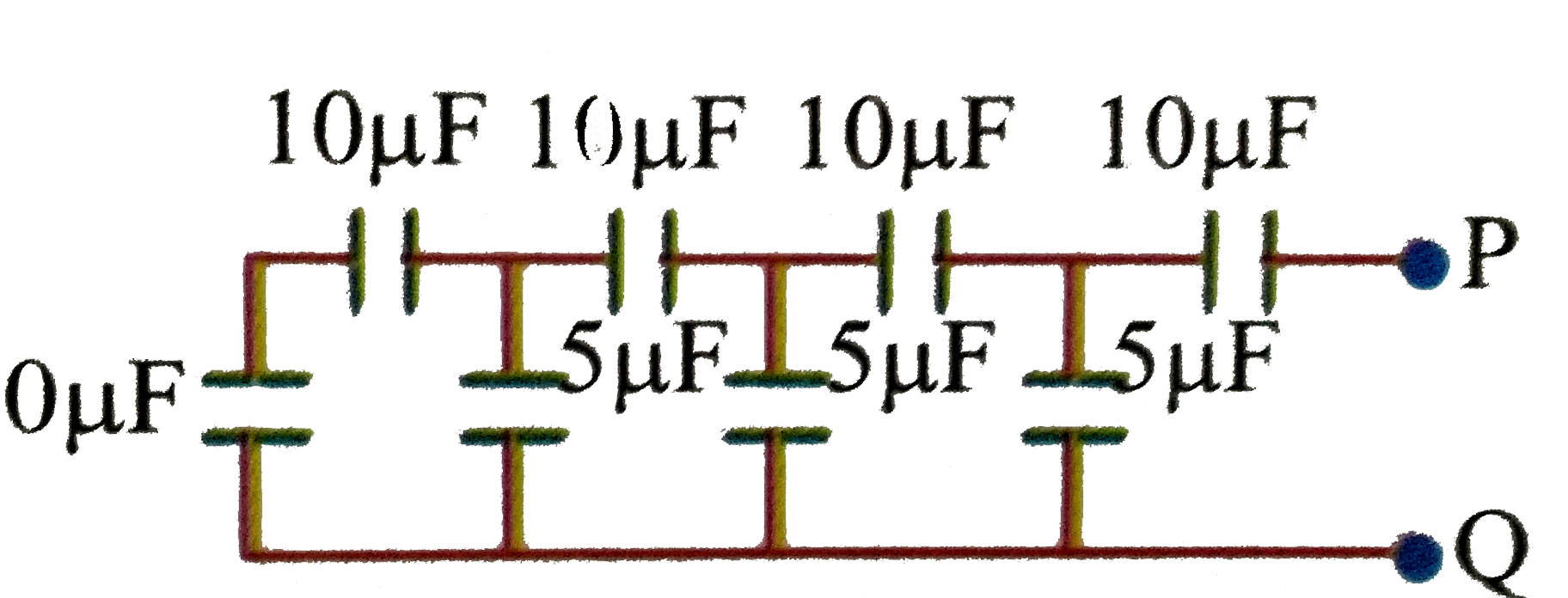 .A10μFB20μFC5μFD15μF
.A10μFB20μFC5μFD15μF
Find equivalent Capacitance between A and B.
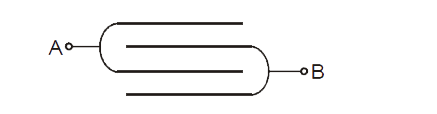 View Solution
View SolutionConsider the following network of capacitors extended up to infinity and find the equivalent capacitance between P and Q.
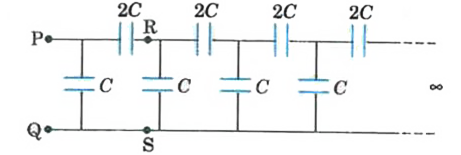 View Solution
View SolutionFind the equivalent capacitance between A and B.
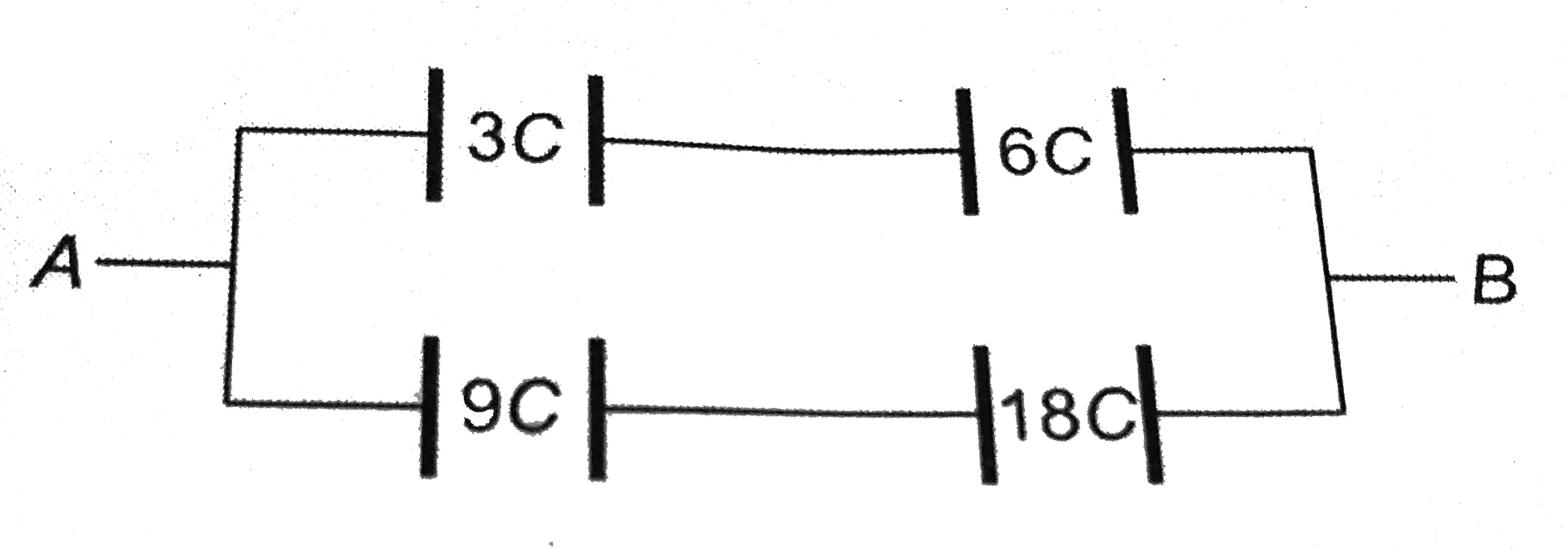 View Solution
View SolutionFind the equivalent capacitance between A and B.
 View Solution
View SolutionFind the equivalent capacitance between A and B
 View Solution
View Solution