What are equipotential surfaces? Give their properties.
Step-by-Step Solution
Step 1: Definition of Equipotential Surfaces
An equipotential surface is defined as the locus of points in an electric field where the electric potential is the same. This means that every point on this surface has the same electric potential value.
Step 2: Understanding with an Example
Consider an electric field represented by lines. If we have a surface in this field where the potential is constant, then that surface is an equipotential surface. For instance, if we have a point charge, the equipotential surfaces around it are spherical shells where every point on a given shell has the same potential.
Step 3: Relation Between Electric Field and Potential
The relationship between electric potential (V) and electric field (E) is given by the equation:
V=−∫E⋅dr
This indicates that if we move perpendicular to the electric field lines, the potential remains constant, leading to the formation of equipotential surfaces.
Step 4: Properties of Equipotential Surfaces
1. Perpendicular to Electric Field Lines: Electric field lines are always perpendicular to equipotential surfaces. This is because the work done in moving a charge along an equipotential surface is zero.
2. Non-Intersecting: Equipotential surfaces cannot intersect each other. If they did, it would imply that a single point has two different potentials, which is not possible.
3. Different Surfaces for Different Fields: Different electric fields create different equipotential surfaces. For example:
- For a point charge, the equipotential surfaces are concentric spheres.
- For a uniform electric field (like that between two parallel plates), the equipotential surfaces are planar.
- For a linear charge distribution (like a charged wire), the equipotential surfaces are cylindrical.
Step 5: Summary of Equipotential Surfaces
In summary, equipotential surfaces are crucial in understanding electric fields and potentials. They help visualize how electric forces act in space and provide insights into the behavior of charged particles in electric fields.
An equipotential surface is defined as the locus of points in an electric field where the electric potential is the same. This means that every point on this surface has the same electric potential value.
Consider an electric field represented by lines. If we have a surface in this field where the potential is constant, then that surface is an equipotential surface. For instance, if we have a point charge, the equipotential surfaces around it are spherical shells where every point on a given shell has the same potential.
The relationship between electric potential (V) and electric field (E) is given by the equation:
V=−∫E⋅dr
This indicates that if we move perpendicular to the electric field lines, the potential remains constant, leading to the formation of equipotential surfaces.
1. Perpendicular to Electric Field Lines: Electric field lines are always perpendicular to equipotential surfaces. This is because the work done in moving a charge along an equipotential surface is zero.
2. Non-Intersecting: Equipotential surfaces cannot intersect each other. If they did, it would imply that a single point has two different potentials, which is not possible.
- For a point charge, the equipotential surfaces are concentric spheres.
- For a uniform electric field (like that between two parallel plates), the equipotential surfaces are planar.
- For a linear charge distribution (like a charged wire), the equipotential surfaces are cylindrical.
In summary, equipotential surfaces are crucial in understanding electric fields and potentials. They help visualize how electric forces act in space and provide insights into the behavior of charged particles in electric fields.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Long Answer Questions)
13 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Numerical Problems)
25 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Fill in the Blanks)
11 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
What is an equipotential surface ? Write three properties Sketch equipotential surfaces of
(i) Isolated point charge
(ii) Uniform electric field
(iii) Dipole
View SolutionWhat is an equipotential surface ? Write three properties Sketch equipotential surfaces of
(i) Isolated point charge
(ii) Uniform electric field
(iii) Dipole If charge Q is given to a parallel plate capacitor and E is the electric field between the plates of the capacitor the force on each plate is 1/2 QE and if charge Q is placed between the plates experiences a force equal to QE. Give reason to explain the above.
View Solution
Knowledge Check
S1 and S2 are two equipotential surfaces on which the potentials are not equal
AS1 and S2 cannot intersect.BS1 and S2 cannot both be plane surfaces.CIn the region between S1 and S2 the field is maximum where they are closest to each other.DA line of force from S1 and S2 must be perpendicular to both.
Can electric field exist longitudinal to an equipotential surface ? Give reason .
View SolutionEquipotential Surfaces and Dipole
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If a charge of 2 nC is taken from equipotential surface number 2 to 3, what is the amount of work done ?
View SolutionCan two equipotential surfaces cut each other?
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Draw equipotential surfaces around an isolated positive point charge + q. Mark direction of electric field →E also.
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Four equipotential surfaces are shown in the adjoining figure. Find the magnitude and direaction of the electric field.
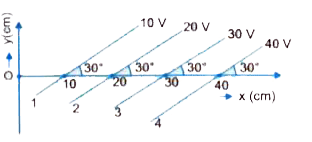
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
A uniform electric field →E=1000ˆiVm−1 exists in space at a given place. What is the shape of equipotential surfaces there ?
View Solution
