How will you orient two circular loops to have (i) smallest mutual inductance (ii) largest mutual inductance?
To determine how to orient two circular loops to achieve the smallest and largest mutual inductance, we can analyze the configurations step by step.
Step 1: Understanding Mutual Inductance
Mutual inductance (M) between two loops depends on how much magnetic flux generated by one loop passes through the other loop. The more flux that passes through the second loop, the larger the mutual inductance.
Step 2: Orientation for Largest Mutual Inductance
To achieve the largest mutual inductance:
- Configuration: Place the two circular loops coaxially (i.e., one loop directly above the other, sharing the same axis).
- Explanation: In this configuration, the magnetic field produced by one loop will completely pass through the other loop. This maximizes the magnetic flux linkage between the two loops, resulting in the largest possible mutual inductance.
Step 3: Orientation for Smallest Mutual Inductance
To achieve the smallest mutual inductance:
- Configuration: Place the two circular loops perpendicularly to each other (i.e., one loop lying flat while the other stands vertically).
- Explanation: In this orientation, the magnetic field lines from one loop do not pass through the other loop at all. As a result, the magnetic flux linkage is effectively zero, leading to the smallest possible mutual inductance.
Summary of Configurations
- Largest Mutual Inductance: Coaxial orientation (loops aligned on the same axis).
- Smallest Mutual Inductance: Perpendicular orientation (loops at 90 degrees to each other).
---
Mutual inductance (M) between two loops depends on how much magnetic flux generated by one loop passes through the other loop. The more flux that passes through the second loop, the larger the mutual inductance.
To achieve the largest mutual inductance:
- Configuration: Place the two circular loops coaxially (i.e., one loop directly above the other, sharing the same axis).
- Explanation: In this configuration, the magnetic field produced by one loop will completely pass through the other loop. This maximizes the magnetic flux linkage between the two loops, resulting in the largest possible mutual inductance.
To achieve the smallest mutual inductance:
- Configuration: Place the two circular loops perpendicularly to each other (i.e., one loop lying flat while the other stands vertically).
- Explanation: In this orientation, the magnetic field lines from one loop do not pass through the other loop at all. As a result, the magnetic flux linkage is effectively zero, leading to the smallest possible mutual inductance.
- Largest Mutual Inductance: Coaxial orientation (loops aligned on the same axis).
- Smallest Mutual Inductance: Perpendicular orientation (loops at 90 degrees to each other).
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Additional Questions (Carrying I mark))
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Fill in the blanks)
7 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - HIGHER ORDER THINKING SKILLS AND ADVANCED LEVEL
8 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
Knowledge Check
The unit of mutual inductance is-
AvoltBweberCteslaDhenryThe coefficient of mutual inductance
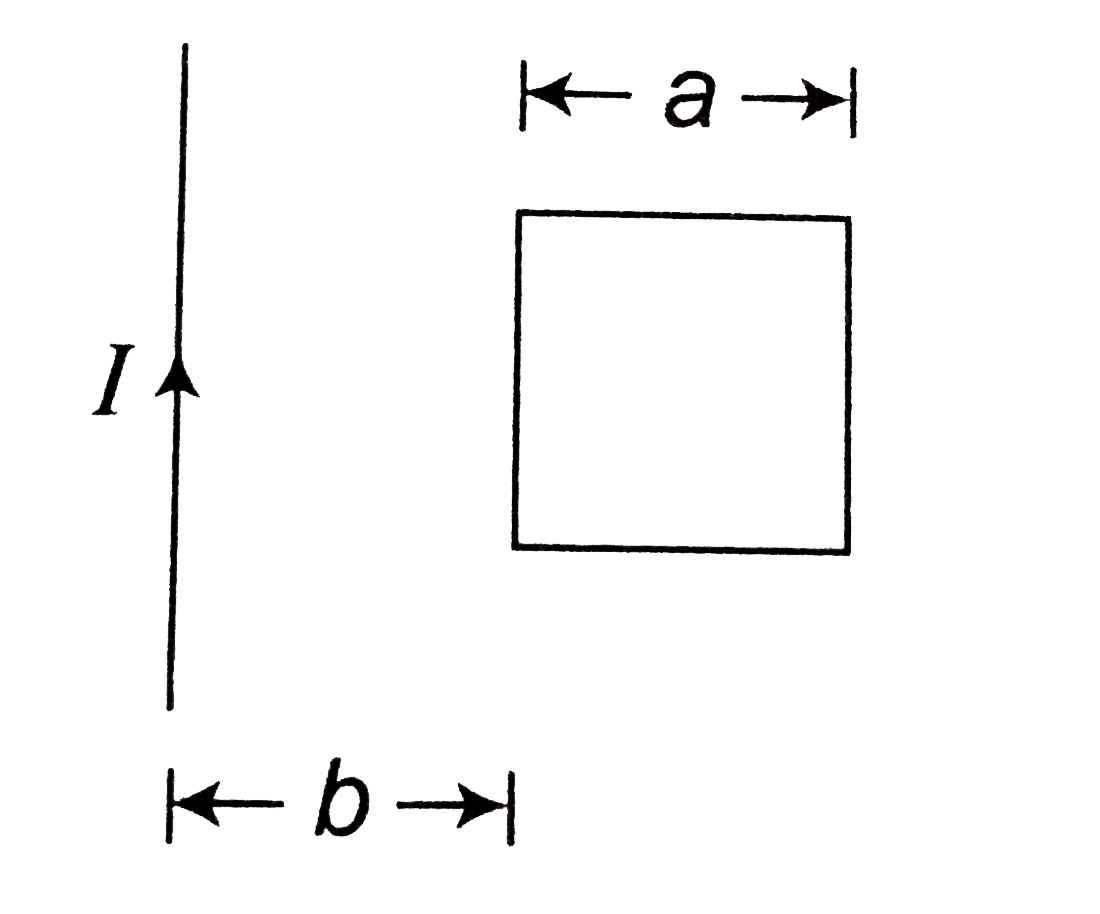 Aμ0a2π1n(1+a2b)Bμ0aπ1n(1+b2a)Cμ0a2π1n(1+ab)Dμ0a2π1n(1+ba)
Aμ0a2π1n(1+a2b)Bμ0aπ1n(1+b2a)Cμ0a2π1n(1+ab)Dμ0a2π1n(1+ba)Mutual inductance depends on:
Anumber of turns of coil 1 onlyBnumber of turns of coil 2 onlyCboth (a) and (b)Dneither (a) nor (b)