Consider the following circuit. Switch Sw is closed at t = 0. Let i1 be the 2R current drawn from battery immediately after the switch is closed and i2 be the current long time after the switch is closed. Calculate i2i2

A
3
B
2
C
1
D
0
Text Solution
The correct Answer is:B
At time i=0 inductor behaves as infinite resistance but capacitor behaves like a zero resistance. Hence, at t = 0 effective resistance of three branches in parallel becomes zero because capacitor is like a zero resistance. Hence, R is the effective resistance of network at t = 0. Hence, i1 can be written as follows: I=E/R.
Long time after the switch is closed, inductor behaves like a zero resistance but capacitor behaves like infinite resistance. Hence, resistances 2R and 2R come in parallel to give effective resistance R which is connected in series with another R. Hence, total resistance of the network long time after the switch is closed becomes 2R. Hence, current flowing can be written as follows:
I2=E/2R.
Ratio of currents: i1/i2=2.
Hence, option (b) is correct.
|
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (MULTIPLE CHOICE QUESTIONS) (AIPMT/NEET & Other State Boards for Medical Entrance)
8 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (MULTIPLE CHOICE QUESTIONS) (JEE (Main) & Other State Boards for Engineering Entrance)
38 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Numerical Problems)
16 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
Knowledge Check
In the circuit diagram the current through the battery immediately after the switch S is closed is
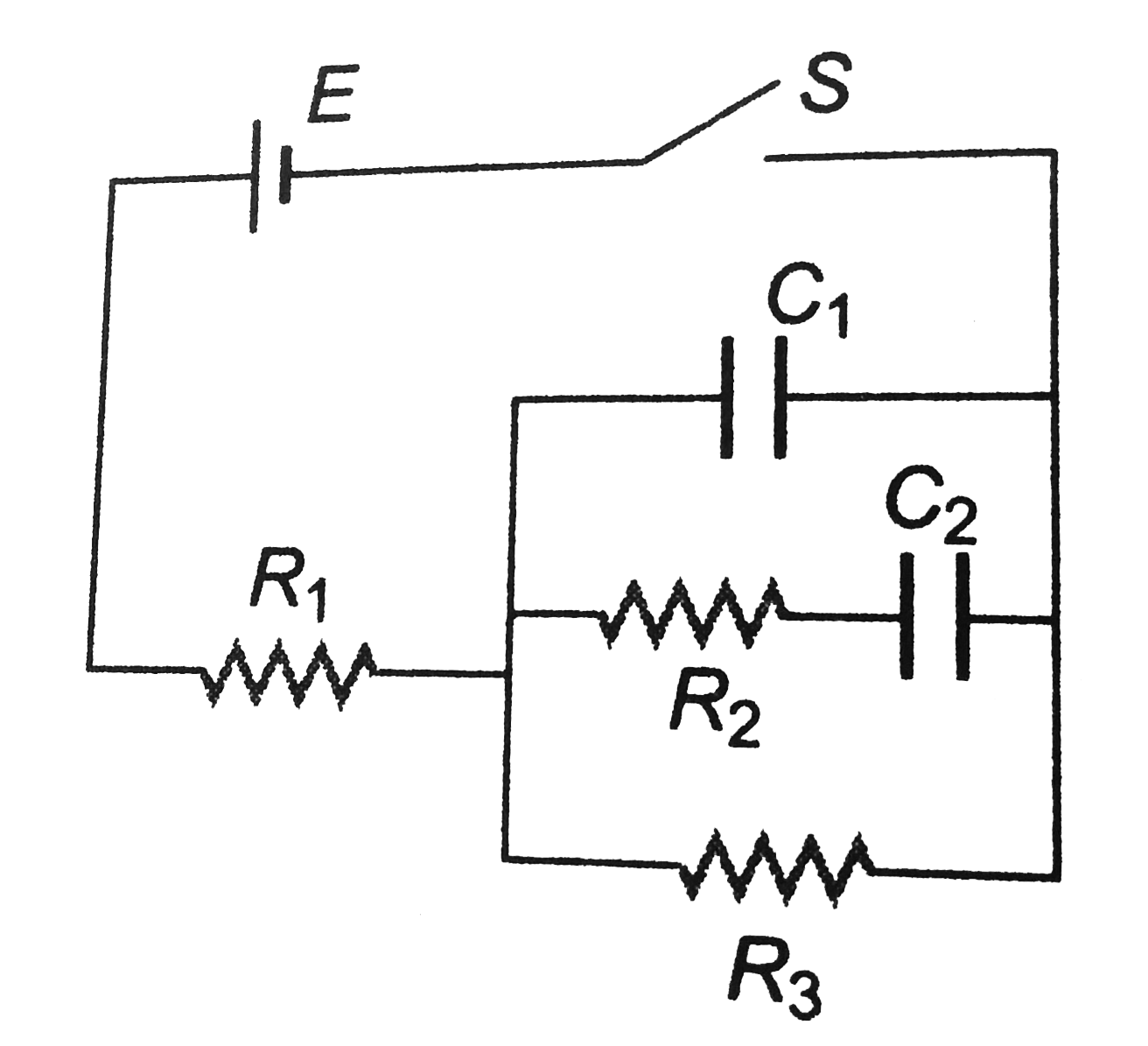 AzeroBER1CER1+R2DER1+R2R3R2+R3
AzeroBER1CER1+R2DER1+R2R3R2+R3In the circuit as shown in figure the switch is closed at t=0.

A long time after closing the switchAvoltage drop across the capacitor is EBcurent through the battery is E/R1+R2Cenegru stores in the capacitor is
12C(R2ER1+R2)2Dcurrent through the capacitor becomes zero
Current drawn by the battery just after switch 'S' is closed is :-
View SolutionFind the values of i1 and i2
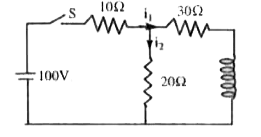
i) immediately after the switch S is closed.
View SolutionFind the current through the battery
(i) just after the switch is closed.
(ii) long after the switch has been closed.
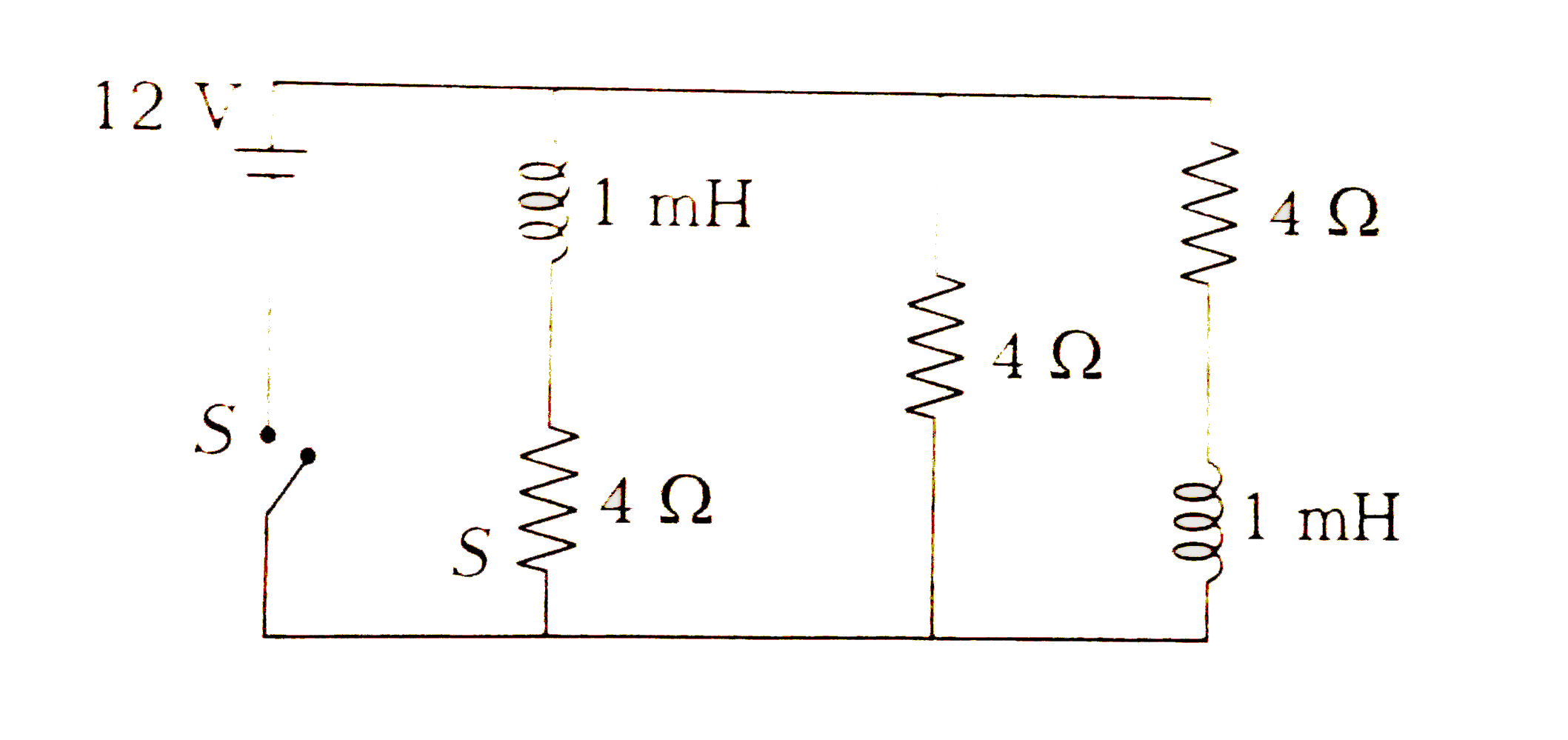
View SolutionFind the current through the battery
(i) just after the switch is closed.
(ii) long after the switch has been closed.
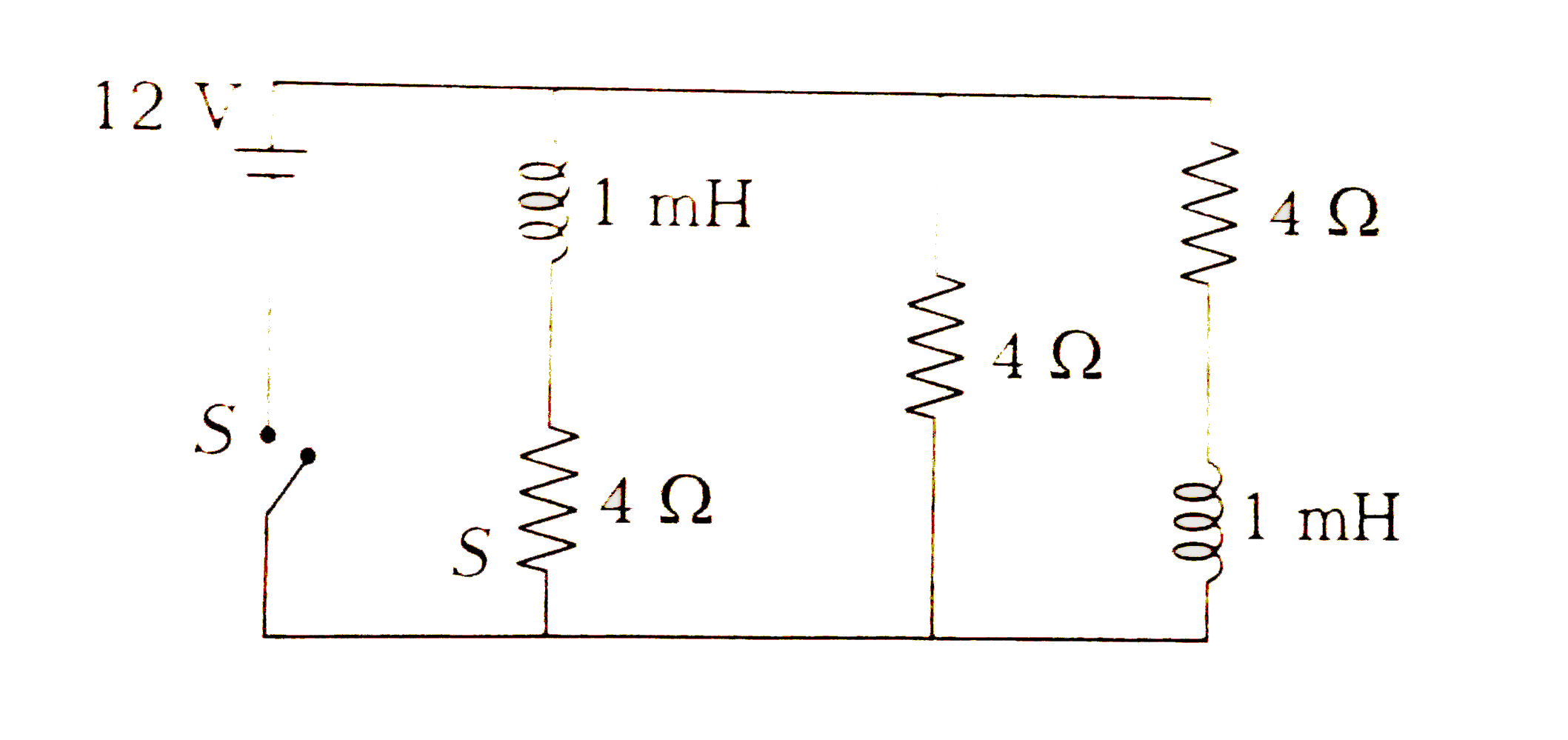
View SolutionFind current through the battery
(a) just after the switch is closed
(b) long after the switch has been closed
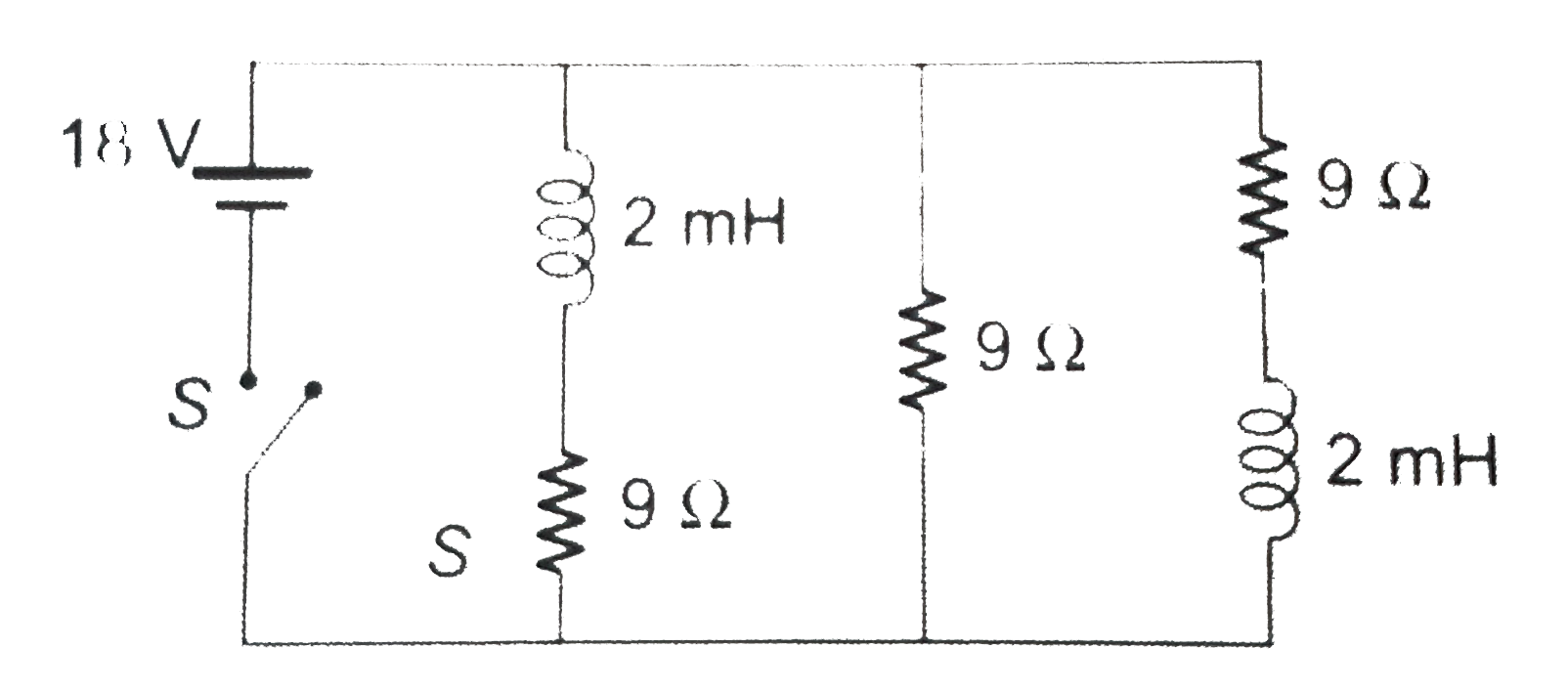
View SolutionFind current through the battery
(a) just after the switch is closed
(b) long after the switch has been closed

View Solution
