Two ploarising sheets are kept with their polarising direction parallel such that the intensity of transmitted light is maximum. Calculate the angle through which one of the sheets must be turned if the intensity of transmitted light is to drop by 14 ?
To solve the problem of how much one of the polarising sheets must be turned to reduce the intensity of transmitted light by 1/4, we will use Malus's Law. Here’s the step-by-step solution:
Step 1: Understand the Initial Condition
When two polarising sheets are aligned parallel to each other, the intensity of the transmitted light is at its maximum. Let the initial intensity of the light be I0.
Step 2: Apply Malus's Law
According to Malus's Law, the intensity I of light transmitted through a polariser is given by:
I=I0cos2(θ)
where θ is the angle between the light's polarisation direction and the axis of the polariser.
Step 3: Define the Condition for Intensity Drop
We want the transmitted intensity to drop to 14 of the initial intensity:
I=14I0
Step 4: Set Up the Equation
Substituting into Malus's Law:
14I0=I0cos2(θ)
Step 5: Simplify the Equation
We can cancel I0 from both sides (assuming I0≠0):
14=cos2(θ)
Step 6: Solve for cos(θ)
Taking the square root of both sides gives:
cos(θ)=±12
Step 7: Find the Angles
The values of θ that satisfy cos(θ)=12 are:
θ=60∘andθ=120∘
Conclusion
Thus, the angle through which one of the sheets must be turned to reduce the intensity of transmitted light by 14 is either 60∘ or 120∘.
---
When two polarising sheets are aligned parallel to each other, the intensity of the transmitted light is at its maximum. Let the initial intensity of the light be I0.
According to Malus's Law, the intensity I of light transmitted through a polariser is given by:
I=I0cos2(θ)
where θ is the angle between the light's polarisation direction and the axis of the polariser.
We want the transmitted intensity to drop to 14 of the initial intensity:
I=14I0
Substituting into Malus's Law:
14I0=I0cos2(θ)
We can cancel I0 from both sides (assuming I0≠0):
14=cos2(θ)
Taking the square root of both sides gives:
cos(θ)=±12
The values of θ that satisfy cos(θ)=12 are:
θ=60∘andθ=120∘
Thus, the angle through which one of the sheets must be turned to reduce the intensity of transmitted light by 14 is either 60∘ or 120∘.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICALEXERCISE - PRACTICE PROBLEMS (1)23videos
WAVE OPTICAL
- BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICALEXERCISE - PRACTICE PROBLEMS (2)10videos
WAVE OPTICAL
- BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION12videos
SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS
Similar Questions
Two polarising sheets are placed with their planes parallel, so that light intensity transmitted is max. Through what angle must either sheet be turned so that light intensity drops to half the maximum value ?
View SolutionThe orientation between a polariser and an analyser is such that the intensity of transmitted light is maximum. On rotating the analyser through 60∘, what fraction of maximum light will be transmitted?
View Solution
Knowledge Check
- Question 1 - Select One
Three polarizing sheets are placed in a stack with the polarizing directions of the first and third perpendicular to each other. What angle should the polarizing direction of the middle sheet make with the polarizing direction of the first sheet to obtain maximum transmitted intensity when unpolarized light is incident on the stack ?
A0B30∘C45∘D60∘ - Question 2 - Select One
When a polaroid sheet is rotated between two crossed polaroids, the intensity of the transmitted will be maximum, when angle θ between pass axes is
Aπ2B3π4Cπ4D2π3 - Question 3 - Select One
The graph showing the dependence of intensity of transmitted light on the angle between polariser and analyser is
A B
B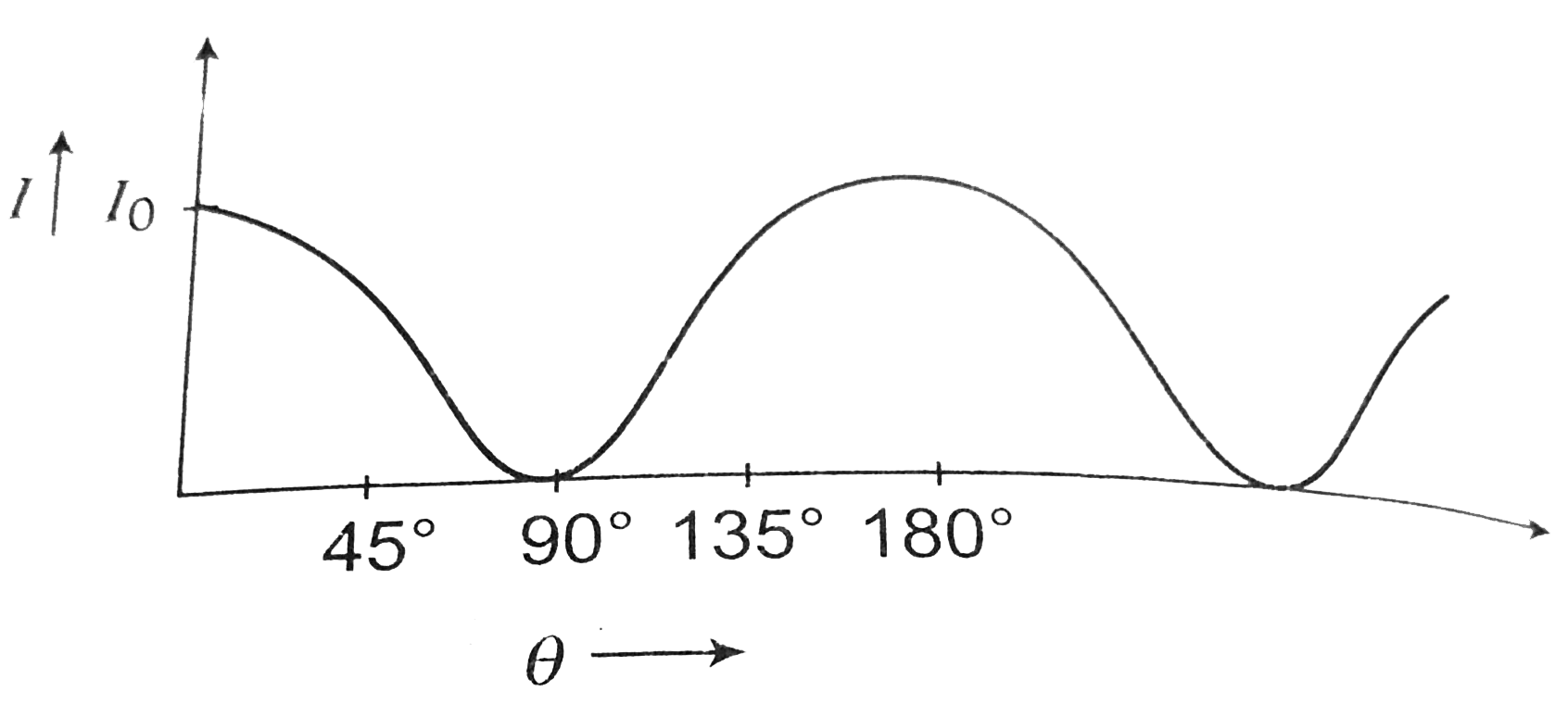 C
C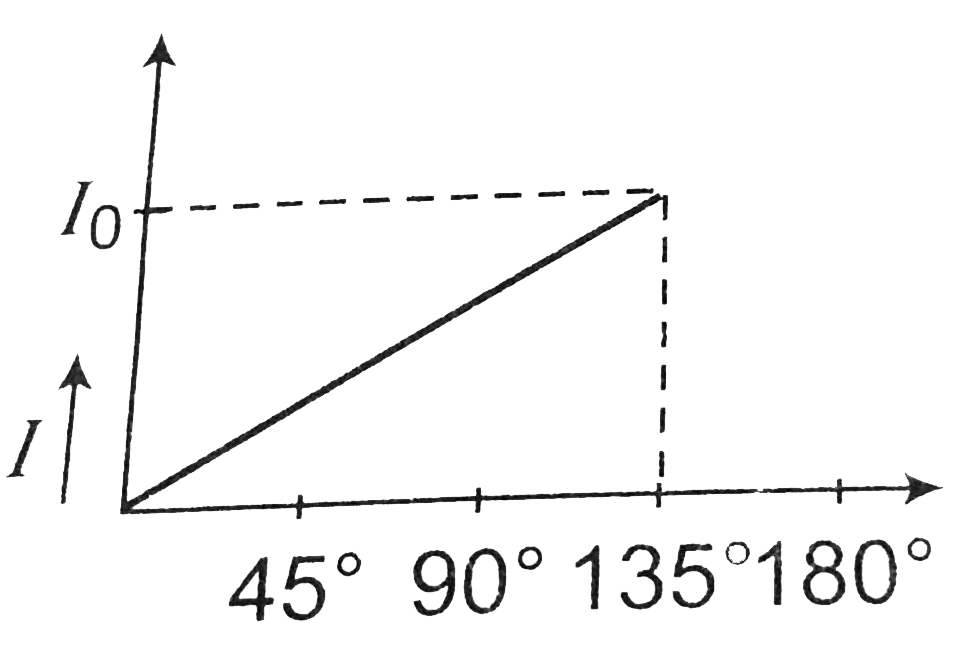 D
D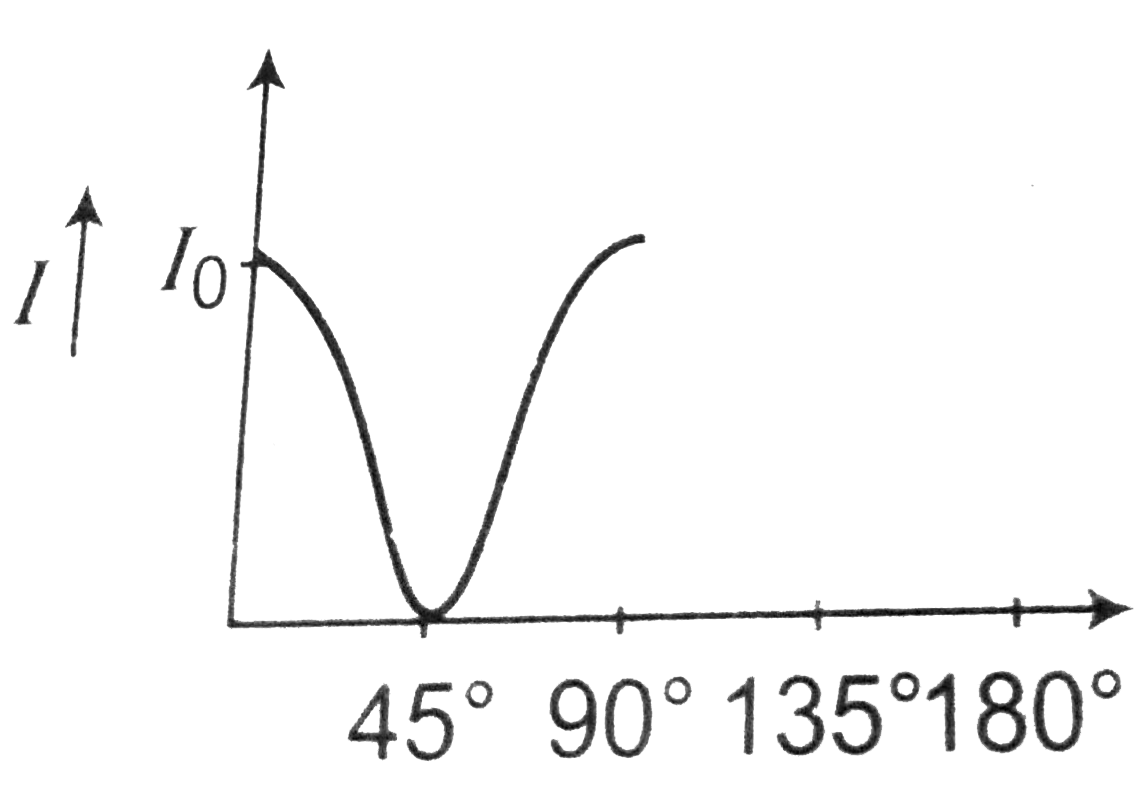
Unplarised light falls on two polarising sheets placed one on the top of the other what must be the angle between the characteristic directions of the sheets if the intensity of the transmitted light is (a) one-third of the maximum intensity of the transmitted beam (b) one third of the intensity of the incident beam.
View SolutionDefine the term 'linearly polarised light'.
When does the intensity of transmitted light become maximum, when a polaroid sheet is rotated between two crossed polaroids ?View SolutionAn unpolarized light with intensity 2I0 is passed through a polaroid. The resultant intensity of the transmitted light will be
View SolutionDisuss the intensity of transmitted light when a polaroid sheet is rotated between two crossed polaroids ?
View SolutionFind an expression for intensity of transmitted light when a polaroid sheet is rotated between I polaroids. In which position of the polaroid sheet will the transmitted intensity be maximum ?
View Solution

