Two slits are made on an opaque surface at a separation of 1 mm from each other. A screen is placed at a distance 1 m from the plane of the slits. A small hole is made on the screen at a distance 1 mm from the centre of the screen as shown in the figure. If we observe the light coming out from hole on the other side, then which wavelengths in the visible region will be absent and which will show strong presence ? Wavelength of visible light ranges from 400 nm to 700 nm.

Video Solution

Text Solution

Verified by Experts

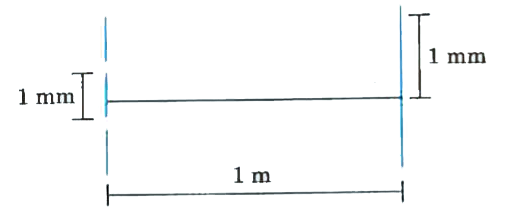
Path difference between two waves from slits reaching the hole can be written as follows :
$Δ x = \frac{y d}{D} = \frac{1 \times 10^{- 3} \times 1 \times 10^{- 3}}{1} = 10^{- 6}$
(A) Wavelengths interfering destructively at the location of hole will be absent from light coming out from hole.
Destructive interference $\Rightarrow Δ x = (2 n + 1) λ / 2$ .
$(2 n + 1) \frac{λ}{2} = 10^{- 6}$
By substituting minimum and maximum wavelengths of visible region in the above relation, we get corresponding maximum and minimum values of n and then we can find integers in this range of values.
$\Rightarrow (2 n_{min} + 1) \times 700 \times 10^{- 9} = 2 \times 10^{- 6}$
$\Rightarrow 2 n_{min} + 1 = 2.86$
$\Rightarrow 2 n_{min} = 1.86$
$\Rightarrow n_{min} = 0.93$
Similarly we can proceed to get $n_{max}$
$\Rightarrow (2 n_{max} + 1) \times 400 \times 10^{- 9} = 2 \times 10^{- 6}$
$\Rightarrow (2 n_{max} + 1) = 5$
$\Rightarrow n_{max} = 2 ... ... (1)$
Integers in the above-calculated range are $n = 1$ and 2, corresponding wavelenghts can be calculated by substituting back in the same relation.
$(2 n + 1) λ = 2 \times 10^{- 6} \Rightarrow (2 \times 1 + 1) λ_{1} = 2 \times 10^{- 6} \Rightarrow λ_{1} = 667 n m$
$(2 n + 1) λ = 2 \times 10^{- 6} \Rightarrow (2 \times 2 + 1) λ_{2} = 2 \times 10^{- 6} \Rightarrow λ_{2} = 400 n m$
The above-calculated two wavelengths will be absent from light coming out from the hole.
(B) Wavelengths interfering constructively at the location of the hole will be present strongly in the light coming out from the hole.
Constructive interference, $Δ x = n λ$
$n λ = 10^{- 6} ... . (1)$
By substituting minimum and maximum wavelengths of visible region in the above relation, we get corresponding maximum and minimum values of n and then we can find intergers in this range of values.
$\Rightarrow n_{min} \times 700 \times 10^{- 9} = 1 \times 10^{- 6}$
$\Rightarrow n_{min} = 1.43$
Similarly we can proceed to get $n_{max}$
$\Rightarrow n_{max} \times 400 \times 10^{- 9} = 1 \times 10^{- 6}$
$\Rightarrow n_{max} = 2.5$
Only integer in the above calculated range is 2 and corresponding wavelength can be calculated by substituting back in the same relation.
$n λ = 10^{- 6} \Rightarrow 2 λ = 10^{- 6} \Rightarrow λ = - 500 n m$
Hence 500 nm wavelength will be strongly present in the light coming out from hole.

Updated on:21/07/2023

In Young' s double-slit experiment, separation between the two slits is d and separation between plane of the slits and the screen is D. A small hole is made on the screen at a point directly in front of one of the slit. Some wavelengths are missing from the light coming out from hole on the other side. These wavelengths are
A $\frac{d^{2}}{D}$
B $\frac{d^{2}}{2 D}$
C $\frac{d^{2}}{3 D}$
D $\frac{d^{2}}{4 D}$
Two coherent light sources $S_{1}$ and $S_{2} (λ = 6000 Å)$ are $1 m m$ apart from each other. The screen is placed at a distance of $25 c m$ from the sources. The width of the fringes on the screen should be
A(a) $0.015 c m$
B(b) $0.025 c m$
C(c) $0.010 c m$
D(d) $0.030 c m$
The distance between two coherent sources is 1 mm. The screen is placed at a distance of 1 m from the sources. If the distance of the third bright fringe is $1.2$ mm from the central fringe, the wavelength of light used is
A $4000 Å$
B $5000 Å$
C $6000 Å$
D $7200 Å$