There is a hemisphere whose radius of curvature is r and inner side of it is made completely reflecting . A point source of light of power P is kept at the centre of curvature of hemisphere . Calculate the force exerted by the light falling on inner side of hemispherical surface.

Text Solution

Verified by Experts

If P is power of point source , then intensity of light which means energy crossing per unit area per unit time can be written as $P / 4 π r^{2}$ . Let us select a small are $Δ A$ at angle $θ$ with the vertical radius of hemisphere as shown in the following figure:

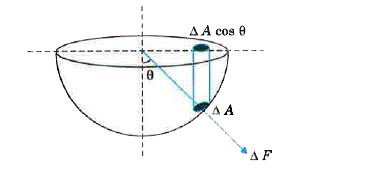
Amount of energy falling on this area will be $\frac{P Δ A}{4 π r^{2}}$
per unit time . Hence , amount of linear momentum imparted to the surface per unit time can be written as $\frac{P Δ A}{4 π r^{2} c}$ . Since surface is completely reflecting and hence it will reflect back the light and hence rate of change of linear momentum will be double to this value , hence equal to $\frac{2 P Δ A}{4 π r^{2} c}$ . From Newton.s second law , we known that rate of chane of linear momentum is equal to the force exerted , and hence force exerted by the light on the surface area $Δ A$ selected can be written as follows :
$Δ F = \frac{2 P Δ A}{4 π r^{2} c}$
We can see that horizontal component of this force $Δ F \sin θ$ will be balanced by the force on corresponding area selected on hemisphere due to symmetry , and hence contribution to the net force by this selected area will be $Δ F \cos θ$ and direction will be vertically downward as per figure . Net vertical downward force applied by the light on this hemisphere can be calculated by summig up forces on such small areas selected .
$F = \sum Δ F \cos θ = \sum \frac{2 P Δ A \cos θ}{4 π r^{2} c}$
$F = \frac{2 P}{4 π r^{2} c} \sum Δ A \cos θ ... (i)$
In the above expression , we can see that $Δ A \cos θ$ is projected area of selected are $Δ A$ as shown in figure .
We can understand that total projected area will be $π r^{2}$ and hence force can be written as follows :
$F = \frac{2 P}{4 π r^{2} c} π r^{2} = \frac{P}{2 c}$

Updated on:21/07/2023

A perfectly reflecting solid hemisphere of radius R is placed in the path of a parallel beam of light of large aperture. If the beam carrries an intensity l, find the force exerted by the beam on the hemisphere.
A $\frac{2 π R^{2} l}{c}$
B $\frac{π R^{2} l}{c}$
C $\frac{4 π R^{2} l}{c}$
DNone of these