When light off frequency v1 is incident on a photosensitive surface , the stopping potential is V1 . If the frequency of incident radiation becomes v1/2 the stopping potential changes to V2 Find out the expression for the threshold frequency for the surface in terms of V1andV2
If the frequency of incident radiation is doubled , will the maximum kinetic energy of the photoelectrons also be doubled ? Given reason .
To solve the problem, we will follow these steps:
Step 1: Understand the Photoelectric Effect
According to the photoelectric effect, the maximum kinetic energy (K.E.) of the emitted photoelectrons can be expressed as:
K.E.=hν−hν0
where:
- h is Planck's constant,
- ν is the frequency of the incident light,
- ν0 is the threshold frequency.
The stopping potential V is related to the maximum kinetic energy by:
K.E.=eV
where e is the charge of an electron.
Step 2: Set Up the Equations
When the frequency of the incident light is ν1, the stopping potential is V1:
hν1−hν0=eV1(1)
When the frequency is ν12, the stopping potential is V2:
h(ν12)−hν0=eV2(2)
Step 3: Rearranging the Equations
From equation (1):
hν1=eV1+hν0(3)
From equation (2):
hν12=eV2+hν0
Multiplying through by 2 gives:
hν1=2eV2+2hν0(4)
Step 4: Equate the Two Expressions for hν1
From equations (3) and (4):
eV1+hν0=2eV2+2hν0
Step 5: Solve for the Threshold Frequency ν0
Rearranging gives:
eV1−2eV2=hν0
Thus, we can express ν0 in terms of V1 and V2:
hν0=eV1−2eV2
Dividing through by h:
ν0=eV1−2eV2h
Final Expression
Thus, the expression for the threshold frequency ν0 in terms of V1 and V2 is:
ν0=e(V1−2V2)h
Step 6: Analyze the Effect of Doubling the Frequency
If the frequency of the incident radiation is doubled, say to 2ν1, the maximum kinetic energy becomes:
K.E.=h(2ν1)−hν0
This can be expressed as:
K.E.=2hν1−hν0
Comparing this with the original kinetic energy:
K.E.=hν1−hν0
The difference in kinetic energy when the frequency is doubled is:
K.E.=(2hν1−hν0)−(hν1−hν0)=hν1
Thus, the maximum kinetic energy increases by hν1 but does not double.
Conclusion
The maximum kinetic energy of the photoelectrons does not double when the frequency is doubled; it increases by an amount equal to the energy corresponding to the original frequency ν1.
According to the photoelectric effect, the maximum kinetic energy (K.E.) of the emitted photoelectrons can be expressed as:
K.E.=hν−hν0
where:
- h is Planck's constant,
- ν is the frequency of the incident light,
- ν0 is the threshold frequency.
K.E.=eV
where e is the charge of an electron.
When the frequency of the incident light is ν1, the stopping potential is V1:
hν1−hν0=eV1(1)
h(ν12)−hν0=eV2(2)
From equation (1):
hν1=eV1+hν0(3)
hν12=eV2+hν0
Multiplying through by 2 gives:
hν1=2eV2+2hν0(4)
From equations (3) and (4):
eV1+hν0=2eV2+2hν0
Rearranging gives:
eV1−2eV2=hν0
Thus, we can express ν0 in terms of V1 and V2:
hν0=eV1−2eV2
Dividing through by h:
ν0=eV1−2eV2h
Thus, the expression for the threshold frequency ν0 in terms of V1 and V2 is:
ν0=e(V1−2V2)h
If the frequency of the incident radiation is doubled, say to 2ν1, the maximum kinetic energy becomes:
K.E.=h(2ν1)−hν0
This can be expressed as:
K.E.=2hν1−hν0
K.E.=hν1−hν0
The difference in kinetic energy when the frequency is doubled is:
K.E.=(2hν1−hν0)−(hν1−hν0)=hν1
The maximum kinetic energy of the photoelectrons does not double when the frequency is doubled; it increases by an amount equal to the energy corresponding to the original frequency ν1.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Revision Exercises (Long Answer Questions)
3 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Revision Exercises (Numerical Problems)
20 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Revision Exercises (Fill in the Blanks)
11 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Chapter Practice Test
15 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
Similar Questions
If frequency of radiation incident on a photosensitive surface is doubled, the maximum kinetic energy of emitted photoelectrons also gets doubled.
View SolutionKinetic energy of surface photoelectrons is x when frequency of incident radiation is v .If frequency of incident radiation is made 2v, the kinetic energy of surface photoelectron will be
View Solution
Knowledge Check
Which of the following is the graph between the frequency (v) of the incident radiations and the stopping potential ?
A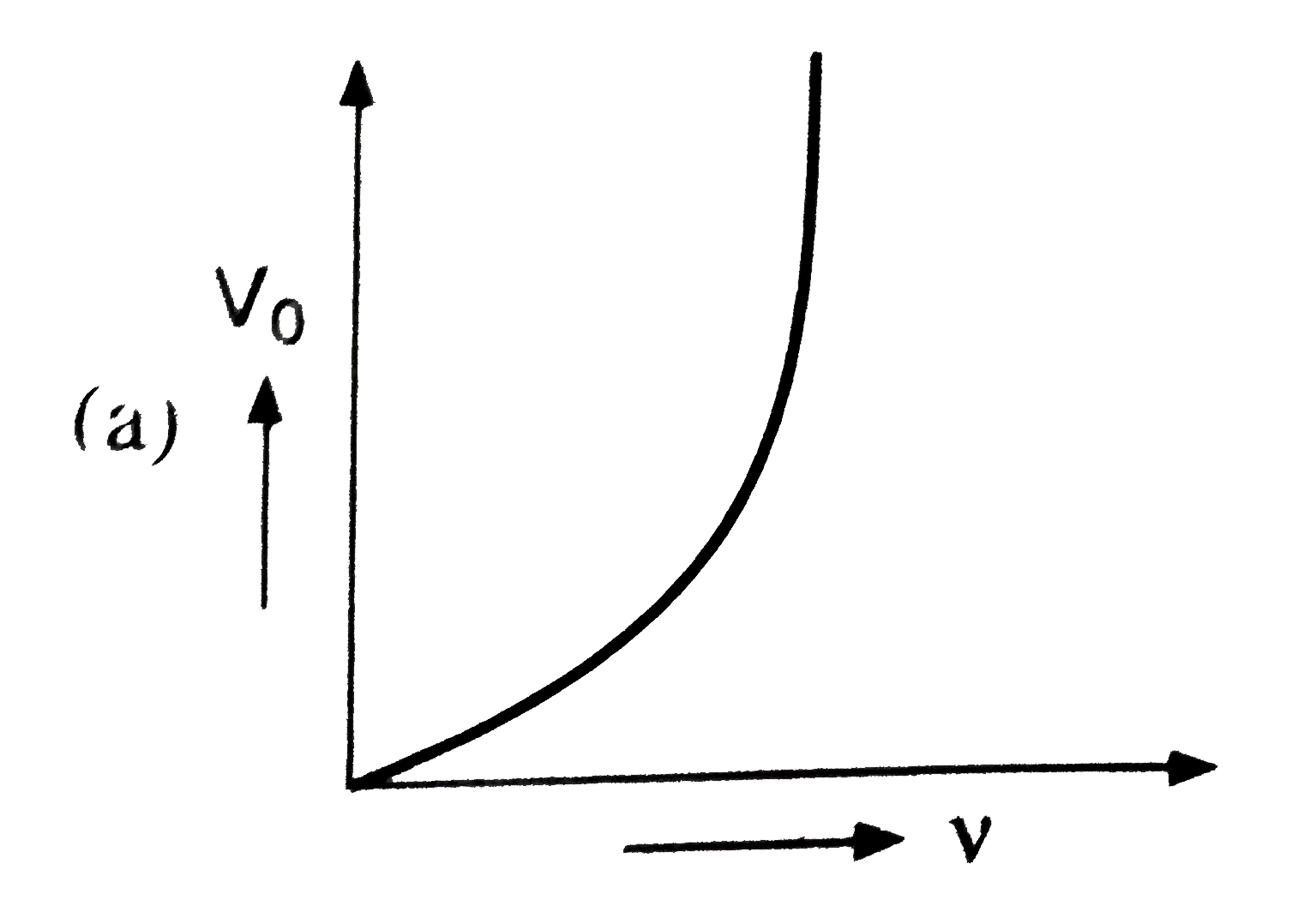 B
B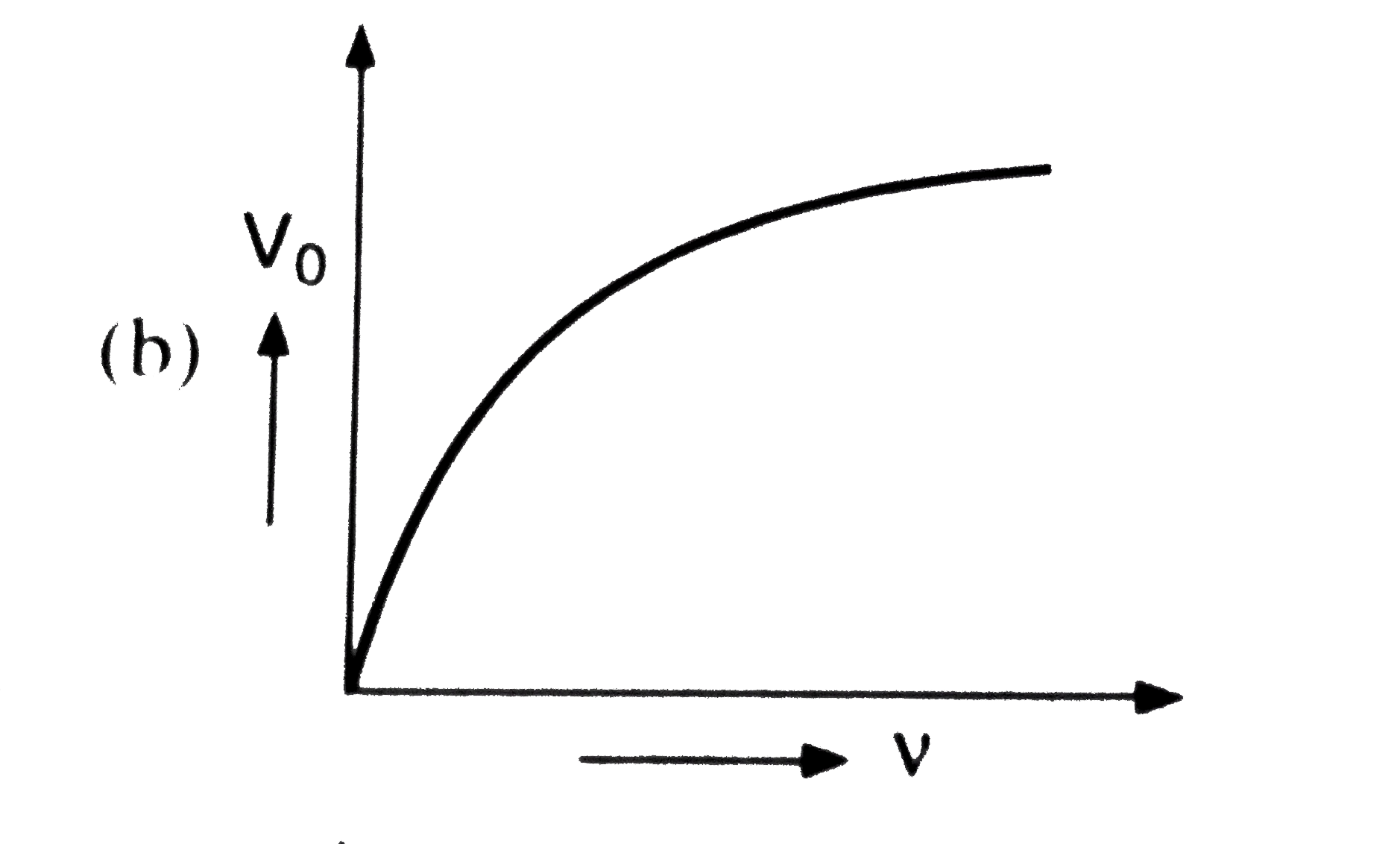 C
C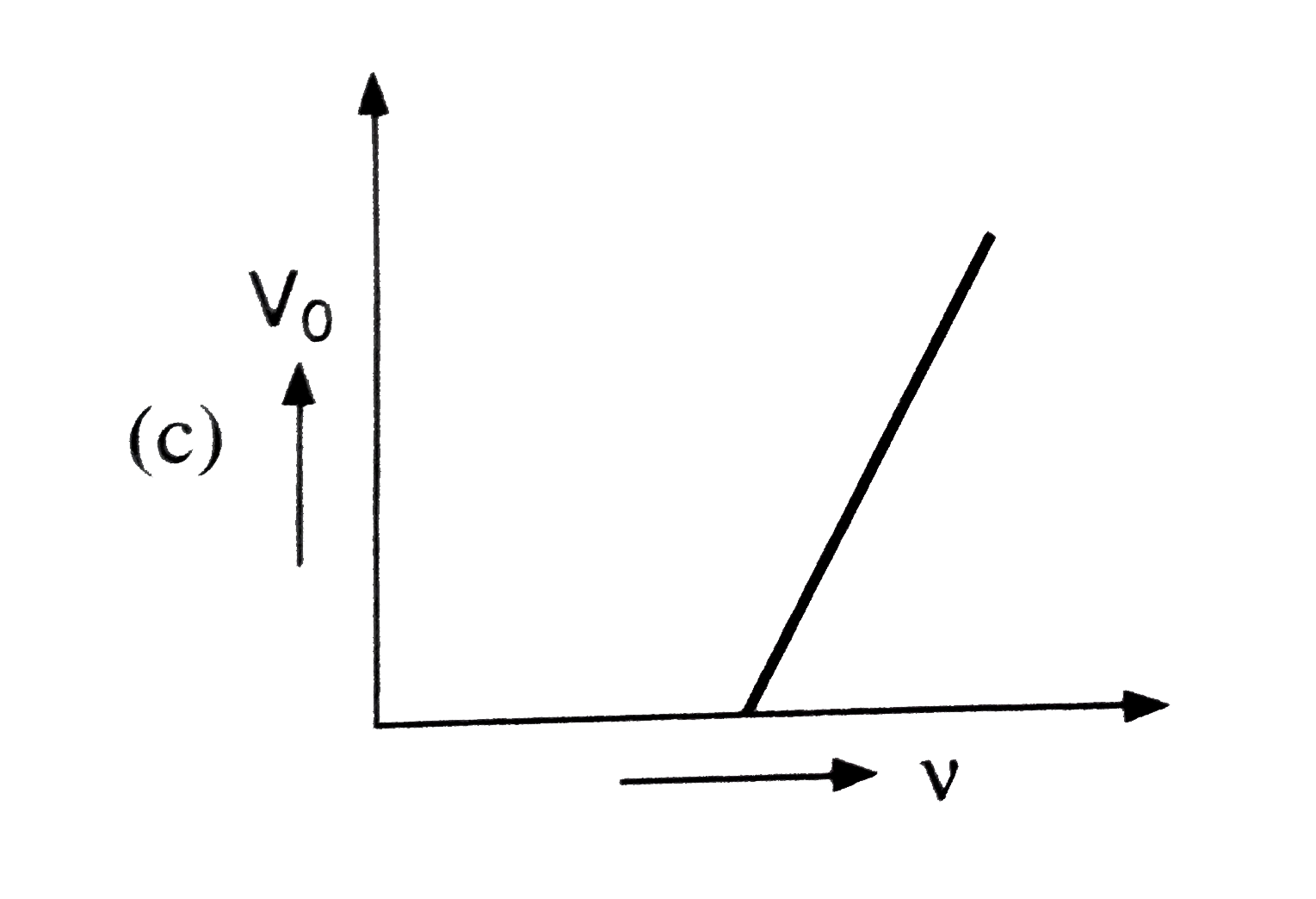 D
D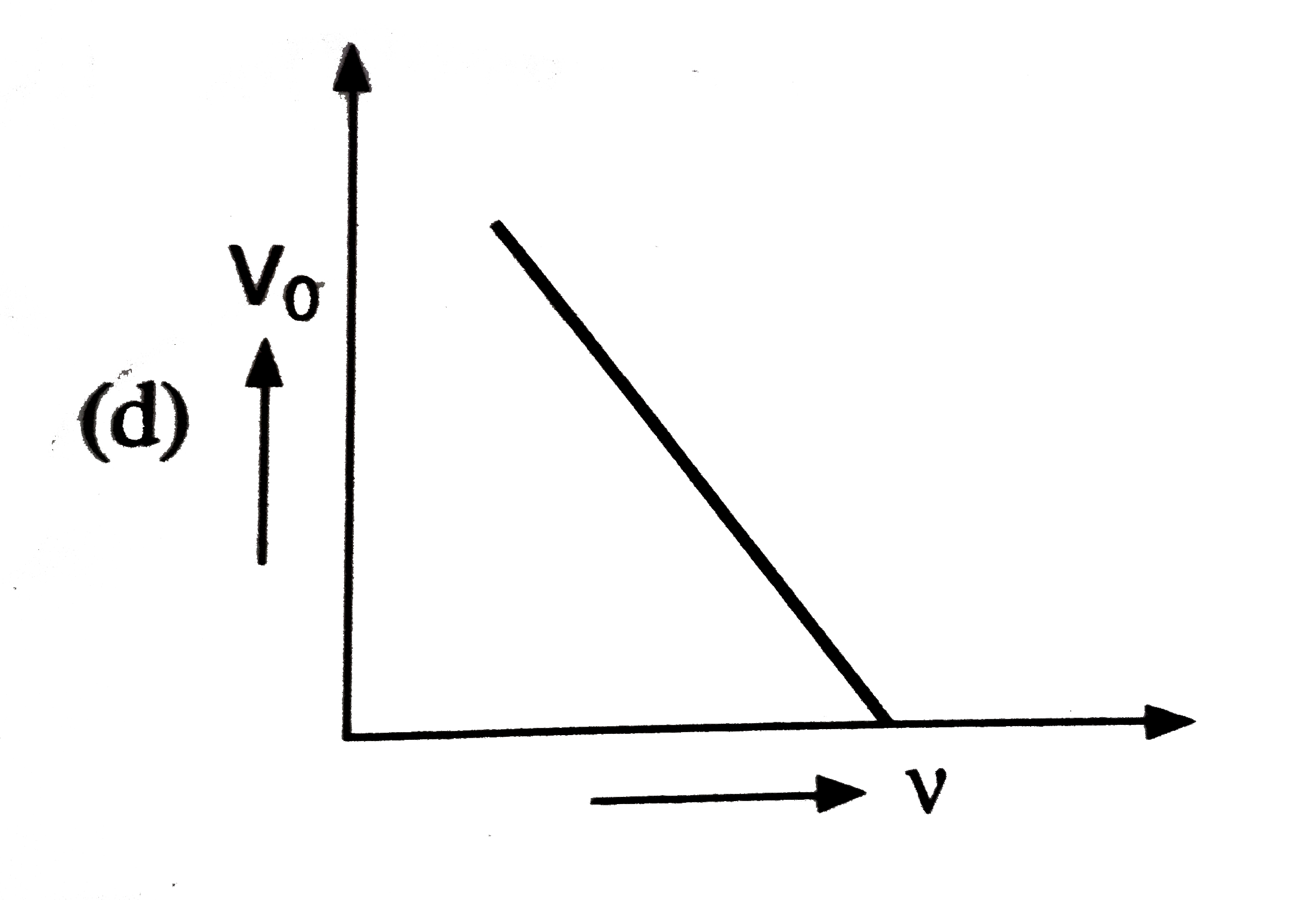
When light of wavelength λ is incident on photosensitive surface, the stopping potential is V. When light of wavelength 3λ is incident on same surface, the stopping potential is V6Thereshould wave length for the surface is
A2λB3λC4λD5λThe photoelectric threshold frequency of a metal is v0. When ligth of frequency 3v0 is incident on the metal, the maximum kinetic energy of emitted photoelectrons will be
Ahv0B2hv0C3hv0D12hv0
The stopping potential for acertain photosensitive metal is Vq when the frequency of incident radiation is V0 When the frequency of the incident radiations is doubled, what will be the stopping potential ?
View SolutionThe graph shows variation of stopping potential V0 versus frequency of incident radiation v for two photosensitive metals A and B. Which of two metals has higher threshold frequency and why?
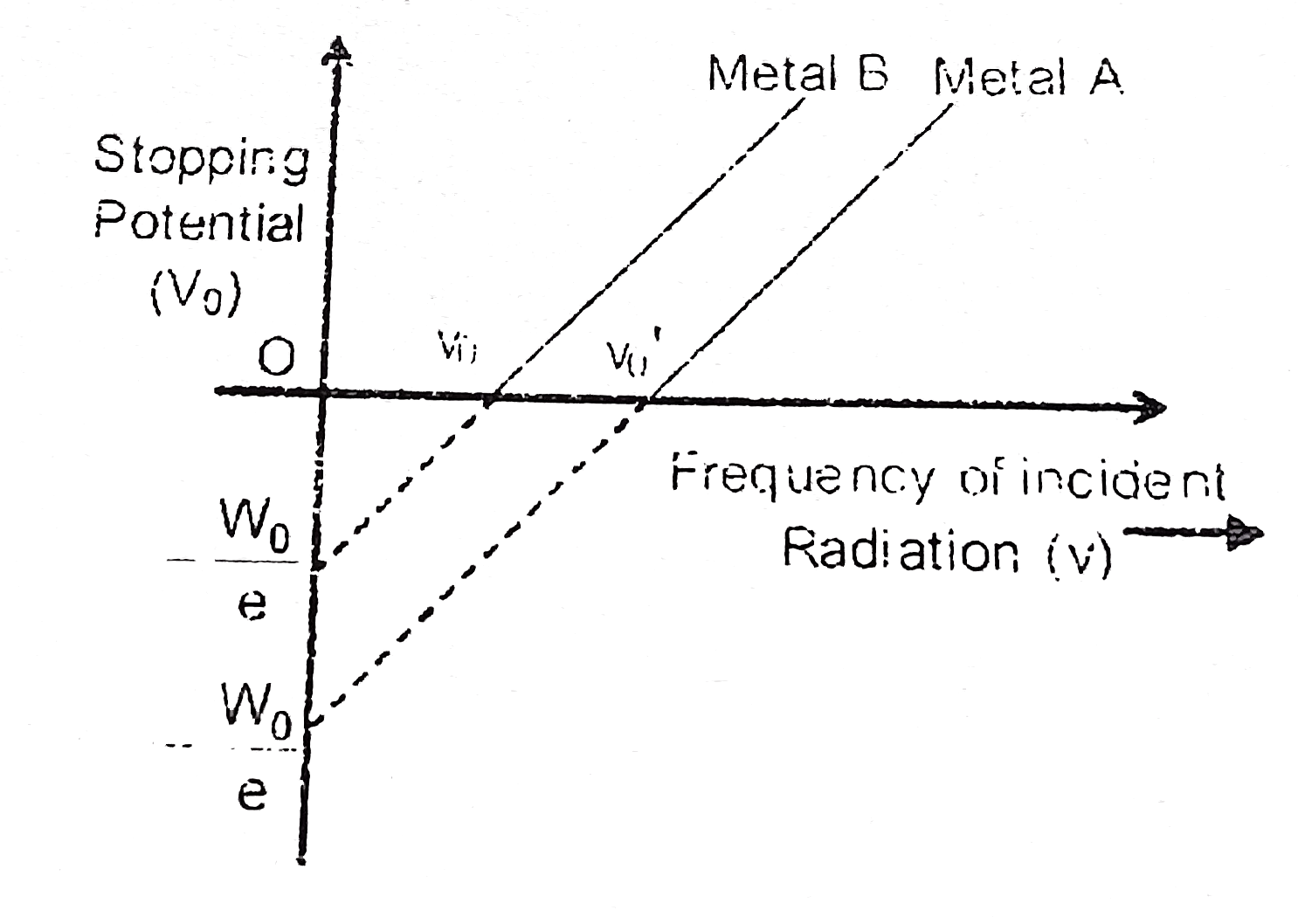
View SolutionWhen light of frequency v1 incident on a metal with work function W0 (where hv1>W0), the photocurrent falls to zero at a stopping potential of V1. If the frequency of light is increased to v2, the stopping potential changes to V2. Therefore, the charge of an electron is given by
View SolutionThe photoelectric threshold frequency of a metal is v. When light of frequency 4v is incident on the metal . The maximum kinetic energy of the emitted photoelectrons is
View SolutionThe photoelectric threshold frequency of a metal is v. When light of frequency 4v is incident on the metal . The maximum kinetic energy of the emitted photoelectrons is
View Solution
