If the gravitational force is assumed to vary as the nth power of the distance, then the time period of a planet round the sun will be proportional to : Solution in Kannada
Rn
R−n
Rn+12
(R)n−12
The correct Answer is:C
Gravitational force provides the necessary centripetal force
∴mv2R=GMmRn⇒v=√GMRn−1
Now T=2πRv=2πR√GMRn−1=2π√Rn+1GM
⇒T∝R(n+12)
Thus correct choice is (c ).
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Multiple Choice Questions (LEVEL-II)64videos
GRAVITATION
- BOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Multiple Choice Questions (LEVEL-III Questions From AIEEE/JEE Examination)6videos
GRAVITATION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS39videos
ELECTROSTATICS
- BOOK - MODERN PUBLICATIONCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - Recent Competitve Questions17videos
HEAT AND THERMODYNAMICS
Similar Questions
In order that a body of 15 kg weighs zero at the equator, then the angular speed of earth is :
(g=10ms−1)02:37View SolutionTwo bodies of masses m and 4 m are placed at a distance r. The gravitational potential at a point on the line joining them where the gravitational field is zero is :
01:35View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
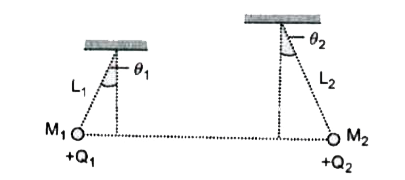 05:38View Solution
05:38View SolutionThe temperature of a gas contained in a closed vessel of constant volume increases by 1∘C when the pressure of the gas is increased by 1%. The initial temperature of the gas is:
01:13View SolutionSuppose the gravitational force varies inversely as the nth power of distance. Then the time period of a planet in circular orbit of radius 'R' around the sun will be proportional to
03:45View Solutionif the gravitaional force ware to vary inversely as m^(th) power of the distance then the time period of a planet in circular orbit of radius r aroung the sun will be proportional to
03:05View SolutionSuppose the gravitational force varies inversely as the n^(th) power of distance. Then the time period of a planet in circular orbit of radius R around the sun will be proportional to-
03:05View SolutionSuppose the gravitational force varies inverseley as the nth power of the distance. Show that the time period of a planet in circular orbit of raidus r arount the sun will be proportional to r^(n+1)//^(2) .
03:56View SolutionSuppose the gravitational force varies inversely as then n th power of distance then the time period of a planet in circular orbit of radius r around the sun will be propotinal to
View Solutionमान लीजिए गुरुत्वाकर्षण बल दूरी की nवीं घात के व्युत्क्रमानुपाती है । तब सूर्य की R त्रिज्या की वृत्तीय कक्षा में परिक्रमा करने वाले किसी ग्रह का आवर्तकाल अनुक्रमानुपाती होगा -
02:43View Solutionमान लीजिए, गुरुत्वाकर्षण बल दूरी की n वी घात के व्युत्क्रमानुपाती है, तब सूर्य की, R त्रिज्या की वृत्तीय कक्षा में परिक्रमा करने वाले किसी ग्रह का आवर्तकाल अनुक्रमानुपाती होगा
05:27View SolutionSuppose the gravitational force varies inversely as the n th power of the distance. Thus the time period of a planet moving in a circular orbit of radius r around the sun will be proportional to
05:21View SolutionIf the gravitational force varies inversely as the nth power of the distance then, the time period T of a satellite revolving in a circular orbit round the earth at distance r is proportional to :
01:07View SolutionSuppose the gravitational force varies inversely as the nth power of distance. Then the time period of planet in circular orbit of radius R around the sun will be proportional to ..........
03:09View SolutionSuppose the gravitational force varies inversely as the nthpower of distance. Then the time period of a planet in circular orbit of radius R around the sun will be proportional to-
02:48View Solution

