If the potential energy of body on a planet is numerically U and the escape velocity for the same body is ve for same planet then Uve will be : Solution in Kannada
m√GM2R
m√GMR
m√2GMR
mGMR
The correct Answer is:A
Potential energy of body
U=GMmR (numerically)
Escape velocity is ve=√2GMR
∴Uve=GMmR×√R2GM
∴Uve=m√GM2R.
Thus correct choice is (a).
Topper's Solved these Questions
- GRAVITATIONBook:MODERN PUBLICATIONChapter:GRAVITATIONExercise:Multiple Choice Questions (LEVEL-II)
Explore 64 Videos
- GRAVITATIONBook:MODERN PUBLICATIONChapter:GRAVITATIONExercise:Multiple Choice Questions (LEVEL-III Questions From AIEEE/JEE Examination)
Explore 6 Videos
- ELECTROSTATICSBook:MODERN PUBLICATIONChapter:ELECTROSTATICSExercise:RECENT COMPETITIVE QUESTIONS
Explore 39 Videos
- HEAT AND THERMODYNAMICSBook:MODERN PUBLICATIONChapter:HEAT AND THERMODYNAMICSExercise:Recent Competitve Questions
Explore 17 Videos
Similar Questions
In order that a body of 15 kg weighs zero at the equator, then the angular speed of earth is :
(g=10ms−1)
View SolutionTwo bodies of masses m and 4 m are placed at a distance r. The gravitational potential at a point on the line joining them where the gravitational field is zero is :
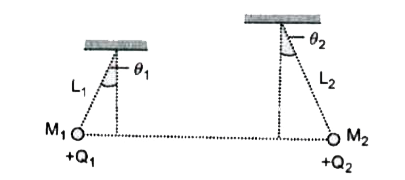
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionThe temperature of a gas contained in a closed vessel of constant volume increases by 1∘C when the pressure of the gas is increased by 1%. The initial temperature of the gas is:
View SolutionThe ratio of escape velocity at earth (v_(e)) to the escape velocity at a planet (v_(y)) whose radius and density are twice
View SolutionStatement-1:The escape speed of a body of mass m is ve. The escape speed of another body of mass 2m for same planet is ve.
Statement-2: The escape speed of a body for a given planet is independent of mass of body.
View SolutionIf ve is the escape velocity of a body from a planet of mass 'M' and radius 'R. Then the velocity of the satellite revolving at height 'h' from the surface of the planet will be
View SolutionAcceleration due to gravity on a planet is 10 times the value on the earth. Escape velocity for the planet and the earth are Vp and Ve respectively. Assuming that the radii of the planet and the earth are the same, then -
View SolutionThe escape velocity of a body on an imaginary planet which is thrice the radius of the earth and double the mass of the earth is (ve is the escape velocity of earth)
View SolutionThe radius of a planet is double that of the earth but then average densities are the same. If the escape velocities at the planet and at the earth are vP and vE respectively, then prove that vP=2vE.
View SolutionIf the gravitational potential energy of a body on a planet is numerically equal to . U and the escape velocity of the same body is V_(e') then
View SolutionThe escape velocity of a body from the earth is Ve. The escape velocity of a planet whose mass and radius are twice those of the earth is
View SolutionFor a planet, the escape velocity is Ve. The presence of atmosphere on the planet shows that the
View SolutionThe escape velocity from a planet is v_(0) . The escape velocity from a planet having twice the radius but same density will be
View SolutionThe escape velocity of a body on an imaginary planet which is thrice the radius of the earth and double the mass of the earth is ( v_(e ) is the escape velocity of earth) :
View Solution