Thermal resistance of two metallic plates of same material and cross -section joined in series are 3 and 4 respectively. What is thermal resistance of the combination ? Solution in Kannada
3/4
4/3
7
1/7
The correct Answer is:C
In series R5=R1+R2 R5=3+4=7
Thus correct choice is (c).
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Multiple Choice Questions (Level - III) Questions From AIEEE/JEE Examination)5videos
TRANSFERENCE OF HEAT
- BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Recent Competitive Questions4videos
TRANSFERENCE OF HEAT
- BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Recent Competitive Questions4videos
TRANSFERENCE OF HEAT
- BOOK - MODERN PUBLICATIONCHAPTER - SOLIDS & SEMICONDUCTOR DEVICESEXERCISE - Revision Test28videos
SOLIDS & SEMICONDUCTOR DEVICES
- BOOK - MODERN PUBLICATIONCHAPTER - UNIFORM CIRCULAR MOTIONEXERCISE - Revision Test57videos
UNIFORM CIRCULAR MOTION
Similar Questions
A composite block is made of slabs A,B,C,D and E of different thermal conductivities (given in terms of a constant K) and sizes (given in terms of length, L) as shown in the figure. All slabs are of same width. Heat 'Q' flows only from left to right through the blocks.
Then in steady state
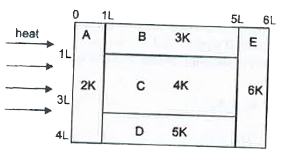 01:47View Solution
01:47View SolutionTwo slabs are of the thicknesses d1 and d2. Their thermal conductivities are K1 and K2 respectively They are in series. The free ends of the combination of these two slabs are kept at temperatures θ1 and θ2. Assume θ1>θ2. The temperature θ of their common junction is
02:10View SolutionTwo slabs are of the thicknesses d1 and d2. Their thermal conductivities are K1 and K2 respectively They are in series. The free ends of the combination of these two slabs are kept at temperatures θ1 and θ2. Assume θ1>θ2. The temperature θ of their common junction is
02:02View SolutionChoose the forward biased p-n junction :
03:36View SolutionA sphere of mass m is tied to end of a string of length 1 and rotated through the other and along a horizontal circular path with speed . The work done in full horizontal circle is:
02:28View SolutionA metal rod of area of cross section A has length L and coefficient of thermal conductivity K the thermal resistance of the rod is .
03:58View SolutionTwo metal plates of same area and thickness l_(1) and l_(2) are arranged in series If the thermal conductivities of the materials of the two plates are K_(1) and K_(2) The thermal conductivity of the combination is .
06:21View SolutionThe ratio of thermal conductivity of two rods of different material is 5:4. The two rods of same area of cross-section and same thermal resistance will have the lengths in the ratio
02:41View SolutionTwo wires of same metal have the same length but their cross- sections are in the ratio 1 :3 . They are joined in series. The resistance of the thicker wire is 10Ω . The total resistance of the combination will be
04:23View SolutionTwo wires of the same metal have the same length, but their cross-sections are in the ratio of 3:1. They are joined in series. The resistance of the thicker wire is 10Ω. The total resistance of the combinations will be
02:39View SolutionTwo rods of equal length and diameter have thermal conductivity 3 and 4 units respectively. If they are joined in series, the thermal conductivity of the combination in the given units would be
View Solutionदो उष्मीय चालक जिनके उष्मीय प्रतिरोध क्रमश: व है | (i) श्रेणी क्रम में (ii) समान्तर क्रम में जुड़े है | इनका तुल्य उष्मीय प्रतिरोध क्या है ?
View SolutionTwo wires of the same metal have same length, but their cross-sections are in the ratio 3:1 . They are joined in series. The resistance of thicker wire is 10 Omega . The total resistance of the combination will be
04:53View SolutionThe ratio of thermal conductivity of two rods of different material is 5:4. The two rods of same area of cross-section and same thermal resistance will have the lengths in the ratio
01:41View Solution" A metal rod of area of cross section A has length L and coefficient of thermal conductivity K The thermal resistance of the rod is "
03:35View Solution

