A cylindrical rod is having temperature T1 and T2 at its ends. The rate of flow of heat is Q1 cal/s. If all the linear dimensions are doubled keeping temperatures constant, the rate of flow of heat Q2 will be : Solution in Kannada
4Q1
Q1/4
2Q1
Q1/2.
The correct Answer is:C
dQdt=KA(T1−T2)l=Kπr2(T1−T2)l=Q1
When all dimensions are doubled then dQdt becomes two times i.e. 2Q1
Correct choice is (c ).
Topper's Solved these Questions
- TRANSFERENCE OF HEAT BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Multiple Choice Questions (Level - III) Questions From AIEEE/JEE Examination)
5 Videos
- TRANSFERENCE OF HEAT BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Recent Competitive Questions
4 Videos
- TRANSFERENCE OF HEAT BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Recent Competitive Questions
4 Videos
- SOLIDS & SEMICONDUCTOR DEVICESBOOK - MODERN PUBLICATIONCHAPTER - SOLIDS & SEMICONDUCTOR DEVICESEXERCISE - Revision Test
28 Videos
- UNIFORM CIRCULAR MOTIONBOOK - MODERN PUBLICATIONCHAPTER - UNIFORM CIRCULAR MOTIONEXERCISE - Revision Test
57 Videos
Similar Questions
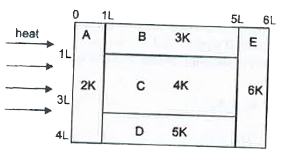
A composite block is made of slabs A,B,C,D and E of different thermal conductivities (given in terms of a constant K) and sizes (given in terms of length, L) as shown in the figure. All slabs are of same width. Heat 'Q' flows only from left to right through the blocks.
Then in steady state
View SolutionTwo slabs are of the thicknesses d1 and d2. Their thermal conductivities are K1 and K2 respectively They are in series. The free ends of the combination of these two slabs are kept at temperatures θ1 and θ2. Assume θ1>θ2. The temperature θ of their common junction is
View SolutionTwo slabs are of the thicknesses d1 and d2. Their thermal conductivities are K1 and K2 respectively They are in series. The free ends of the combination of these two slabs are kept at temperatures θ1 and θ2. Assume θ1>θ2. The temperature θ of their common junction is
View SolutionChoose the forward biased p-n junction :
View SolutionA sphere of mass m is tied to end of a string of length 1 and rotated through the other and along a horizontal circular path with speed . The work done in full horizontal circle is:
View SolutionA cylindrical rod having temperature T1 and T2 at its ends. The rate of flow of heat is Q1cal/sec. If all the linear dimensions are doubled keeping temperature constant, then rate of flow of heat Q2 will be
View SolutionRate of heat flow through a cylindrical rod is H1. Temperatures of ends of rod are T1 and T2. If all the dimensions of rod become double and temperature difference remains same and rate of heat flow becomes H2. Then H1H2 is 0.x. Find value of x.
View SolutionA heat engine absorbs heat Q_(1) at temperature T_(1) and heat Q_(2) at temperature T_(2) . Work done by the engine is (Q_(1)+Q_(2)) . This data
View SolutionA cylinderical rod has temperature T1andT2 at its ends. The rate of flow of heat is Q1 cal/s. if all the linear dimensions are doubled keeping temperature constant then rate of flow of heat Q2 will be
View SolutionA heat engine absorbs heat Q1 at temperature T1 and heat Q2 at temperature T2, work done by the engine is J (Q1+Q2) this data
View SolutionA cylindrical rod is having temperature T1 and T2 at its ends. The rate of flow of heat is Q1 cal/sec. If all the linear dimensions are doubled keeping temperatures constant, then rate of flow of heat Q2 will be :
View SolutionHeating of the ends of a cylindrical rod T_(1) and T_(2) Huh. Heat flow rate Q_(1)cal//s is. If all the linear dimensions of the rod are doubled and the temperature is fixed, then the rate of heat flow Q_(2) Will be
View SolutionA heat engine absorbs heat q1 from a source at temperature T1 and heat q2 from a source at temperature T2. Work done is found to be J(q1+q2). This is in accordance with
View SolutionA cylindrical rod having temperature T_(1) and T_(2) at its end. The rate of flow of heat is Q_(1)("Cal")/(s) If all linear dimensions are doubled keeping temperature constant, then the rate of flow of heat Q_(2) in ("Cal")/(s) will be . . . . . . . .
View SolutionA cylindrical rod having temperature T1 and T2 at its end. The rate of flow of heat is Q1Cals If all linear dimensions are doubled keeping temperature constant, then the rate of flow of heat Q2 in Cals will be . . . . . . . .
View Solution
