Question

The area bounded by the curve $x=a{\cos }^{3}t,,y=a{\sin }^{3}t,$ is :

Options

A. `12a^(2)overset(pi//2)underset(0)intcos^(4)t sin^(2) t dt`
B. `12a^(2)overset(pi//2)underset(0)intcos^(2)t sin^(4) t dt`
C. `2overset(a)underset(-a)int(a^(2//3)-x^(2//3))^(3//2)dx`
D. `4overset(a)underset(0)int(a^(2//3)-x^(2//3))dx`

Answer

A::C::D

Explanation

`"Eliminating t, we have "x^(2/(3))+y^((2)/(3))=a^((2)/(3))rArry=(a^(2//3)-x^(2//3))^(3//2).`

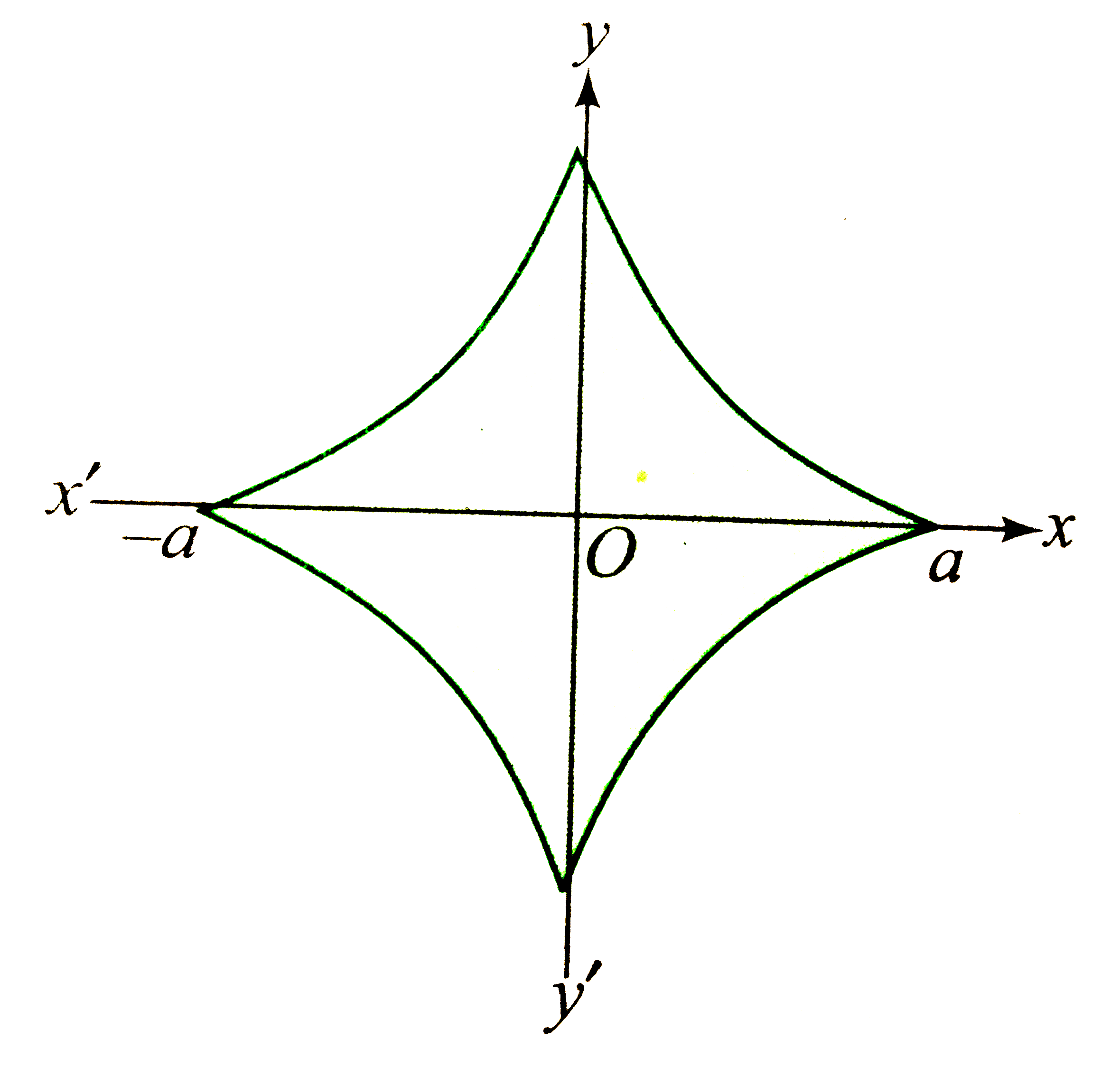
From the figure,
`A=2overset(a)underset(-a)int(a^(2//3)-x^(2//3))dx`
`=4overset(a)underset(0)int(a^(2//3)-x^(2//3))^(3//2)dx=4overset(a)underset(0)inty dx`
`=4a^(2)overset(pi//2)underset(0)int3 cos^(3) t sin^(2) t cos t dt.`