Question

Find the two whole numbers such that their sum is utmost 10 and the difference is atleast 4 and also the resultant sum is maximum

Explanation

Let the two whole numbers be x and y
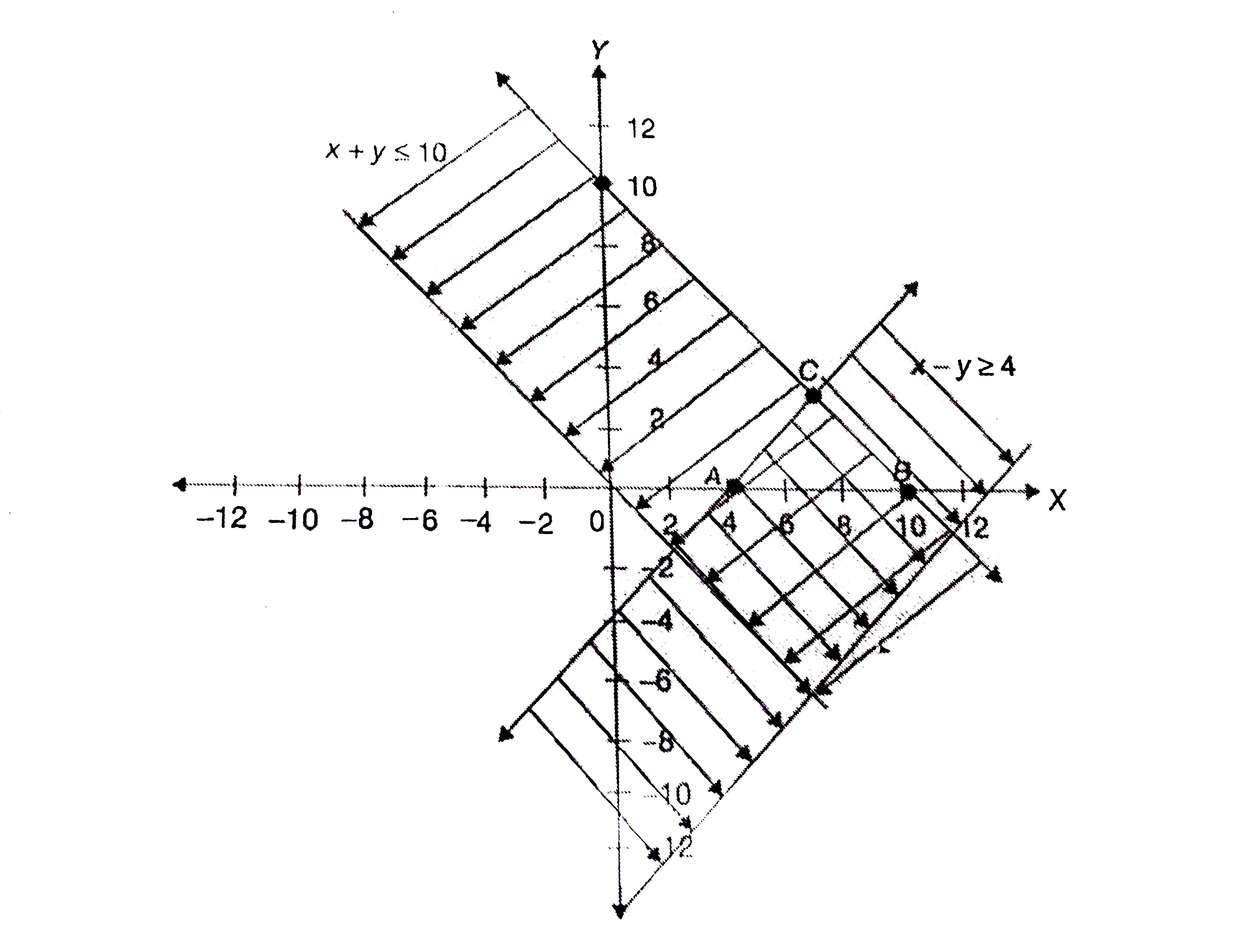
The required inequations subject to the given conditions are
`x+y lt 10` (1)
`x -y ge 4` (2)
`x ge 0, y ge 0` (3)
Tracing the solution set of the above four inequations, clearly the solution set is the triangular region ABC. For the sum of the numbers to be maximum, the solution ocuurs at the vertices of the region ABC.
The vertices are (4,0), (10,0) and (7,3). Out of these (10,0) and (7,3) give the maximum value for `x +y`
`:.` The solution are (10,0), (7,3)