A bullet of mass 50 g is fired from below into a suspended object of mass 450 g. The object rises through a height of 1.8 m with bullet remaining inside the object. Find the speed of the bullet. Take g=10ms−2Solution in Tamil
Step by step video & image solution for A bullet of mass 50 g is fired from below into a suspended object of mass 450 g. The object rises through a height of 1.8 m with bullet remaining inside the object. Find the speed of the bullet. Take g=10ms_(-2) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER 15 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER 15 (UNSOLVED)EXERCISE - Part-IV
10 Videos
- SAMPLE PAPER 15 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER 15 (UNSOLVED)EXERCISE - Part -II
9 Videos
- SAMPLE PAPER 13 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER 13 (UNSOLVED)EXERCISE - Part -IV
10 Videos
- SAMPLE PAPER-14 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER-14 (UNSOLVED)EXERCISE - Part-IV
10 Videos
Similar Questions
What do you mean by propagation of errors? Explain the propagation of errors in addition and multiplication.
View SolutionDefine - Astronomical unit?
View Solution(i) Explain the use of screw gauge and vernier caliper in measuring smaller distances.
(ii) Write a note on triangultion method and radar method to measure larger distances.
View SolutionConvert 76cm of mercury pressure into Nm−2 using the method of dimensions.
View SolutionA bullet is fired horizontally aiming at an object which starts falling at the instant the bullet is fired. Show that the bullet will hit the object.
View SolutionA bullet of mass 50 g is fired rom below into the bob of mass 450 g of a long simple pendulum as hown in figure. The bullet premains inside the bob and the bob rises thrugh a height of 1.8 m. Find the speed of the bullet. Take g=10ms2
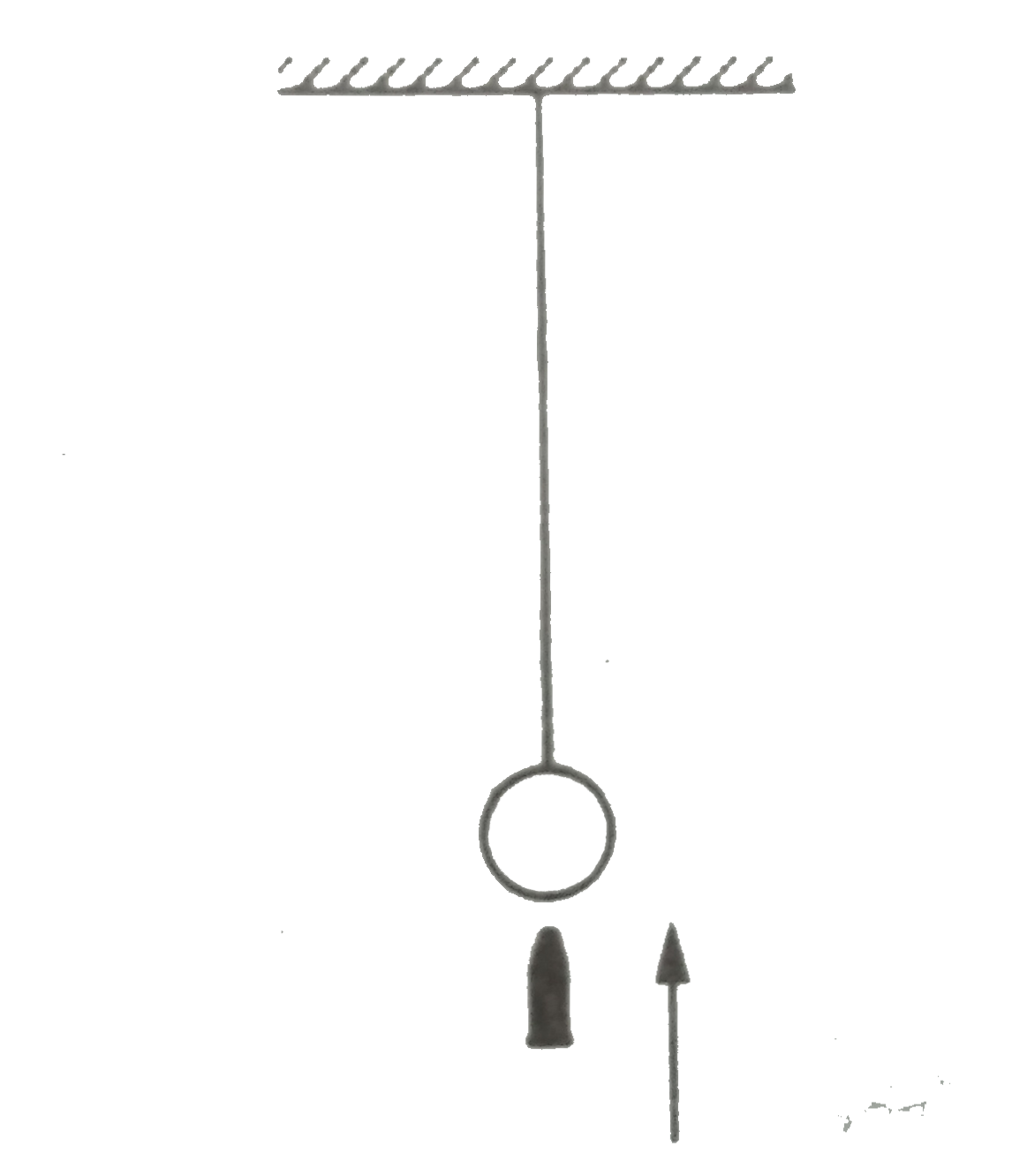
View SolutionA bullet of mass 50 g is fired rom below into the bob of mass 450 g of a long simple pendulum as hown in figure. The bullet premains inside the bob and the bob rises thrugh a height of 1.8 m. Find the speed of the bullet. Take g=10ms2

View Solutionएक लम्बे सरल दोलक में बढे का द्रव्यमान 450 g है निचे से इस बाब पर 50 g की एक गोली दागी जाती है गोली इस बाब के अंदर ही रह जाती है और बाब 1.8m उचाई तक तह जाता है g=10m/s2 लेते हुए गोली का प्रारंभिक वेग बताइये
View Solutionभौतिकी की सम् अतः, संवेग-संरक्षण के सिद्धांत से, एक लंबे सरल दोलक में बँधे बॉब का द्रव्यमान 450 g है। नीचे से इस बॉब पर 50 g की एक गोली दागी जाती है। गोली इस बाब के अंदर ही रह जाती है और बॉब 1.8 m ऊँचाई तक उठ जाता है। g=10m/s2 लेते हुए गोली का प्रारंभिक वेग बताएँ।
View Solutionयदि एक बंदूक से 200 ग्राम द्रव्यमान की एक गोली 50 मीटर/सेकण्ड वेग से दागी जाती है , तो गोली की गतिज ऊर्जा ज्ञात कीजिए।
View SolutionA bullet of mass 50 g is fired from below into a suspended object of mass 450 g. The object rises through a height of 1.8 m with bullet remaining inside the object. Find the speed of the bullet. Take g=10ms−2
View SolutionA bullet of mass 50 g is fired from below into a suspended object of mass 450 g. The object rises through a height of 1.8 m with bullet remaining inside the object. Find the speed of the bullet. Take g=10ms−2
View SolutionA bullet moving with a speed of 200 m s−1 is absorbed by a wooden block of mass 1500 g suspended by a string . Caclulate the vertical height through which the block will rise . The mass of the bullet is 20 g .
View Solution(अ)5 किग्रा. की एक वस्तु को 4 मीटर ऊँचाई पर ले जाने में किये गए कार्य की गणना कीजिए। (g = 10 मीटर/सेकण्ड2)
(ब) यदि एक बंदूक से 200 ग्राम द्रव्यमान की एक गोली 50 मीटर/सेकण्ड वेग से दागी जाती है, तो गोली की गतिज ऊर्जा ज्ञात कीजिए।
View SolutionA bullet is fired horizontally aiming at an object which starts falling at the instant the bullet is fired. Show that the bullet will hit the object.
View SolutionA bullet of mass 50g if fired from below into the bob of mass 450g of a long simple pendulum as shown in Fig. The bullet remains inside the bob and the bob rises through a height of 1.8m. Find the speed of the bullet.Take g=10ms2

View Solution
