A bullet of mass 50 g is fired rom below into the bob of mass 450 g of a long simple pendulum as hown in figure. The bullet premains inside the bob and the bob rises thrugh a height of 1.8 m. Find the speed of the bullet. Take $g = 10 \frac{m}{s^{2}}$

Video Solution

Text Solution

Verified by Experts

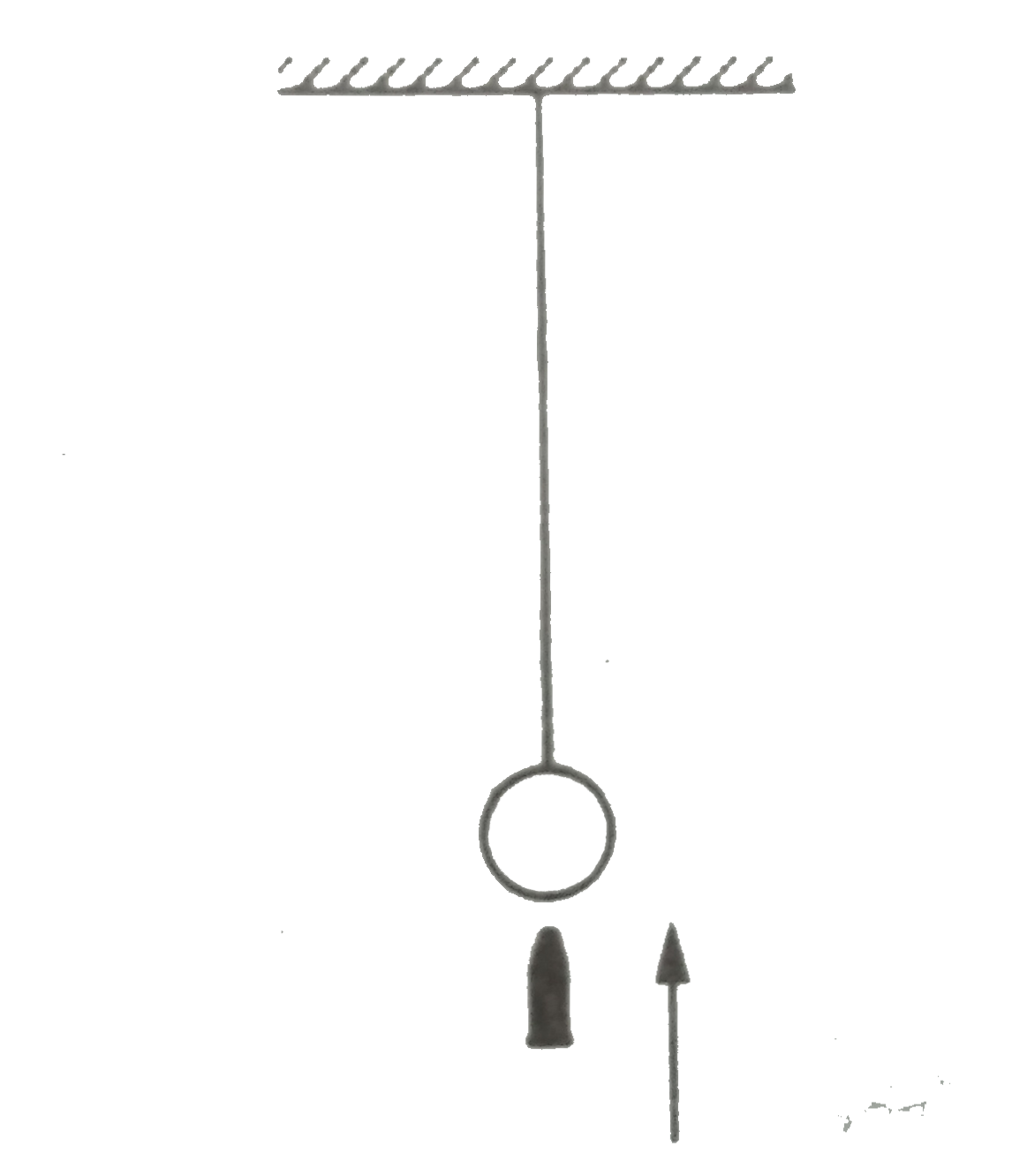
Let the speed of the bullet b v. Let the common velocity of the bullet and the bob, after the bullet is embedded ito the bob, is V. By the principle of conservation of linear momentum,
$V = \frac{(0.05 k g) v}{0.45 k g + 0.05 k g} = \frac{v}{10}$
The string becomes loose and the bob will go up with a deceleration of $g = 10 \frac{m}{s^{2}}$ . As it comes to rest at a height of 1.8 m, usn the equation $v^{2} = u^{2} + 2 a x, < b r >$ 1.8m=((v/10)^2)/(2xx10m/s^2) $< b r > or$ v=60 m/s

Updated on:21/07/2023

A bullet of mass 10 g is fired from a gun of mass 1 kg with recoil velocity of gun 5 m/s. The muzzle velocity will be
A30 km/min
B60 km/min
C30 m/s
D500 m/s
A bullet of mass 10 g is fired with a velocity of 100 m/s from a rifle of mass 2.5 kg, the recoil velocity of the rifle is
A $- 0.4 m / s$
B $+ 0.4 m / s$
C $- 4 m / s$
D $+ 4 m / s$
A bullet of mass 40 g is fired from a gun of mass 10 kg. If velocity of bullet is 400 m/s. then the recoil velocity of the gun wil be
A $1 \cdot 6$ m/s in the direction of bullet
B $1 \cdot 6$ m/s opposite to the direction of bullet
C $1 \cdot 8$ m/s in the direction of bullet
D $1 \cdot 8$ m/s opposite to the direction of bullet