Question

Define orbital velocity and establish an expression for it.

Explanation

(i) Orbital velocity is the velocity given to artificial satellite so that it may start revoling around the earth.
Expression for orbital velocity
Consider a satellite of mass .m. is revolving around the earth in a circular orbit of radius .r. at a height .h. from the surface of the earth.
Let .M. be the mass of the earth and .R. be the radius of the earth
Therefore , r = R + h
The centripetal force that is required to revolve the satellite ` = (mv_0^2)/(r )`
where `V_0` is orbital velocity
Orbital velocity is produced by the gravitational force b/w the earth and the satellite ` = (GMm)/(r^2)`

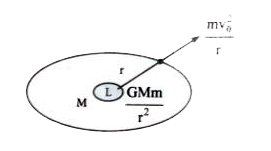
` therefore (mv_2^2)/(r ) = (GMm)/(r^2)`
` v_0^2 = (GM)/( r) = (GM)/( R + h)`
` v_0 = sqrt((GM)/(r )) = sqrt((GM)/(R +h))`
This is the expression for the orbital velocity.
(ii) Mass of the earth `M = 6 xx 10^24 kg `
Radius of the earth R = 64000 km
The height of the artificial satellite from the earth (h) = 1000 km
Gravitational constant ` (G) = 6.67 xx 10^(-11) Nm^(-2) kg^(-2)`
Orbital velocity ` V_0 = sqrt((GM)/(R+h))`
` = sqrt( (6.7 xx 10^(-11) xx 6 xx 10^24)/(64000+1000)) = sqrt( (40.02 xx 10^13)/(65 xx 10^3)) = sqrt( (400.2)/(65)) = sqrt(6.1569)`
` V_0 = 2.48 ms^(-1)`