A battery has an emf of 12 V and connected to a resistor of 3Ω. The current in the circuit is 3.93 A. Calculate (a) terminal voltage and the internal resistance of the battery (b) power delivered by the battery and power delivered to the resistor. Solution in Tamil
The given values I=3.93A,ξ=12V,R=3Ω
(a) The terminal voltage of the battery is equal to voltage drop across the resistor
V=IR=3.93×3=11.79V
The internal resistance of the battery,
r=[ξ−VV]R=[12−11.7911.79]×3=0.05Ω
(b) The power delivered by the battery
P=Iξ=3.93×12=47.1W
The power delivered to the resistor =I2R=46.3W
The remaining power =(47.1−46.3)P=0.772W is delivered to the internal resistance and cannot be used to do useful work. (it is equal to I2r).
Topper's Solved these Questions
- BOOK - FULL MARKSCHAPTER - CURRENT ELECTRICITYEXERCISE - TEXTBOOK EVALUATION (Choose the correct answer)15videos
CURRENT ELECTRICITY
- BOOK - FULL MARKSCHAPTER - CURRENT ELECTRICITYEXERCISE - TEXTBOOK EVALUATION (Short Answer Questions)21videos
CURRENT ELECTRICITY
- BOOK - FULL MARKSCHAPTER - COMMUNICATION SYSTEMSEXERCISE - ADDITIONAL QUESTIONS (Additional problems)3videos
COMMUNICATION SYSTEMS
- BOOK - FULL MARKSCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - ADDITIONAL QUESTIONS -( ADDITIONAL NUMERICAL PROBLEMS : )10videos
DUAL NATURE OF RADIATION AND MATTER
Similar Questions
The following graph shows current versus voltage values of some unknown conductor. What is the resistance of this conductor?
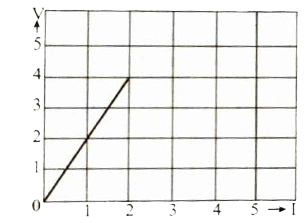 02:41View Solution
02:41View SolutionWhy current is a scalar?
02:18View SolutionA radio can tune into any station in the 7.5 MHz to 12 MHz band. What is the corresponding wavelength band ?
01:34View SolutionIf a light of wavelength 4950 Å is viewed as a continuous flow of photons, what is the energy of each photon in eV ? (Given h=6.6×10−34Js, c =3×108ms−1)
05:50View SolutionA battery of emf 15 V and internal resistance of 4Omega is connected to a resistor. If the current in the circuit is 2A and the circuit is closed. Resistance of the resistor and terminal voltage of the battery will be
View SolutionA battery having 12V emf and internal resistance 3Ω is connected to a resistor. If the current in the circuit is 1A, then the resistance of resistor and lost voltage of the battery when circuit is closed will be
03:59View SolutionA battery of emf 10 V and internal resistance 3Ωis connected to a resistor. The current in the circuit is 0.5 A . The terminal voltage of the battery when the circuit is closed is
03:27View Solution10 V विधुत-वाहक बल (emf) तथा 3Ω आंतरिक प्रतिरोधवाली बैटरी को किसी प्रतिरोधक (resistor) से जोड़कर बंद परिपथ (closed circuit) से 0.5A की विधुत-धारा प्रवाहित होती है। प्रतिरोधक का प्रतिरोध (resistance of the resitstor ) तथा बैटरी की टर्मिनल वोल्टता (ternimal voltage) ज्ञात करें जब परिपथ बंद हो।
03:10View SolutionThe power delivered by a battery of emf, 10 is 10W. Find the current delivered by the battery.
01:06View SolutionA battery of emf 10V and internal resistance 3Ω is connected to a resistor. If the current in the circuit is 0.5A, what is the resistance of the resistor? What is the terminal voltage of the battery when the circuit is closed?
03:12View SolutionA battery of 3.0 V is connected to a resistor dissipating 0.5 W of power . If the terminal voltage of the battery is 2.5 V , the power dissipated within the internal resistance is :
03:22View SolutionA battery of emf 10 V and internal resistance 3 ohm is connected to a resistor. The current in the circuit is 0.5 A. The terminal voltage of the battery when the circuit is closed is
02:38View SolutionA battery of emf 10 V and internal resistance 3Omega is connected to a resistor. The current in the circuit is 0.5A. The terminal voltage of the battery when the circuit is closed is
02:38View Solution12 V वि.वा.बल एवं आंतरिक प्रतिरोध 3Ω वाली एक बैटरी को किसी प्रतिरोधक से जोड़ा जाता है। यदि परिपथ में धारा 1A हो, तो प्रतिरोधक का प्रतिरोध एवं बैटरी का हास वोल्टता क्या होगी जब परिपथ बन्द हो?
View SolutionA battery of 3.0 V is connected to a resistor dissipating 0.5 W of power . If the terminal voltage of the battery is 2.5 V , the power dissipated within the internal resistance is :
04:01View Solution

