Suppose there is vertically upwards magnetic field. How will the particle deviate according 1. If positive charge is moving horizontally, in front of you, away from you? 2. If negative charge is moving horizontally, in front of you, towards you? 3. If positive charge is moving horizontally, in front of you, towards you? 4. If negative charge is moving horizontally, in front of you, away from you?
To solve the problem, we will analyze the deviation of charged particles in a vertically upward magnetic field using the right-hand rule and the Lorentz force equation F=q(v×B).
1. Understanding the Setup:
- We have a magnetic field directed vertically upwards.
- We will analyze the motion of charged particles (positive and negative) moving horizontally in two directions: towards the observer and away from the observer.
2. Case 1: Positive Charge Moving Horizontally Away from the Observer:
- Direction of Velocity (v): Away from the observer (let's assume this is to the right).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers in the direction of v (to the right) and curl them upwards (in the direction of B). Your thumb will point out of the page, indicating the force direction.
- Result: The particle will deviate out of the page (towards you).
3. Case 2: Negative Charge Moving Horizontally Towards the Observer:
- Direction of Velocity (v): Towards the observer (let's assume this is to the left).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers to the left (direction of v) and curl them upwards. Your thumb will point out of the page.
- Result: Since the charge is negative, the force will be in the opposite direction of your thumb, which means it will deviate into the page (away from you).
4. Case 3: Positive Charge Moving Horizontally Towards the Observer:
- Direction of Velocity (v): Towards the observer (to the left).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers to the left (direction of v) and curl them upwards. Your thumb will point out of the page.
- Result: The particle will deviate out of the page (towards you).
5. Case 4: Negative Charge Moving Horizontally Away from the Observer:
- Direction of Velocity (v): Away from the observer (to the right).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers to the right (direction of v) and curl them upwards. Your thumb will point out of the page.
- Result: Since the charge is negative, the force will be in the opposite direction of your thumb, which means it will deviate into the page (away from you).
Summary of Deviations:
1. Positive charge moving away: Deviates towards you (out of the page).
2. Negative charge moving towards you: Deviates away from you (into the page).
3. Positive charge moving towards you: Deviates towards you (out of the page).
4. Negative charge moving away: Deviates away from you (into the page).
- We have a magnetic field directed vertically upwards.
- We will analyze the motion of charged particles (positive and negative) moving horizontally in two directions: towards the observer and away from the observer.
- Direction of Velocity (v): Away from the observer (let's assume this is to the right).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers in the direction of v (to the right) and curl them upwards (in the direction of B). Your thumb will point out of the page, indicating the force direction.
- Result: The particle will deviate out of the page (towards you).
- Direction of Velocity (v): Towards the observer (let's assume this is to the left).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers to the left (direction of v) and curl them upwards. Your thumb will point out of the page.
- Result: Since the charge is negative, the force will be in the opposite direction of your thumb, which means it will deviate into the page (away from you).
- Direction of Velocity (v): Towards the observer (to the left).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers to the left (direction of v) and curl them upwards. Your thumb will point out of the page.
- Result: The particle will deviate out of the page (towards you).
- Direction of Velocity (v): Away from the observer (to the right).
- Direction of Magnetic Field (B): Vertically upwards.
- Using the Right-Hand Rule: Point your fingers to the right (direction of v) and curl them upwards. Your thumb will point out of the page.
- Result: Since the charge is negative, the force will be in the opposite direction of your thumb, which means it will deviate into the page (away from you).
1. Positive charge moving away: Deviates towards you (out of the page).
2. Negative charge moving towards you: Deviates away from you (into the page).
3. Positive charge moving towards you: Deviates towards you (out of the page).
4. Negative charge moving away: Deviates away from you (into the page).
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Tough & Tricky (PROBLEMS )
15 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - NCERT FILE (NCERT Textbook Exercises)
13 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - PRACTICE PROBLEMS
59 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
Similar Questions
Knowledge Check
Whe a negative charge is releases and moves in electric field , it moves toward a position of -
Alower electric potential and lower potential energyBlower electric potential energyChigher electric potential and lower potential energyDhigher electric potential and higher potential energyA proton moves horizontally towards a vertical conductor with a uniformly distributed positive charge. It will, undergo
Ahorizontal deflectionBvertical deflectionCno deflectionDretardationA charge +Q is moving upwards vertically. It enters a magnetic field directed to the north. The force on the charge will be towards
ANorthBSouthCEastDWest
If the magnetic field is parallel to the positive y-axis and the charged particle is moving along the positive x-axis (Fig.), which way would the Lorentz force be for (a) an electron (negative charge), (b) a proton (positive charge).
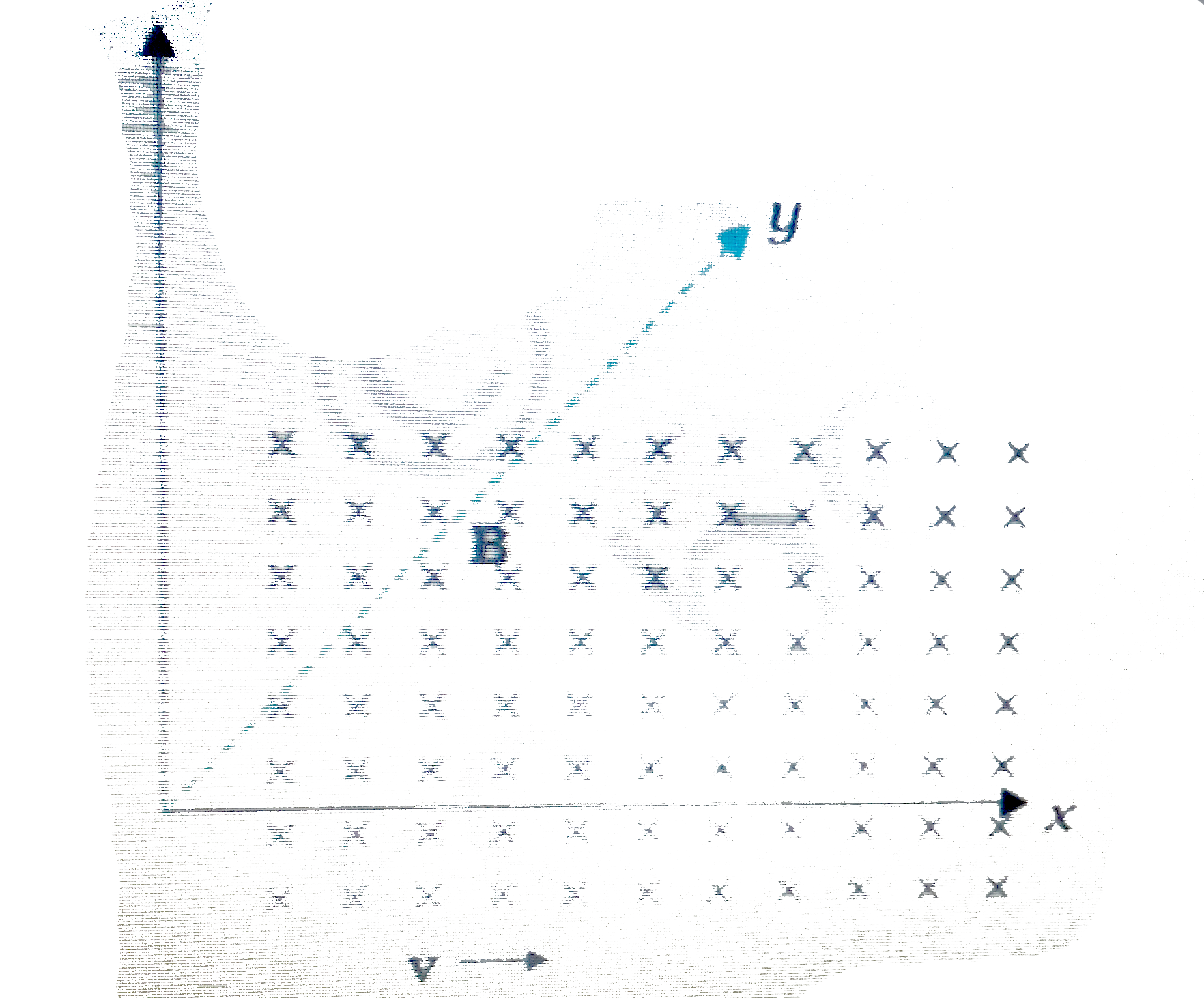
View SolutionA beam of protons is moving horizontally towards you. As it approaches, it passes through a magnetic field directed downward. The beam deflects-
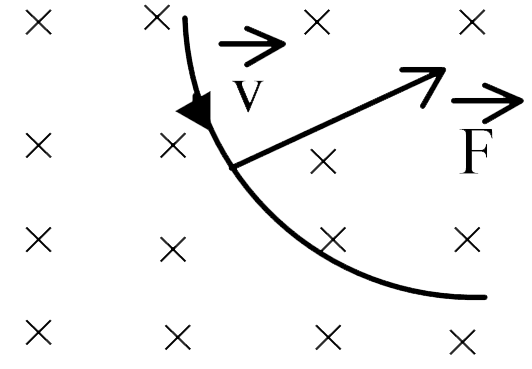
View SolutionIf a positively charged particle is moving as shown in the figure, then it will get deflected due to magnetic field towards

View SolutionA positive charge of 20μC is placed 2 m away from a negative charge of 20μC. Determine the electric field at a point 2 m away from each charges.
View SolutionA positive charge of 20μC is placed 2 m away from a negative charge of 20μC. Determine the electric field at a point 2 m away from each charges.
View Solution
