Electron, proton, He+ and Li+ are projected with the same speed perpendicular to a uniform magnetic field. Which particle will experience the maximum magnetic force?
To determine which particle experiences the maximum magnetic force when projected with the same speed perpendicular to a uniform magnetic field, we can use the formula for magnetic force:
F=q⋅v⋅B⋅sin(θ)
Where:
- F is the magnetic force,
- q is the charge of the particle,
- v is the speed of the particle,
- B is the magnetic field strength,
- θ is the angle between the velocity and the magnetic field (which is 90 degrees in this case, making sin(90∘)=1).
Since all particles are projected with the same speed v and are in the same magnetic field B, the magnetic force depends solely on the charge q of each particle.
1. Identify the charges of the particles:
- Electron (e−): Charge q=−1.6×10−19C
- Proton (p): Charge q=+1.6×10−19C
- Helium ion (He+): Charge q=+2×1.6×10−19C=+3.2×10−19C
- Lithium ion (Li+): Charge q=+3×1.6×10−19C=+4.8×10−19C
2. Calculate the magnetic force for each particle:
- For the electron:
Fe=|q|⋅v⋅B=(1.6×10−19)⋅v⋅B
- For the proton:
Fp=|q|⋅v⋅B=(1.6×10−19)⋅v⋅B
- For the helium ion:
FHe=|q|⋅v⋅B=(3.2×10−19)⋅v⋅B
- For the lithium ion:
FLi=|q|⋅v⋅B=(4.8×10−19)⋅v⋅B
3. Compare the magnitudes of the forces:
- The electron and proton have the same charge magnitude, so their forces will be equal.
- The helium ion has a charge of +3.2×10−19C, resulting in a greater force than the electron and proton.
- The lithium ion has the highest charge of +4.8×10−19C, resulting in the maximum force.
4. Conclusion:
The particle that experiences the maximum magnetic force is the lithium ion (Li+).
- F is the magnetic force,
- q is the charge of the particle,
- v is the speed of the particle,
- B is the magnetic field strength,
- θ is the angle between the velocity and the magnetic field (which is 90 degrees in this case, making sin(90∘)=1).
- Electron (e−): Charge q=−1.6×10−19C
- Proton (p): Charge q=+1.6×10−19C
- Helium ion (He+): Charge q=+2×1.6×10−19C=+3.2×10−19C
- Lithium ion (Li+): Charge q=+3×1.6×10−19C=+4.8×10−19C
- For the electron:
Fe=|q|⋅v⋅B=(1.6×10−19)⋅v⋅B
- For the proton:
Fp=|q|⋅v⋅B=(1.6×10−19)⋅v⋅B
- For the helium ion:
FHe=|q|⋅v⋅B=(3.2×10−19)⋅v⋅B
- For the lithium ion:
FLi=|q|⋅v⋅B=(4.8×10−19)⋅v⋅B
- The electron and proton have the same charge magnitude, so their forces will be equal.
- The helium ion has a charge of +3.2×10−19C, resulting in a greater force than the electron and proton.
- The lithium ion has the highest charge of +4.8×10−19C, resulting in the maximum force.
The particle that experiences the maximum magnetic force is the lithium ion (Li+).
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Tough & Tricky (PROBLEMS )
15 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - NCERT FILE (NCERT Textbook Exercises)
13 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - PRACTICE PROBLEMS
59 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
Similar Questions
Knowledge Check
Particles 1, 2 and 3 are moving perpendicular to a uniform magnetic field, then particle
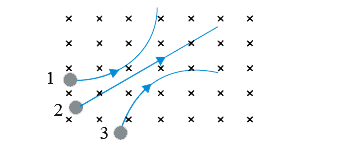 A1 is positively charged and particle 3 is negatively chargedB1 is negatively charged and particle 3 is positively chargedC1 is negatively charged and particle 2 is neutralD1 and 3 are positively charged and particle 2 is neutral
A1 is positively charged and particle 3 is negatively chargedB1 is negatively charged and particle 3 is positively chargedC1 is negatively charged and particle 2 is neutralD1 and 3 are positively charged and particle 2 is neutralAn electron and a proton with equal momentum enter perpendicularly into a uniform magnetic field, then
AThe path of proton shall be more curved than that of electronBThe path of proton shall be less curved than that of electronCBoth are equally curvedDPath of both will be straight linePath of an electron entering perpendicular in a uniform magnetic field will be:
ALineasrBparaobolicCellipseDcircular
An electron and a proton are projected with same velocity perpendicular to a magnetic field.
(a) Which particle will describe the smaller circle?
(b) Which particle will have greater frequency?
View SolutionA particle is projected in a plane perpendicular to a uniform magnetic field. The area bounded by the path described by the particle is proportional to
View SolutionA proton is projected with a velocity of 3X106ms−1 perpendicular to a uniform magnetic field of 0.6 T. find the acceleration of the proton.
View SolutionIf a charged particle enters perpendicular in the uniform magnetic field then
View SolutionFour charged particles electron, proton, He+ and Li+ are projected perpendicularly into the magnetic field with equal speed. Which will have minimum frequency?
View Solution
