Let d be the perpendicular distance from the centre of the ellipse x2a2+y2b2=1 to the tangent drawn at a point P on the ellipse. If F1&F2 are the two foci of the ellipse, then show the (PF1−PF2)2=4a2[1−b2d2].
Let the coordinates of point P be (acosθ,bsinθ).
then , equation of tangent at P is
xacosθ+ybsinθ=1
we have ,d= length fo perpendicular from O to the tangent at P ,
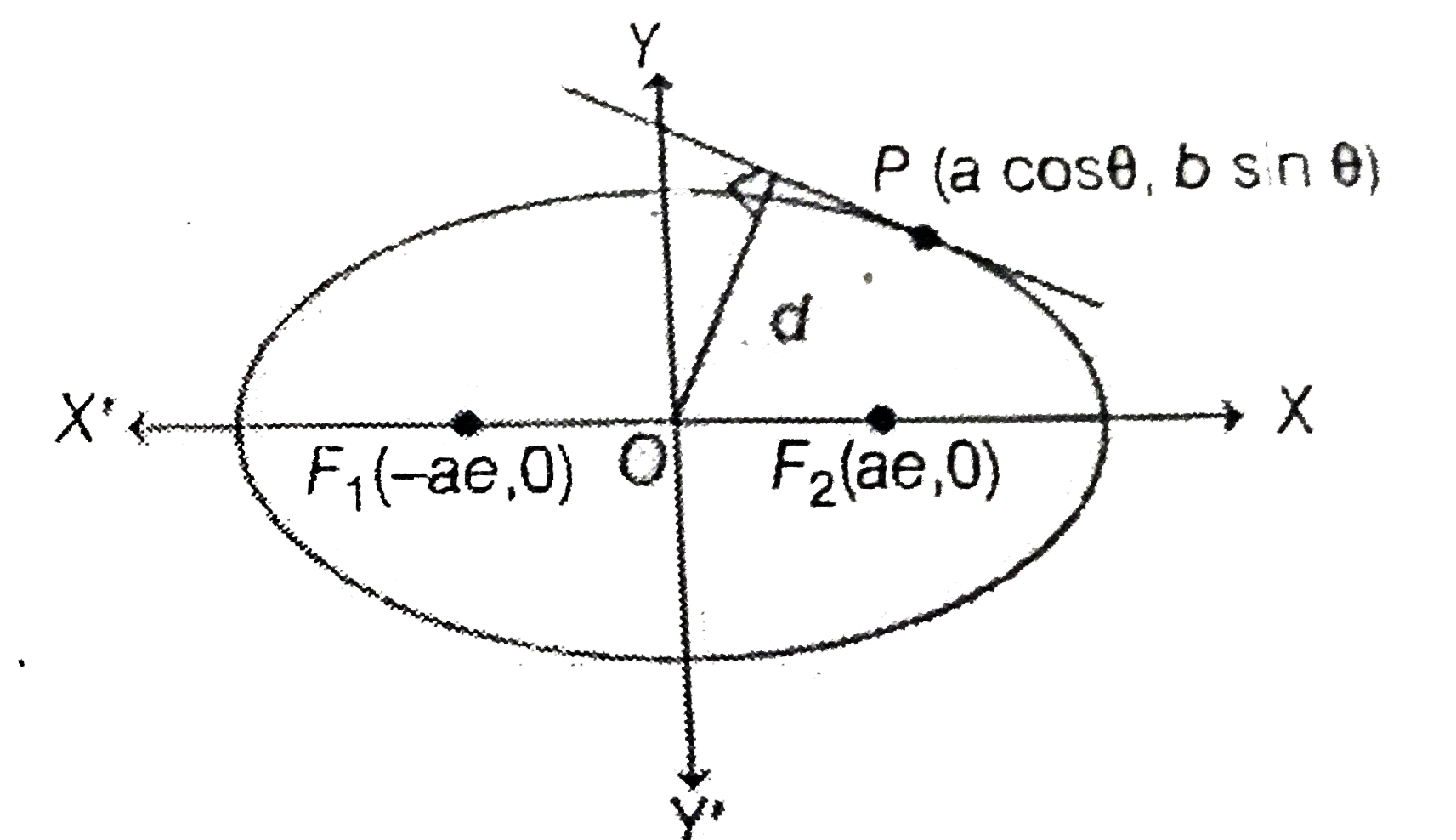
d=|0+0−1|√cos2θa2+sin2θb2
1d=√cos2θa2+sin2θb2
⇒1d2=cos2θa2+sin2θb2
we have to prove (PF1−PF2)2=4a2(1−b2d2)
Now , RHS=4a2(1−b2d2)=4a2−4a2b2d2
=4a2−4a2b2(cos2θa2+sin2θb2)
=4a2−4b2cos2θ−4a2sin2θ
=4a2(1−sin2θ)−4b2cosθ
=4a2cos2θ−4b2cos2θ−4b2cos2θ
=4cos2θ(a2−b2)=4cos2θ.a2e2[∵e=√2−(b/a)2
Again PF1=e|acosθ+a/ee|=a|ecosθθ+1|
=a(ecosθ+1)
[∵−1≤cosθ1and0<e<1]
Similarly , PF2=a(1−ecosθ)
Therefore,LHS=(PF1−PF2)2
=[a(ecosθ+1)−a(1−ecosθ)]2
=(aecosθ+α−a+aecosθ)2
=(2aecosθ)2=4a2e2cos2θ
hence LHS=RHS
Topper's Solved these Questions
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - ELLIPSEEXERCISE - EQUATION OF CHORD OF CONTACT, CHORD BISECTED AT A GIVEN POINT & DIAMETER3videos
ELLIPSE
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - ELLIPSEEXERCISE - EQUATION OF CHORD OF CONTACT, CHORD BISECTED AT A GIVEN POINT & DIAMETER3videos
ELLIPSE
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - DIFFERENTIAL EQUATIONS EXERCISE - TOPIC 3 APPLICATIONS OF HOMOGENEOUS DIFFERENTIAL EQUATIONS <br> ANALYTICAL & DESCRIPTIVE QUESTIONS7videos
DIFFERENTIAL EQUATIONS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - FUNCTIONSEXERCISE - INVERSE AND PERIODIC FUNCTIONS11videos
FUNCTIONS
Similar Questions
Let 'p' be the perpendicular distance from the centre C of the hyperbola x2a2−y2b2=1 to the tangent drawn at a point R on the hyperbola. If S&S' are the two foci of the hyperbola, then show that (RS+RS')2=4a2(1+b2p2).
View SolutionThe product of the perpendiculars from the two foci of the ellipse x29+y225=1 on the tangent at any point on the ellipse
View Solution
Knowledge Check
- Question 1 - Select One
The product of the perpendiculars from the foci of the ellipse x2144+y2100=1 on any tangent is:
A144B100C122D200 - Question 2 - Select One
If p is the length of the perpendicular from the focus S of the ellipse x2a2+y2b2=1 to a tangent at a point P on the ellipse, then 2aSP−1=
Aa2p2Bb2p2Cp2Da2+b2p2 - Question 3 - Select One
The locus of foot of perpendicular from focus of ellipse x2a2+y2b2=1 to its tangents is
Ax2+y2=a2+b2Bx2+y2=a2Cx2+y2=(a+b)2Dnone of these
The locus of the feet of perpendiculars drawn from the centre of the ellipse x2a2+y2b2=1 on any tangent to it is .......... .
View SolutionLet d1 and d2 be the lengths of perpendiculars drawn from foci S' and S of the ellipse x2a2+y2b2=1 to the tangent at any point P to the ellipse. Then S'P:SP is equal to
View SolutionNumber of points on the ellipse x2a2+y2b2=1 at which the normal to the ellipse passes through at least one of the foci of the ellipse is
View SolutionLet P be a point on the ellipse x2100+y225=1 and the length of perpendicular from centre of the ellipse to the tangent to ellipse at P be 5√2andF1andF2 be the foci of the ellipse, then PF1.PF2.
View SolutionIf M1 and M2 are the feet of perpendiculars from foci F1 and F2 of the ellipse x264+y225=1 on the tangent at any point P of the ellipse then
View Solution

