Question

9 Prove that the values of the function $\frac{\sin x\cos 3x}{\sin 3x\cos x}$ cannot lie between $\frac{1}{3}$ and $3$ for any real $x$

Explanation

Let `y=(sinxcos3x)/(sin3x cos x)=(tanx)/(tan 3x)`
`implies y = (tanx)/(tan3x)=(tanx(1-3tan^(2)x))/(3tanx-tan^(3)x)`
`=(1-3tan^(2)x)/(3-tan^(2)x)" "[because x ne0]`
Put tan x = t
`implies y = (1-3t^(2))/(3-t^(2))`
`implies 3y-t^(2)y = 1-3t^(2)`
`implies 3y-1=t^(2)y-3t^(2)`
`implies 3y-1=t^(2)(y-3)`

`implies (3y-1)/(y-3)=t^(2)implies(3y-1)/(y-3)gt0`
`therefore t^(2) gt 0`
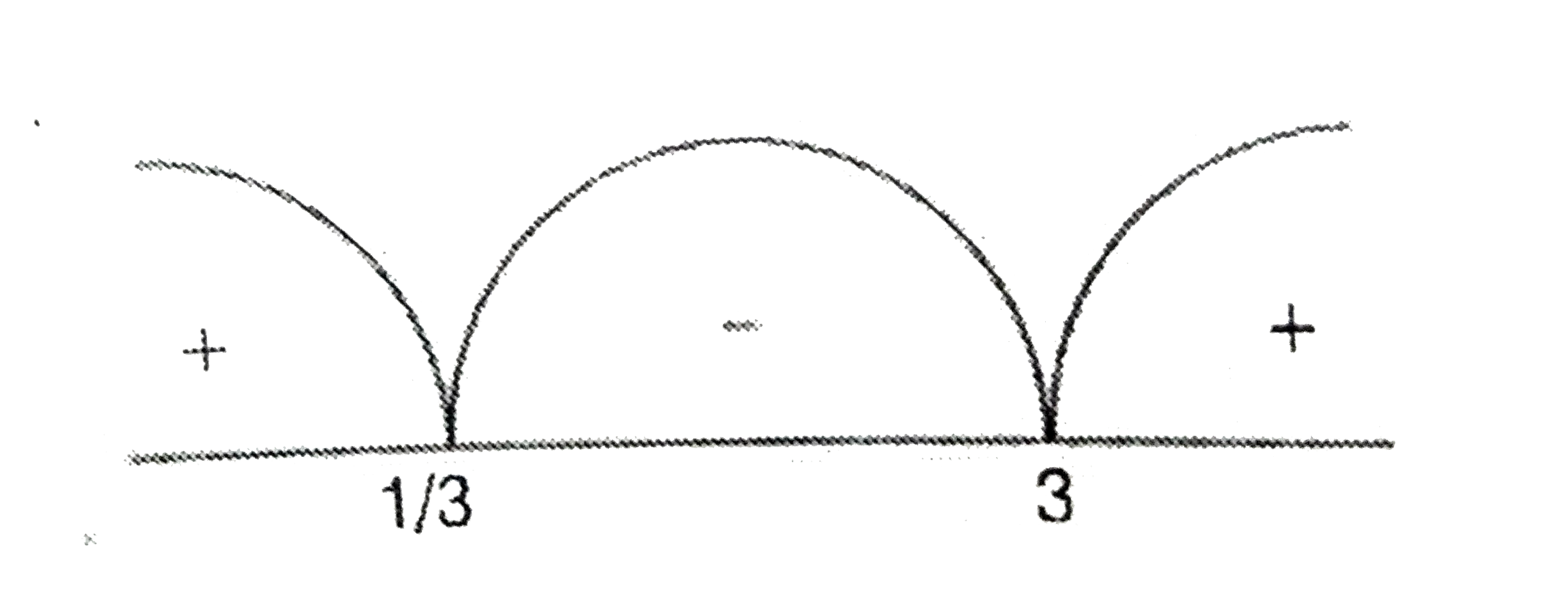
NOTE It is a brilliant technique to convert equation into inequation and asked in IIT papers frequently. `impliesylt1//3orygt3`. This shows that y cannot lie between `1//3` and 3.