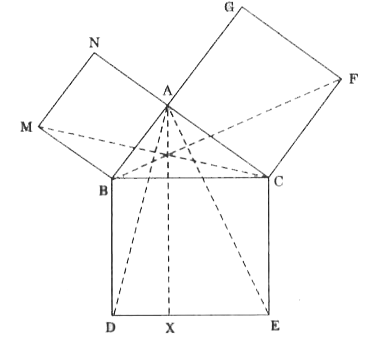
లంబకోణ త్రిభుజము ABC లో A లంబకోణం. BC,CA మరియు AB లపై వరుసగా BCED, ACFG మరియు ABMN అనే చతురస్రాలు గీయబడ్డాయి, రేఖాఖండం AX _|_DE, BC ని Y వద్ద, DE ని X వద్ద ఖండించింది. AD, AE లు కలుపబడ్డాయి. అదే విధంగా BF, CM లు కలుపబడ్డాయి. (పటంలో చుడండి). అయితే కింది వానిని నిరూపించండి. △FCB≅△ACE
Step by step video & image solution for లంబకోణ త్రిభుజము ABC లో A లంబకోణం. BC,CA మరియు AB లపై వరుసగా BCED, ACFG మరియు ABMN అనే చతురస్రాలు గీయబడ్డాయి, రేఖాఖండం AX _|_DE, BC ని Y వద్ద, DE ని X వద్ద ఖండించింది. AD, AE లు కలుపబడ్డాయి. అదే విధంగా BF, CM లు కలుపబడ్డాయి. (పటంలో చుడండి). అయితే కింది వానిని నిరూపించండి. /_\FCB~=/_\ACE by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
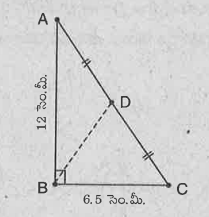
△ABC లో ∠ABC=90∘, AD=DC, AB=12 సెం.మీ. మరియు BC=6.5 సెం.మీ. అయిన △ADB వైశాల్యము కనుగొనండి.
View Solutionపటంలో O వృత్త కేంద్రం. ఆయన దిగువ ఇవ్వబడిన భాగాల పేర్లు తెలపండి.
 ¯¯¯¯¯¯AO
¯¯¯¯¯¯AO
View Solutionఒక నాణెన్నీ 100 సార్లు ఎగురవేసినప్పుడు పర్యవసానాలు కింది విధంగా ఉన్నాయి. బొమ్మ : 45 సార్లు , బొరుసు : 55 సార్లు అయిన . ప్రతి పర్యవసానం యొక్క సంభావ్యత కనుక్కోండి.
View SolutionIn the following figure , ABC is a right triangle right angled at A. BCED ACFG and ABMN and squares on the sides BC, CA and AB respectively , Line segement AX ⊥ DE meets BC at Y. Show that
(i) ti⟩MBC=ti⟩(MBC)
(ii) ar (BXYD) = 2 ar (MBC)
(iii) ar (BYXD) = ar(ABMN)
(iv) ti⟩FCB=ti⟩ACE
(v) ar (CYXE) = 2 ar (FCB)
(vi) ar (CYXE ) = ar (ACFG)
(vii) ar (BCED) = ar (ABMN) + ar (ACFG)
View Solution3.28నిpq రూపంలో రాయండి. (ఇక్కడ q!=0 మరియు p,q లు పూర్ణ సంఖ్యలు).
View SolutionIn the side map AC=XD, c మరియు D లు AB మరియు XY ల మధ్య బిందువులు. అయిన Show AB = XY.
View Solutionకింది పటంలో AC=XD, c మరియు D లు AB మరియు XY ల మధ్యబిందువులు వరుసగా అయిన AB మరియు XY లు .
View SolutionA right triangle is a right angle A in ABC. The squares BCED, ACFG, and ABMN are drawn on BC, CA, and AB, respectively. AD, AE are combined. In the same way BF and CM are combined. (See map). Prove the following though. (BYXD) Y || = (ABMN) Why ||
View SolutionA right triangle is a right angle A in ABC. The squares BCED, ACFG, and ABMN are drawn on BC, CA, and AB, respectively. AD, AE are combined. In the same way BF and CM are combined. (See map). Prove the following though. /_\FCB~=/_\ACE
View SolutionA right triangle is a right angle A in ABC. The squares BCED, ACFG, and ABMN are drawn on BC, CA, and AB, respectively. AD, AE are combined. In the same way BF and CM are combined. (See map). Prove the following though. (CYXE) Y || = (ACFG) Why ||
View SolutionA right triangle is a right angle A in ABC. BCED, ACFG and ABMN are the squares drawn on BC, CA and AB, respectively. AX _|_DE , Denounces BC at Y and DE at X. AD, AE are combined. In the same way BF and CM are combined. (See map). Prove the following though. (BCED) Why || =(ABMN) Why || +(ACFG) Why || (vii) Write the result in words.
View SolutionABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB espectively. Line segments AX bot DE meets BC at Y. and DE at X. Join AD, AE also BF and CM (See the figure). Show that (i) DeltaMBC ~=DeltaABD
View SolutionIn shape, ABC There is a right angled triangle whose angle A is right angled. BCED,ACFG And ABMN sides respectively BC, CA And AB There are classes built on. Line segment AX_|_DE arm BC meets at point Y. Show that: ar (BCED) =ar (ABMN) + ar (ACFG)
View SolutionABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segent AX bot DE meets BC at Y. Show that: triangleMBC congtriangleABD
View SolutionABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segent AX bot DE meets BC at Y. Show that: ar(BYXD) =2ar(MBC)
View SolutionABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segent AX bot DE meets BC at Y. Show that: ar(BYXD) = ar(ABMN)
View Solution
