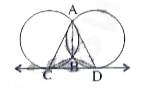
In the adjoining figure AT and BT are the to two tangents at A and B respectively CD is also a tangent at P.
There are some more circles touching each other and the tangents AT and BT also. Which one of the following is true?

$P C + C T = P D + D T$

$R G + G T = R H + H T$

$P C + Q E = C E$

all of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Answer

Step by step video, text & image solution for In the adjoining figure AT and BT are the to two tangents at A and B respectively CD is also a tangent at P. There are some more circles touching each other and the tangents AT and BT also. Which one of the following is true? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.

Updated on:21/07/2023

Class 14 MATHS GEOMETRY

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
What can be the maximum number of common tangent which can be drawn to two non-intersecting circles?
AInfinity
B0
C2
D3
Question 2 - Select One
In the following figure, O is the centre of the circle. AB and CD are equal chords. If $∠ A O B = 100^{\circ}$ , Find the $∠ C E D$ .

A $A C + A B = B C$
B $3 A E = A B + B C + A C$
C $A B + B C + A C = 4 A E$
D $2 A E = A B + B C + A C$
Question 3 - Select One
If two circles touch each other internally, only _____ common tangent can be drawn to them.
A0
B1
C2
D3

ARIHANT SSC-GEOMETRY-EXERCISE(LEVEL 1)

Home
Ask Doubt
Profile

In the adjoining figure AT and BT are the to two tangents at A and B respectively CD is also a tangent at P. There are some more circles touching each other and the tangents AT and BT also. Which one of the following is true?

The correct Answer is:D

Topper's Solved these Questions

Similar Questions

Knowledge Check

What can be the maximum number of common tangent which can be drawn to two non-intersecting circles?

In the following figure, O is the centre of the circle. AB and CD are equal chords. If ∠AOB=100∘ , Find the ∠CED.

If two circles touch each other internally, only _____ common tangent can be drawn to them.

ARIHANT SSC-GEOMETRY-EXERCISE(LEVEL 1)

In the adjoining figure AT and BT are the to two tangents at A and B respectively CD is also a tangent at P.
There are some more circles touching each other and the tangents AT and BT also. Which one of the following is true?

In the following figure, O is the centre of the circle. AB and CD are equal chords. If $∠ A O B = 100^{\circ}$ , Find the $∠ C E D$ .