கீழ்க்கண்ட படத்தினில் குறிக்கப்பட்டுள்ள A, B, C மற்றும் D பாகங்களுக்கு சரியான விடையை தேர்ந்ந்தெடு.
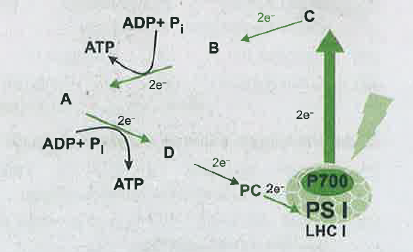
Cyt b6, பெர்ரடாக்ஸின், FRS, Cyt f
Cyt b6, FRS, பெர்ரடாக்ஸின், Cyt f
FRS, Cyt b6, பெர்ரடாக்ஸின், Cyt f
Cyt b6, Cyt f, பெர்ரடாக்ஸின், FRS
Step by step video, text & image solution for கீழ்க்கண்ட படத்தினில் குறிக்கப்பட்டுள்ள A, B, C மற்றும் D பாகங்களுக்கு சரியான விடையை தேர்ந்ந்தெடு. by Biology experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
கீழ்க்கண்டபடத்திற்குரிய சரியான விடையை தேர்ந்தெடு.
02:32View Solutionபொருத்தமான இணையைத் தேர்ந்தெடு:
01:11View SolutionIf a,b,candd are in H.P., then prove that (b+c+d)/a,(c+d+a)/b,(d+a+b)/c and (a+b+c)/d , are in A.P.
00:51View SolutionIf A=[abcd] , then adj A is [−d−b−ca] (b) [d−b−ca] (c) [dbca] (d) [dcba]
07:49View SolutionIf A={a,b},B={c,d},C={d,e} then {(a,c),(a,d)(a,e),(b,c),(b,d),(b,e)}=
05:58View SolutionIf (a+b+c+d):(a+b−c−d)=(a−b+c−d):(a−b−c+d), then prove that a:b=c:d.
View SolutionIf (a+b+c+d)(a−b−c+d)=(a+b−c−d)(a−b+c−d), then prove that a:b=c:d
01:42View SolutionI : If a,b,c,d are four vectors then [b c d] a + [c a d] b + [a b c] c = [a b c]d
II : The points with position vectors a,b,c,d are coplanar then
[a b d] + [b c d] + [c a d] = [a b c]01:47View Solutionसत्य /असत्य
यदि A = {a, b, c, d) तथा फलन f ={(a,b), (b,d),(c,a),(d,c)} , तब f−1 = {(b,a),(d,b),(a,c),(c,d) } है।02:59View SolutionIf A={a,b},B={c,d},C={d,e}, then : {(a,c),(a,d),(a,e),(b,c),(b,d),(b,e)} is :
02:42View Solutionபின்வரும் கணங்களைப் பட்டியல் முறையில் எழுதுக ?C={x:x என்பது ஒரு முழுக் கன எண் மற்றும் 27 < x < 216}
05:36View Solutionn(A)=25,n(B)=40,n(A∪B)=50 மற்றும் n(B)=25எனில்,n(A nn B) மற்றும்n(U) காண்க ?
03:05View Solutionz1=2−iமற்றும்z2=−4+3iஎனில்,z_1 z_2மற்றும்z_1/z_2 -ன் நேர்மாறைக் காண்க.
04:40View Solutionபின்வரும் வகையீடுகளைப் பொருத்துக
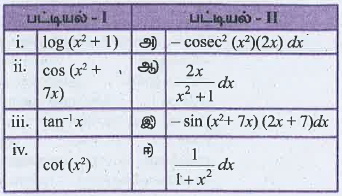. சரியான பொருத்தமானது01:52View SolutionIf four sides of a quadrilateral ABCD are tangential to a circle, then AC+AD=BD+CD (b) AB+CD=BC+AD (c) AB+CD=AC+BC (d) AC+AD=BC+DB
02:22View SolutionIn Figure, AD=BC and BD=CA. Prove that ∠A D B= ∠B C A a n d ∠D A B=∠ C B A.
01:54View Solution