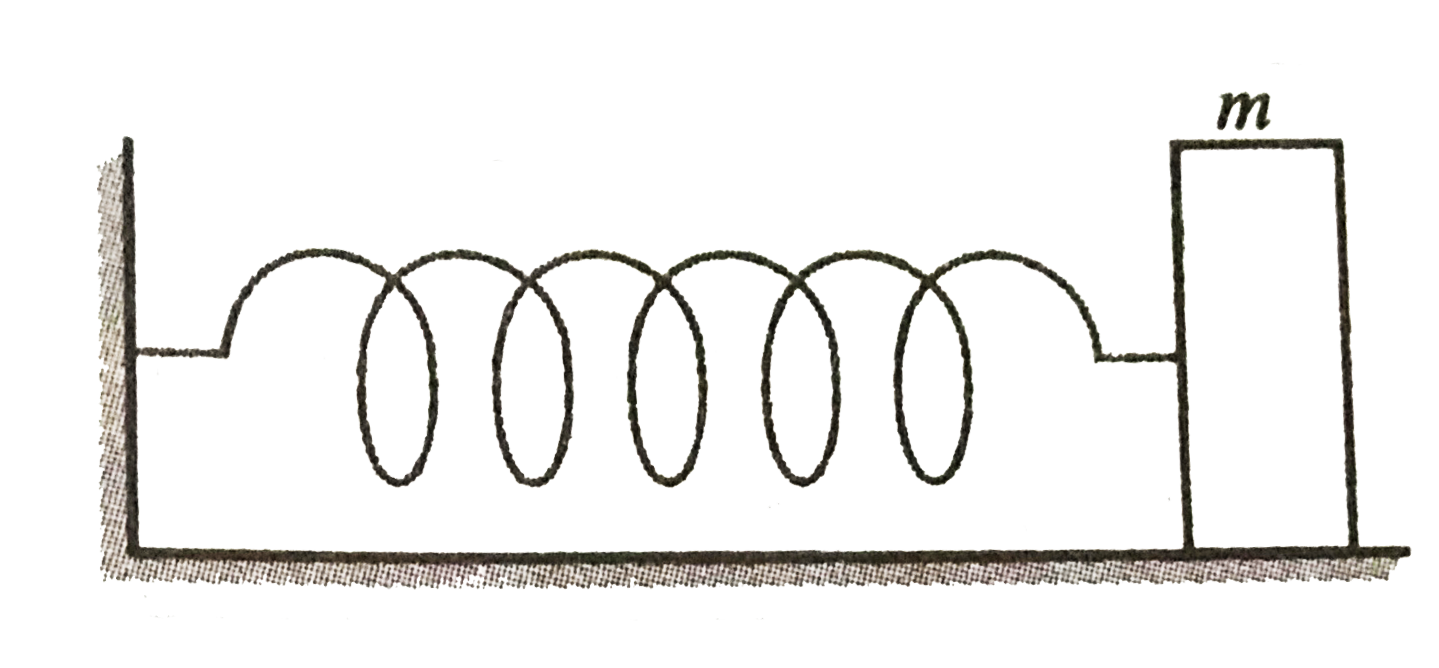
A spring of force constant $1200 N m^{- 1}$ is mounted on a horizontal table as shown in figure. A mass of 3.0kg is attached to the free end of the spring, pulled side ways to a distance of 2.0cm and released. Determing.
(a) the frequency of oscillation of the mass.
(b) the maximum acceleration of the mass.
(c) the maximum speed of the mass.

Video Solution

Text Solution

Verified by Experts

K = 1200 $N m^{- 1}$
m = 3kg
Displacement = y = 2 cm = 0.02 m
(a) frequency = $v = \frac{1}{T} = \frac{1}{2 π} \sqrt{\frac{K}{m}}$
$= \frac{1}{2 \times 3.14} \sqrt{\frac{1200}{s}} = 3.18 s^{- 1}$
(b) Maximum acceleration $A = ω^{2} y$
$ω = \sqrt{\frac{K}{m}}$
$∴ A = \frac{k y}{m}$
$= \frac{1200 \times 0.02}{3} = 8 m s^{- 2}$
( c ) maximum speed $= v = y ω$
$= 0.02 \sqrt{\frac{K}{m}} = 0.02 \sqrt{\frac{1200}{3}} = 0.4 m s^{- 1}$

Updated on:21/07/2023

A spring of force constant $600 N m^{- 1}$ is mounted on a horizontal table. A mass of 1.5 kg is attached to the free end of the spring,pulled sideways to a distance of 2 cm and released . The speed of the mass when the spring is compressed by 1 cm is
A $0.175 m s^{- 1}$
B $0.35 m s^{- 1}$
C $0.7 m s^{- 1}$
D $1.4 m s^{- 1}$
A spring of force constant $600 N m^{- 1}$ is mounted on a horizontal table. A mass of 1.5 kg is attached to the free end of the spring, pulled sideways to a distance of 2 cm and released .P.E. of the mass when it momentarily comes to rest and total energy are
A $0.12 J, 0$
B $0, 0.12 J$
C $0, 0$
D $0.12 J, 0.12 J$
Two springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is
A $\frac{1}{2 π} \sqrt{\frac{m}{k}}$
B $\frac{1}{2 π} \sqrt{2 \frac{k}{m}}$
C $\frac{1}{2 π} \sqrt{3 \frac{k}{m}}$
D $\frac{1}{2 π} \sqrt{\frac{m}{k}}$