A horizontal platform is undergoing vertical S.H>M of time period T. A block of mass M is placed on the platform . The maximum mplitude of the platform so that the block is not detached form it is
gT22π⋅2
gT24π2
2gT22π2
4gT2π2
The correct Answer is:B
To solve the problem, we need to determine the maximum amplitude A of the platform undergoing vertical simple harmonic motion (SHM) such that the block of mass M does not detach from the platform.
1. Understanding Forces Acting on the Block:
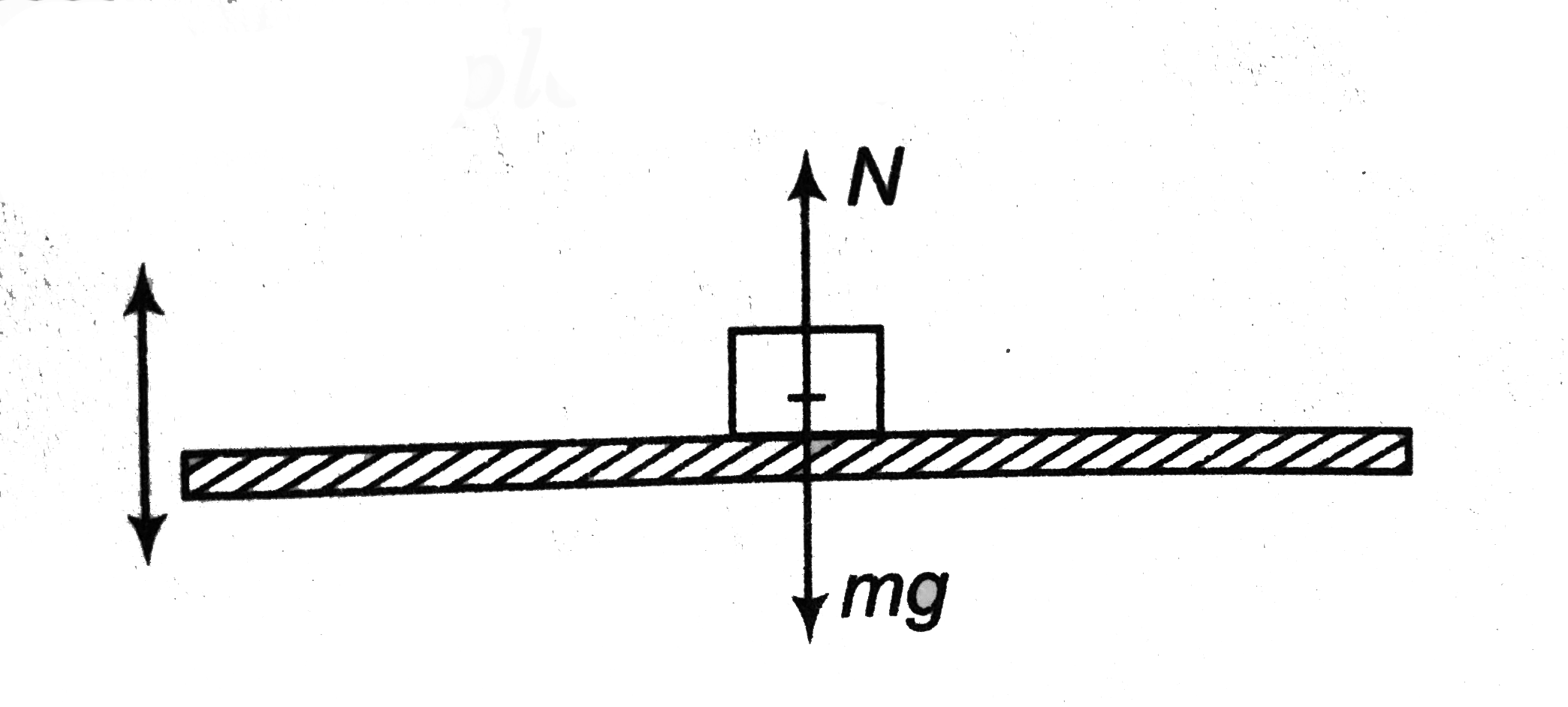
The block experiences two forces: the gravitational force acting downwards, Mg, and the normal force N acting upwards from the platform. The block will not detach from the platform as long as the normal force N is greater than or equal to zero.
2. Applying Newton's Second Law:
According to Newton's second law, the net force acting on the block in the vertical direction can be expressed as:
N−Mg=Ma
where a is the acceleration of the block due to the SHM of the platform.
3. Acceleration of the Platform:
The acceleration a of the platform can be expressed in terms of the amplitude A and angular frequency ω:
a=−ω2Asin(ωt)
Here, ω=2πT, where T is the time period of the SHM.
4. Substituting Acceleration into the Force Equation:
Substituting the expression for a into the force equation gives:
N−Mg=−Mω2Asin(ωt)
Rearranging this, we find:
N=Mg−Mω2Asin(ωt)
5. Condition for the Block to Remain in Contact:
For the block to remain in contact with the platform, the normal force N must be greater than or equal to zero:
Mg−Mω2Asin(ωt)≥0
This simplifies to:
g≥ω2Asin(ωt)
6. Finding Maximum Value of Sine Function:
The maximum value of sin(ωt) is 1. Therefore, we can write:
g≥ω2A
Rearranging this gives:
A≤gω2
7. Substituting for Angular Frequency:
Since ω=2πT, we have:
ω2=(2πT)2=4π2T2
Substituting this into the inequality gives:
A≤gT24π2
8. Conclusion:
Therefore, the maximum amplitude A of the platform so that the block does not detach from it is:
A=gT24π2
Final Answer:
The maximum amplitude of the platform so that the block is not detached from it is A=gT24π2.
The block experiences two forces: the gravitational force acting downwards, Mg, and the normal force N acting upwards from the platform. The block will not detach from the platform as long as the normal force N is greater than or equal to zero.
According to Newton's second law, the net force acting on the block in the vertical direction can be expressed as:
N−Mg=Ma
where a is the acceleration of the block due to the SHM of the platform.
The acceleration a of the platform can be expressed in terms of the amplitude A and angular frequency ω:
a=−ω2Asin(ωt)
Here, ω=2πT, where T is the time period of the SHM.
Substituting the expression for a into the force equation gives:
N−Mg=−Mω2Asin(ωt)
Rearranging this, we find:
N=Mg−Mω2Asin(ωt)
For the block to remain in contact with the platform, the normal force N must be greater than or equal to zero:
Mg−Mω2Asin(ωt)≥0
This simplifies to:
g≥ω2Asin(ωt)
The maximum value of sin(ωt) is 1. Therefore, we can write:
g≥ω2A
Rearranging this gives:
A≤gω2
Since ω=2πT, we have:
ω2=(2πT)2=4π2T2
Substituting this into the inequality gives:
A≤gT24π2
Therefore, the maximum amplitude A of the platform so that the block does not detach from it is:
A=gT24π2
The maximum amplitude of the platform so that the block is not detached from it is A=gT24π2.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (Multiple choice questions) AIPMT/NEET other State Boards for Medical Entrance14videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition file (Multiple choice question) JEE (Main) & Other State Boards for Engineering Entrance25videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Revision Exercises (Numerical Problems)23videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST16videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
Similar Questions
A block is kept is kept over a horizontal platform, executing vertical SHM of angular frequency ω. Find maximum amplitude of oscillations, so that the block does not leave contact with the platform.
 View Solution
View SolutionA horizontal platform with an object placed on it is executing SHM in the vertical direction. The amplitude of oscillation is 4×10−3m. The least period of these oscillations, so that the object is not detached from the platform is π5x second. Find value of x. Take g=10m/s2
View Solution
Knowledge Check
- Question 1 - Select One
A block of 0.5kg is placed on a horizontal platform. The system is making vertical oscillations about a fixed point with a frequency of 0.5Hz. The maximum displacement of oscillation, if the block does not lose contact with the horizontal platform is nearly
A0.5mB0.75mC1mD1.2m - Question 2 - Select One
A horizontal platform with an object placed on it is executing S.H.M. in the vertical direction. The amplitude of oscillation is 2.5 cm. If the object is not detached from the platform, then the least period of these oscillations will be, (g=10m/s2)
A0.1πsBπsC0.5πsD2πs - Question 3 - Select One or More
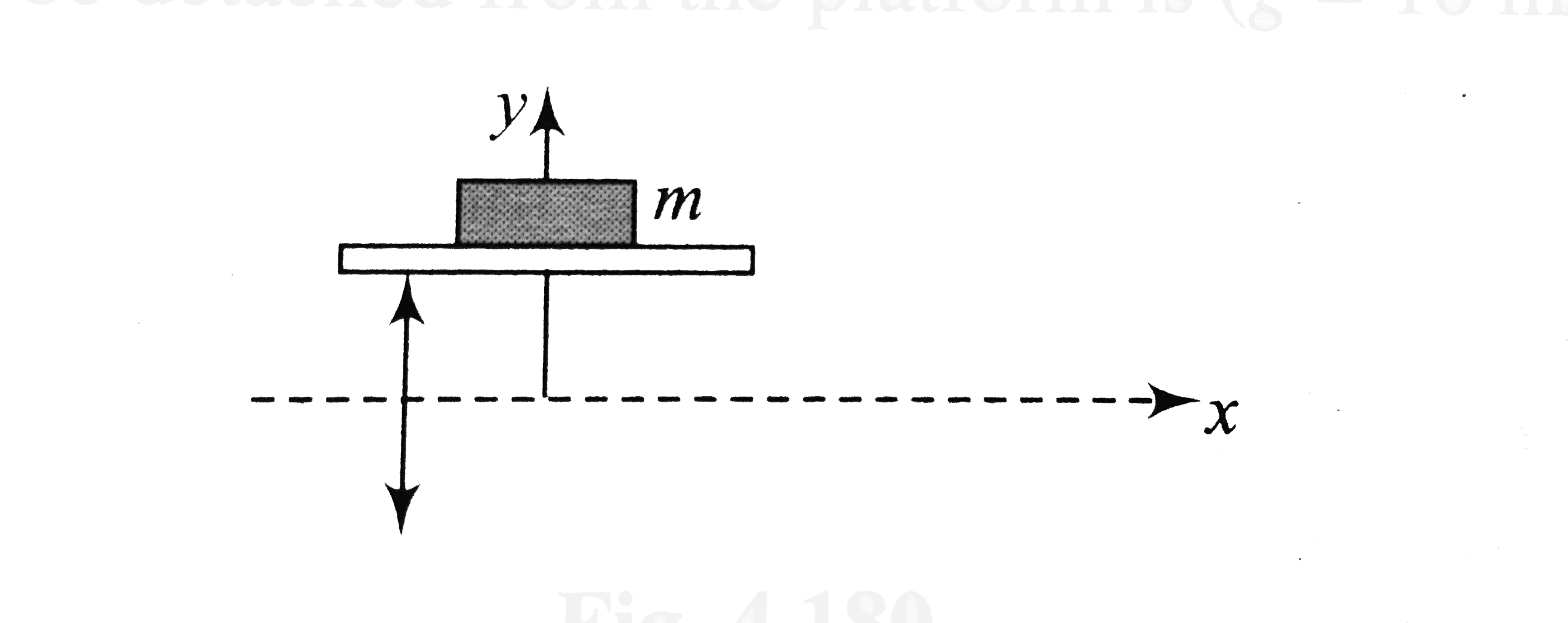
A horizontal platform with a mass m placed on it is executing SHM slong y-axis. If the amplitude of oscillation is 2.5 cm, the minimum period of the motion for the mass not to be detached from the platform (g=10ms2)A10πsBπ10sCπ√10sD1√10s
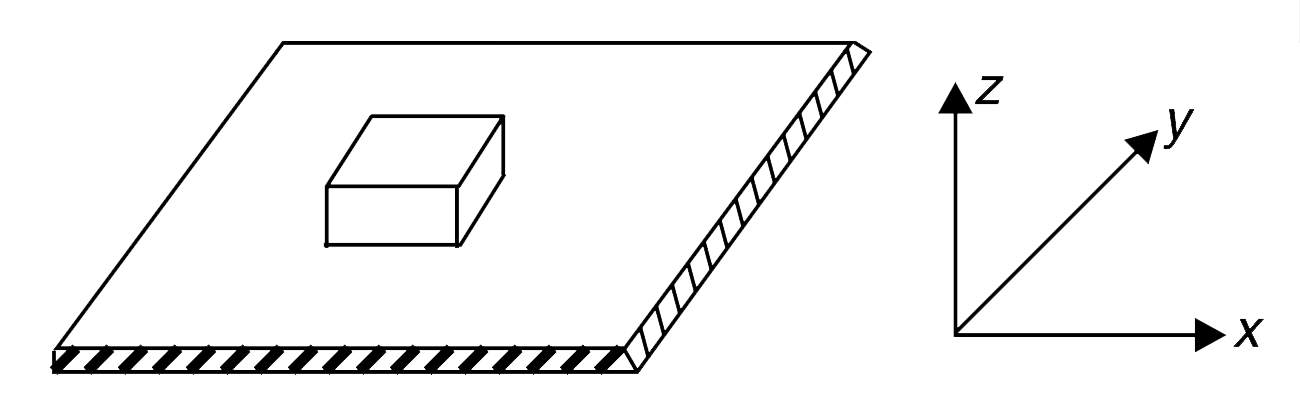
A solid block of mass m = 1 kg is resting on a horizontal platform as shown in figure. The z direction is vertically up. Coefficient of friction between the block and the platform is μ=0.2. The platform is moved with a time dependent velocity givenby →V=(2tˆi+tˆj+3tˆk)m/s . Calculate the magnitude of the force exerted by the block on the platform. Take g=10m/s2
 View Solution
View SolutionA coin is placed on a horizontal platform which undergoes vertical simple harmonic motion of angular frequency ω. The amplitude of oscillation is gradually increased. The coin will leave contact with the platform for the first time :
View SolutionA coin of mass m is placed on a horizontal platform which is undergoing S.H.M. about a mean position O in horizontal plane. The force of friction on the coin is f. while the coin does not slip on the platform f is
View SolutionA coin is placed on a horizontal platform which undergoes vertical simple harmonic motion of angular frequency ω. The amplitude of oscillation is gradually increased. The coin will leave contact with the platform for the first time
View SolutionA coin is placed on a horizontal platform which undergoes vertical simple harmonic motion of angular frequency ω. The amplitude of oscillation is gradually increased. The coin will leave contact with the platform for the first time :
View Solution

