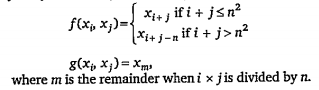The following functions are defined for the set of variables x1,x2,x3,...xn
f(xi,xj)={xi+jifj≤n2xi+j−nifi+j>n2
g(xi,xj)=xm,
where m is the remainder when i×j is divided by n.
Find the value of g(g(x2,x3),g(x7,x8) if n=5
A
x1
B
x2
C
x5
D
none of these
Text Solution
The correct Answer is:A
|
Topper's Solved these Questions
- FUNCTIONS AND GRAPHBOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - Final Round
40 Videos
- FUNCTIONS AND GRAPHBOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - EXERCISE(LEVEL 1)
84 Videos
- ELEMENTS OF ALGEBRABOOK - ARIHANT SSCCHAPTER - ELEMENTS OF ALGEBRAEXERCISE - EXERCISE(LEVEL 1)
32 Videos
- FUNDAMENTALS BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 2
40 Videos
Similar Questions
Knowledge Check
The following functions are defined for the set of variables x1,x2,x3,...xn
f(xi,xj)={xi+jifj≤n2xi+j−nifi+j>n2
g(xi,xj)=xm,
where m is the remainder when i×j is divided by n.
Find the value of f(f(x2,x3),f(x5,x6) if n=3Ax5Bx10Cx13Dnone of theseIf ¯x is the mean of x1,x2,…,xn then the value of n∑i=1(xi−¯x) is
A0B2¯xC¯xD¯x2If the mean of a set of observations x1,x2,…,xn is ¯¯¯X, then the mean of the observations xi+2i,i=1,2,...,n is
A¯x+2B¯x+nC¯x+2nD¯x+n+1
If the mean of the set of numbers x1,x2,...xn is ¯x, then the mean of the numbers xi+2i, 1≤i≤n is
View SolutionThe standard deviation of n observations x1,x2,......,xn is 2. If n∑i=1xi=20 and n∑i=1x2i=100 then n is
View SolutionThe standard deviation of n observationsx1,x2,x3,…xn is 2. If n∑i=1x2i=100 and n∑i=1xi=20 show the values of n are 5 or 20
View SolutionBY Remainder theorem , find the remainder when p(x) is divided by g(x) (i) p(x) =x3−2x2−4x−1,g(x)=x+1 (ii) p(x)=x3−3x2+4x+50,g(x)=x−3
View SolutionBY Remainder theorem , find the remainder when p(x) is divided by g(x)
(i) p(x) =x3−2x2−4x−1,g(x)=x+1
(ii) p(x)=x3−3x2+4x+50,g(x)=x−3
View Solution