In a system of four unequal particles located in an arrangement of non - linear, coplanar system, the centre of mass
must lie within or at the edge at least one of the triangles formed by any three particles.
may lie within or outside the closed figure formed by joining the extreme particles by straight lines.
must lie within the closed figure formed by joining the extreme particles by straight line
does not exist
The correct Answer is:A, C
Topper's Solved these Questions
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - (COMPREHENSIONS - I)
2 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - (COMPREHENSIONS - II)
3 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) Level - I (ASSERTION REASONING TYPE)
2 Videos
- AC CIRCUITSBOOK - FIITJEECHAPTER - AC CIRCUITSEXERCISE - ASSERTION REASONING TYPE
1 Videos
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Comprehension -4
3 Videos
Similar Questions
If all the particle of a system lie in a cube is it necessary that the centre of mass be in the cube?
View SolutionThe position co-ordinates of the centre of mass of a system of three particles of equal masses which are placed on the verctices of an equilateral triangle of a side a are shown. Locate centre mass?
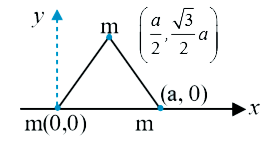
View Solution
Knowledge Check
The sum of moments of masses of all the particle in a system about the centre of mass is always
AzeroBmaximumCinfiniteDminimumFour identical particles each of mass m are arranged at the corners of a square of side length l. If the masses of the particles at the end of a side are doubled, the shift in the centre of mass of the system.
Al6Bl6√2Cl√2Dl5√2The centre of mass of two particles system lies
Aat the midpoint on the line joining the two particles.Bat one end of line joining the two particlesCon the line perpendicular to the line joining two particlesDon the line joining the two particles
The position co-ordinates of the centre of mass of a system of three particles of equal masses which are placed on the verctices of an equilateral triangle of a side a are shown. Locate centre mass?
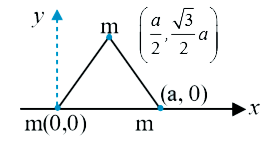
View SolutionStatement-1 : The centre of mass of a system of n particles is the weighted average of the position vector of the n particles making up the system
. Statement-2 : The position of the centre of mass of a system is independent of coordinate system
View SolutionA system consists of 3 particles each of mass m located at points (1, 1), (2, 2) and (3, 3). The coordinates of the centre of mass are
View SolutionA system consists of 3 particles each of mass 'm' are located at (1, 1) (2, 2) and (3, 3). The co-ordinates of the centre of mass are
View SolutionThe centre of mass of a system of particles does not depend on
View Solution
