A wire of certain length carries a steady current. It is first bent to form a circular wire of one turn. The same wire is bent to form a circular coil of three turns. The ratio of magnetic induction at the centre of the coil in the two cases is ________
The correct Answer is:.1 : 9
To solve the problem, we need to find the ratio of the magnetic induction (magnetic field) at the center of a circular coil formed from a wire of length L when it is bent into one turn and then into three turns.
1. Understanding the Problem:
- We have a wire of length L carrying a steady current I.
- The wire is first bent to form a circular coil with 1 turn and then with 3 turns.
- We need to find the ratio of the magnetic induction at the center of the coil in both cases.
2. Magnetic Field for 1 Turn:
- When the wire is bent into 1 turn, the circumference of the circular coil is equal to the length of the wire:
2πr=L⟹r=L2π
- The magnetic field B1 at the center of a circular coil with radius r and carrying current I is given by:
B1=μ04π⋅2πIr
- Substituting r:
B1=μ04π⋅2πIL2π=μ0I2L
3. Magnetic Field for 3 Turns:
- When the wire is bent into 3 turns, the total length of the wire is still L, so the circumference for one turn is:
3⋅2πr′=L⟹r′=L6π
- The magnetic field B2 at the center of this coil is given by:
B2=μ04π⋅2π(3I)r′
- Substituting r′:
B2=μ04π⋅2π(3I)L6π=μ04π⋅12πIL=3μ0IL
4. Finding the Ratio B1B2:
- Now, we find the ratio of the magnetic fields:
B1B2=μ0I2L3μ0IL=12⋅L3=16
- Therefore, the ratio of the magnetic induction at the center of the coil in the two cases is:
B1B2=16
Final Answer:
The ratio of magnetic induction at the center of the coil in the two cases is 1:6.
- We have a wire of length L carrying a steady current I.
- The wire is first bent to form a circular coil with 1 turn and then with 3 turns.
- We need to find the ratio of the magnetic induction at the center of the coil in both cases.
- When the wire is bent into 1 turn, the circumference of the circular coil is equal to the length of the wire:
2πr=L⟹r=L2π
- The magnetic field B1 at the center of a circular coil with radius r and carrying current I is given by:
B1=μ04π⋅2πIr
- Substituting r:
B1=μ04π⋅2πIL2π=μ0I2L
- When the wire is bent into 3 turns, the total length of the wire is still L, so the circumference for one turn is:
3⋅2πr′=L⟹r′=L6π
- The magnetic field B2 at the center of this coil is given by:
B2=μ04π⋅2π(3I)r′
- Substituting r′:
B2=μ04π⋅2π(3I)L6π=μ04π⋅12πIL=3μ0IL
- Now, we find the ratio of the magnetic fields:
B1B2=μ0I2L3μ0IL=12⋅L3=16
- Therefore, the ratio of the magnetic induction at the center of the coil in the two cases is:
B1B2=16
The ratio of magnetic induction at the center of the coil in the two cases is 1:6.
Topper's Solved these Questions
- MAGNETICBOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Assignment Problems (Subjective) (Level - II & III)
15 Videos
- MAGNETICBOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Assignment Problems (Objective) (LEVEL-I)
50 Videos
- MAGNETICBOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Exercise
11 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III
2 Videos
- MAGNETISMBOOK - FIITJEECHAPTER - MAGNETISMEXERCISE - Example
12 Videos
Similar Questions
A direct current is passing through a wire. It is bent to form a coil of one turn. Now it is further bent to form a coil of two turns but at smaller radius. The ratio of the magnetic induction at the centre of this coils and at the centre of the coil of two turn is
View SolutionA wire of length L carries a steady current I. It is bent first to form a circular plane coil of one turn. The same length is now bent more sharply to give a double loop of smaller radius. The magnetic field at the centre caused by the same current is
View Solution
Knowledge Check
A wire is bent in the form of a circular arc with a straight portion AB. Magnetic induction at O when current flowing in the wire, is
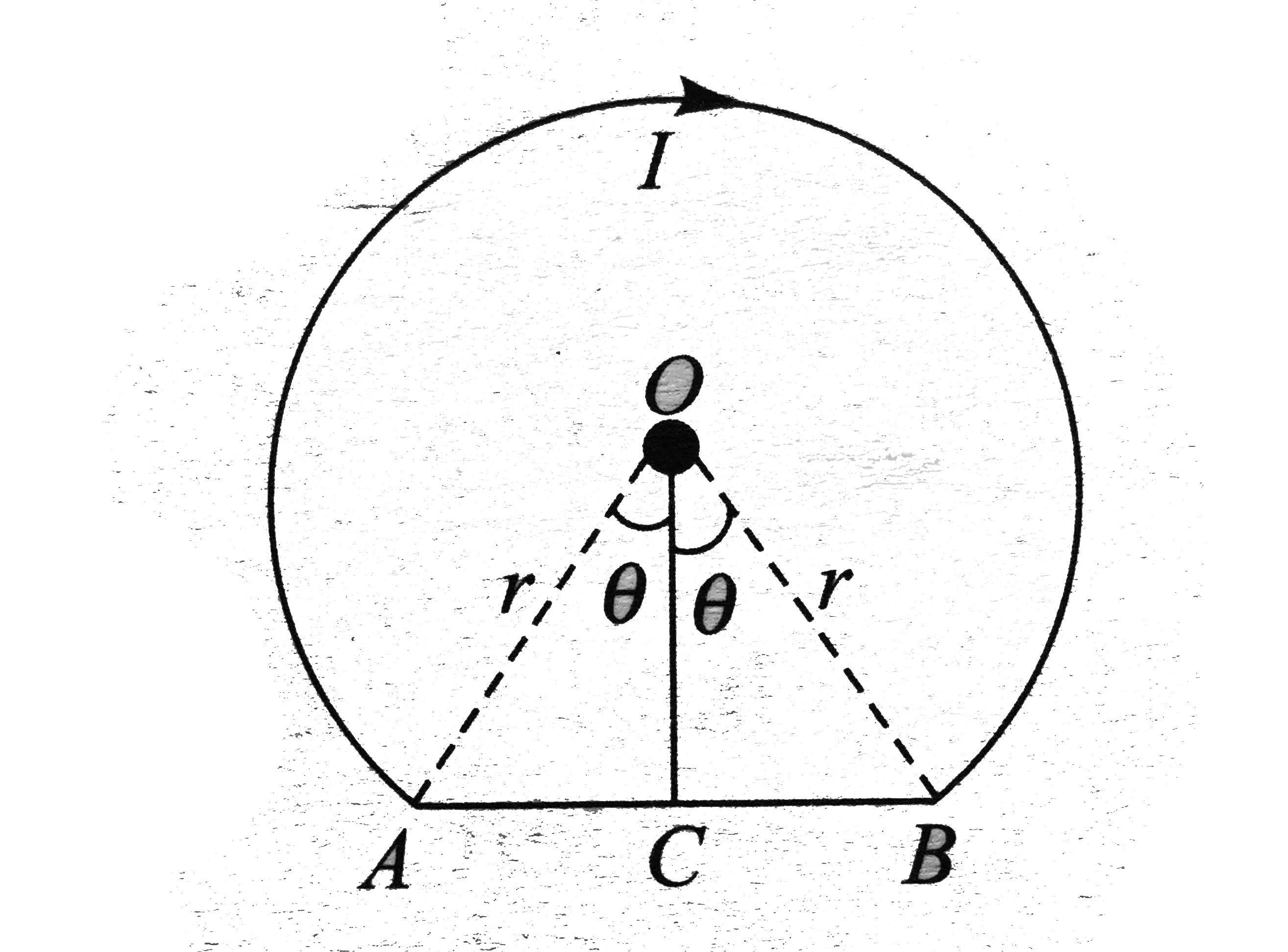 Aμ02r(π−θ+tanθ)Bμ0I2πR(π+θ−tanθ)Cμ0I2πR(π−θ+tanθ)Dμ0I2πR(−tanθ+π−θ)
Aμ02r(π−θ+tanθ)Bμ0I2πR(π+θ−tanθ)Cμ0I2πR(π−θ+tanθ)Dμ0I2πR(−tanθ+π−θ)A long wire carries a steady curent . It is bent into a circle of one turn and the magnetic field at the centre of the coil is B. It is then bent into a circular loop of n turns. The magnetic field at the centre of the coil will be
A2nBBn2BCnBD2n2BA length of wire carries a steady current. It is first bent to form a circular coil of one turn. The same length is now bent more sharply to give a loop of two turns of smaller radius. The magentic field at the centre caused by the same current now will be
Aa quarter of its first valueBsame as that of the first valueCfour times the first valueDdouble of its first value
Along wire carries a steady current. It is bent into a circle of one tum and the magnetic field at the centre of the coil is B. It is then bent into a circular loop of N tums. The magnetic field at the centre of the coil will be
View SolutionA wire of certain length carries a current l.It is bent to form a circle of one turn and the magnetic field at the centre is B1. If it is bent to form a coil of four turns, then magnetic field at centre is B2.The ratio of B1 and B2is
View SolutionA long wire carrying a steady current is bent into a circular loop of one turn. The magnetic field at the centre of the loop is B. It is then bent into a circular coil of n turns. The magnetic field at the centre of this coil of n turns will be
View SolutionA long wire carries a steady curent . It is bent into a circle of one turn and the magnetic field at the centre of the coil is B. It is then bent into a circular loop of n turns. The magnetic field at the centre of the coil will be
View SolutionA length of wire carries a steady current. It is bent first to form a circular coil of one turn. The same length is now bent more sharply to give a double loop of smaller radius. The magnetic field at the centre caused by the same current is
View Solution
