Consider following figure and answer the questions at the end of it.
Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
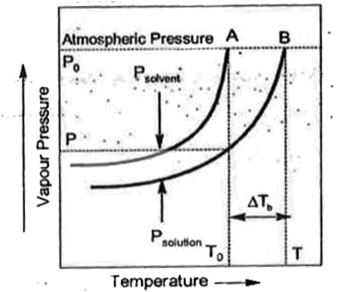
Variation of vapour pressure with temperature and showing elevation in boiling point.
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molality 'm' then Ltm→0(DηlTbm) is equal to
Kb (molal elevation constant)
Lv (latent heat of vaporization)
ΔS (entropy change)
x (mole fraction of solute)
The correct Answer is:A
Topper's Solved these Questions
- LIQUID SOLUTION BOOK - FIITJEECHAPTER - LIQUID SOLUTION EXERCISE - Comprehension - V
3 Videos
- LIQUID SOLUTION BOOK - FIITJEECHAPTER - LIQUID SOLUTION EXERCISE - Comprehension - VI
2 Videos
- LIQUID SOLUTION BOOK - FIITJEECHAPTER - LIQUID SOLUTION EXERCISE - Comprehension - III
4 Videos
- IONIC EQUILIBRIUM BOOK - FIITJEECHAPTER - IONIC EQUILIBRIUM EXERCISE - SINGLE INTEGER ANSWER QUESTIONS
4 Videos
- NUCLEIC ACID AND VITAMIN BOOK - FIITJEECHAPTER - NUCLEIC ACID AND VITAMIN EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE)
15 Videos
Similar Questions
Consider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
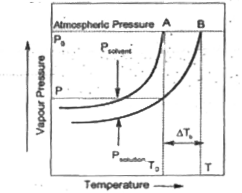
Variation of vapour pressure with temperature and showing elevation in boiling point.
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molality m then Ltm→0(ΔTbm) is equal to
View SolutionConsider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
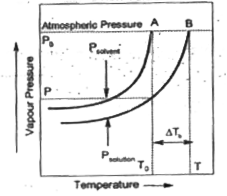
Variation of vapour pressure with temperature and showing elevation in boiling point.
Elevation in boiling point of an aqueous urea solution is 0.52∘.(Kb=0.52∘mol−1kg). Hence, mole fraction of urea in this solution is:
View Solution
Knowledge Check
Consider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.

Variation of vapour pressure with temperature and showing elevation in boiling point.
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molality m then Ltm→0(ΔTbm) is equal toAKb (molal elevation constant)BLy (latent heat of vaporization)CΔS (entropy change)Dx (mole fraction of solute)Figure explains elevation in boiling point when a non-volatile solute is added to a solvent. Variation of vapour pressure with temperaure and elevation in boiling point is marked.
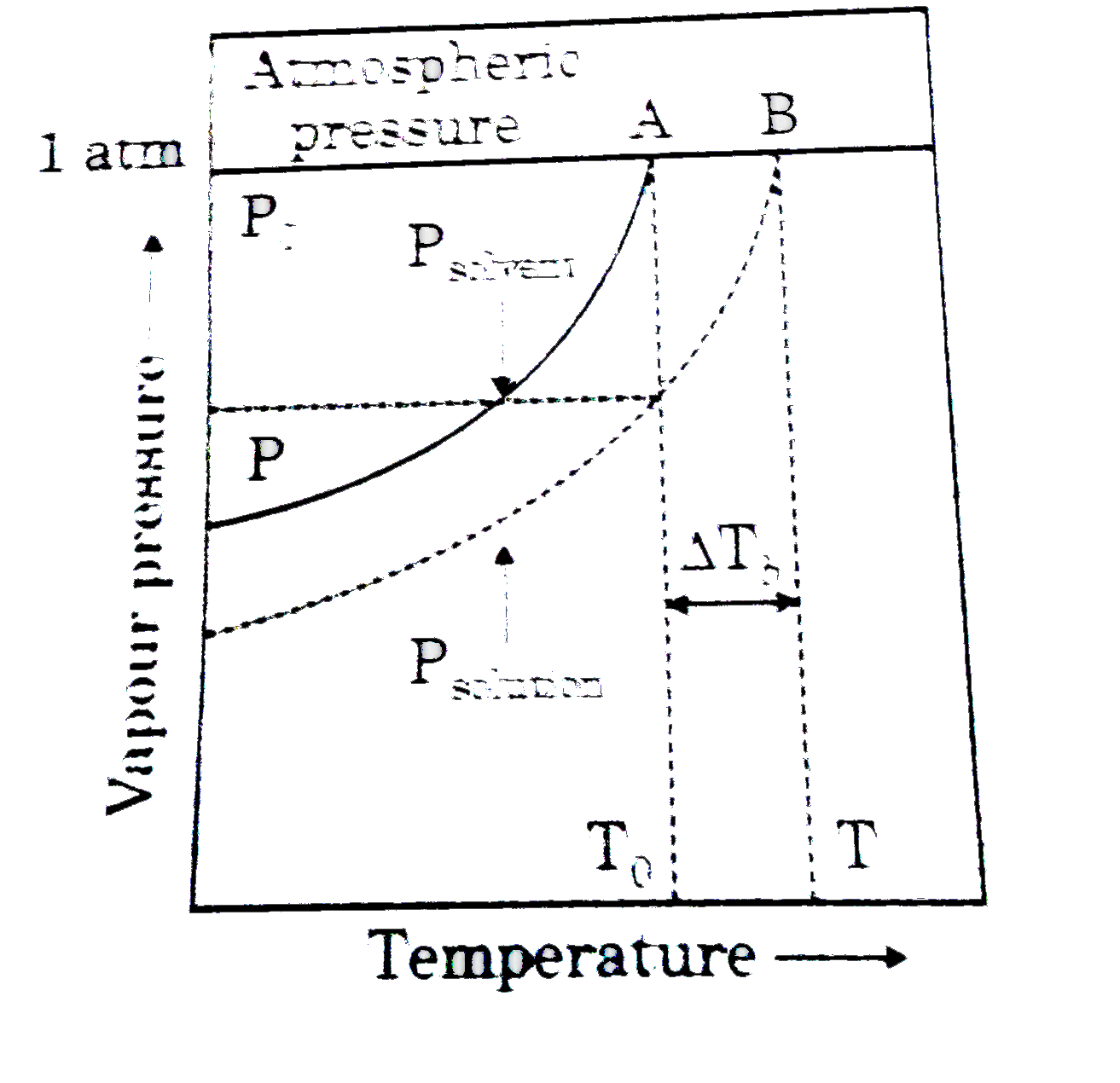
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molarity m then limm→0(ΔTbm) is equal to:AKb (molal elevation constant)BLv (latent heat of vaporisation)CΔS (entropy change)Dx (mole fraction of solute)Consider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.

Variation of vapour pressure with temperature and showing elevation in boiling point.
Elevation in boiling point of an aqueous urea solution is 0.52∘.(Kb=0.52∘mol−1kg). Hence, mole fraction of urea in this solution is:A0.982B0.0567C0.943D0.018
Consider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
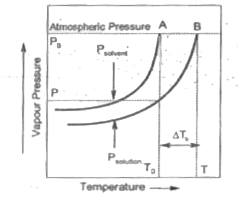
Variation of vapour pressure with temperature and showing elevation in boiling point.
A complex of iron and cyanide ions is 100% ionized at 1m (molal). If elevation in boiling point is 2.08∘(Kb=0.52∘mol−1kg) then the complex is:
View SolutionConsider following figure and answer the questions at the end of it.
Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
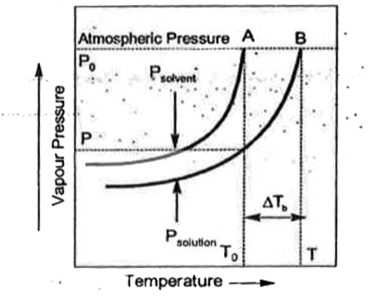
Variation of vapour pressure with temperature and showing elevation in boiling point.
Elevation in boiling point of an aqueous urea solution is 0.52∘. (Kb=0.52∘mol−1kg). Hence, mole fraction of urea in this solution is
View SolutionConsider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.

Variation of vapour pressure with temperature and showing elevation in boiling point.
A complex of iron and cyanide ions is 100% ionized at 1m (molal). If elevation in boiling point is 2.08∘(Kb=0.52∘mol−1kg) then the complex is:
View SolutionConsider following figure and answer the questions at the end of it.
Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
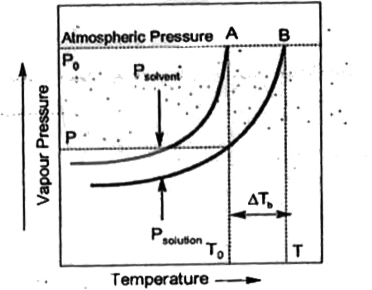
Variation of vapour pressure with temperature and showing elevation in boiling point.
A complex of iron and cyanide ions is 100% ionized at 1m (molal). If its elevation in boiling point is 2.08∘(Kb=0.52∘mol−1kg) then the complex is
View SolutionGiven that ΔTf is the depression in freezing point of the solvent in a solution of a non-volatile solute of molarity m,the quantity Ltm→0(ΔTf/m) is equal to ……………. .
View Solution
