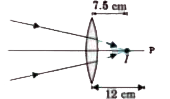
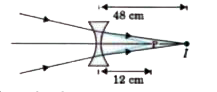
A beam of light converges to a point P. A lens is placed in the path of the convergent beam 12 cm from P. At what point does the beam converge if the lens is (a) a convex lens of focal length 20 cm. (b) a concave lens of focal length 16 cm.

Video Solution

Text Solution

Verified by Experts

Here the object is virtual.
Given, object distance, $u = + 12 c m$ (object is one the right side of the lens)
(a) For lens of focal length, $f = + 20 c m$
Using lens formula, we get
$\frac{1}{v} - \frac{1}{u} = \frac{1}{f}$

$\frac{1}{v} = \frac{1}{f} + \frac{1}{u}$
$= \frac{1}{20} + \frac{1}{12} = \frac{2}{15}$
$\Rightarrow v = 7.5 c m$ , i.e. the image on the right side of the lens and is real. It is located 7.5 cm from the lens.
(b) For lens of focal length, $f = - 16 c m$

$\frac{1}{v} = - \frac{1}{16} + \frac{1}{12}$
$= \frac{1}{48}$
$\Rightarrow v = 48 c m$ , i.e. image on the right side of the lens and is real. It is located 48 cm from the lens.

Updated on:21/07/2023

A beam of light converges at a point P. Now a concave lens of focal length -16 cm is placed in the path of the convergent beam 12 cm from P The point at which the beam converges now is
A6.86cm right side of the lens
B6.86cm left side of the lens
C48cm right side of the lens
D48cm left side of the lens
An eye specialist prescribes spectacles having combination of convex lens of focal length 40 cm in contact with a concave lens of focal length 25 cm. The power of this lens combination in diopters is
A $+ 1.5 D$
B $- 1.5 D$
C $+ 6.67 D$
D $- 6.67 D$