Question

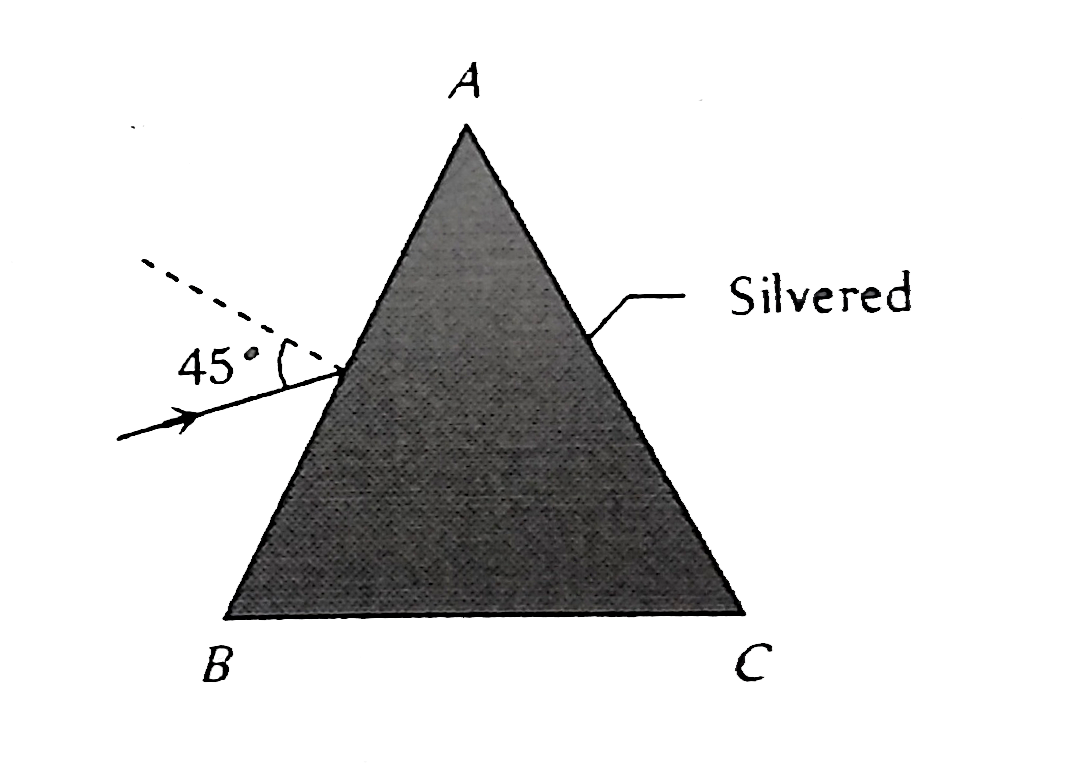
State the conditions under which total internal reflection occurs. One face of a prism with a refracting angle of ${30}^{\circ }$ is coated with silver. A ray incident on another face at an angle of ${45}^{\circ }$ is refracted and reflected from the silver coated face and retraces its path. Find the refractive index of the material of the prism.

Explanation

To solve the problem, we will follow these steps:

Step 1: State the conditions for Total Internal Reflection (TIR)
Total Internal Reflection occurs under the following conditions:
1. The light must travel from a denser medium to a rarer medium.
2. The angle of incidence must be greater than the critical angle for the pair of media.

Step 2: Analyze the given prism
We have a prism with a refracting angle of \(30^\circ\) and one face coated with silver. This means that the silvered face acts like a mirror, reflecting light instead of allowing it to pass through.

Step 3: Identify the angles involved
A ray of light is incident on the other face of the prism at an angle of \(45^\circ\). When this ray enters the prism, it will refract according to Snell's law.

Step 4: Apply Snell's Law at the first interface
Let’s denote:
- \( \mu \) = refractive index of the prism
- \( i \) = angle of incidence = \( 45^\circ \)
- \( r \) = angle of refraction

Using Snell's Law:
\( \mu = \frac{\sin i}{\sin r} \)

Step 5: Determine the angle of refraction
Since the prism has a refracting angle of \(30^\circ\), we can find the angle of refraction \(r\) after the ray passes through the prism. The angle of the prism is \(A = 30^\circ\).

The relationship between the angles is given by:
\( i + r = A \)
Thus:
\( r = A - i = 30^\circ - 45^\circ = -15^\circ \)
This indicates that the ray is actually reflecting off the silvered face.

Step 6: Determine the angle of incidence at the silvered face
The ray will hit the silvered face at an angle of \(90^\circ\) (as it retraces its path). Therefore, the angle of incidence at the silvered face is \(90^\circ\).

Step 7: Calculate the refractive index
Since the ray retraces its path, we can apply Snell's law again at the interface where it exits the prism:
\( \mu = \frac{\sin(45^\circ)}{\sin(30^\circ)} \)
Using known values:
- \( \sin(45^\circ) = \frac{1}{\sqrt{2}} \)
- \( \sin(30^\circ) = \frac{1}{2} \)

Substituting these values:
\( \mu = \frac{\frac{1}{\sqrt{2}}}{\frac{1}{2}} = \frac{2}{\sqrt{2}} = \sqrt{2} \)

Conclusion
The refractive index of the material of the prism is \( \sqrt{2} \).

---