Doubtnut Promotions Sticky

If S1andS2 are respectively the sets of local minimum and local maximum point of the function, f(x)=9x4+12x3−36x2+25,x∈R, then (a) S1={−2}:S2={0,1} (b) S1={−2,0}:S2={1} (c) S1={−2,1}:S2={0} (d) S1={−1}:S2={0,2}

A

S1={−2}:S2={0,1}

B

S1={−2,0}:S2={1}

C

S1={−2,1}:S2={0}

D

S1={−1}:S2={0,2}

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Given function is
f(x)=9x4+12x3−36x2+25=y(let)
For maxima or minima put dydx=0
⇒dxdx=36x2=−72x=0
⇒x3+x2−2x=0
⇒x[x2++x−2]=0
⇒x[x2+2x−x−2]=0
rArx[x(x+2)−1(x+2)]=0
⇒x(x−1)(x+2)=0
⇒x=−2,0,1
By sin method, we have following
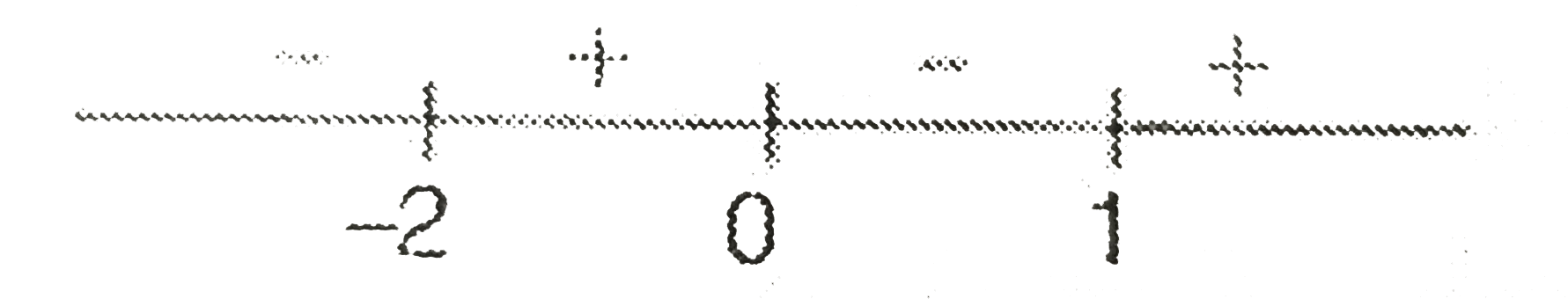
ocr_image
Since, dydx changes its' sign from negative at x='−2'and'1', so x=-2 1 are points of local minima.
Also, dydx changes it's sign from positive to negative at x=0,sox=0 is point of local maxima.
∴S1={−2,1}andS2={0}

|
Updated on:21/07/2023
Class 12MATHSAPPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    If S={x:x2+1=0,x is real }, then S is:

    A(−1)
    B(0)
    C(1)
    DAn empty set
  • Question 2 - Select One

    If S1,S2andS3 are the sums of first n natureal numbers, their squares and their cubes respectively, then S41S22−S22S23S21+S22=

    A4
    B2
    C1
    D0
  • Question 3 - Select One

    Consider the parabolas S1=y2−4ax=0 and S2=y2−4bx=0.S2 will S1, if

    Aa>b>0
    Bb>a>0
    Ca>0,b<but |b|>a
    Da<0,b>but |b|>a