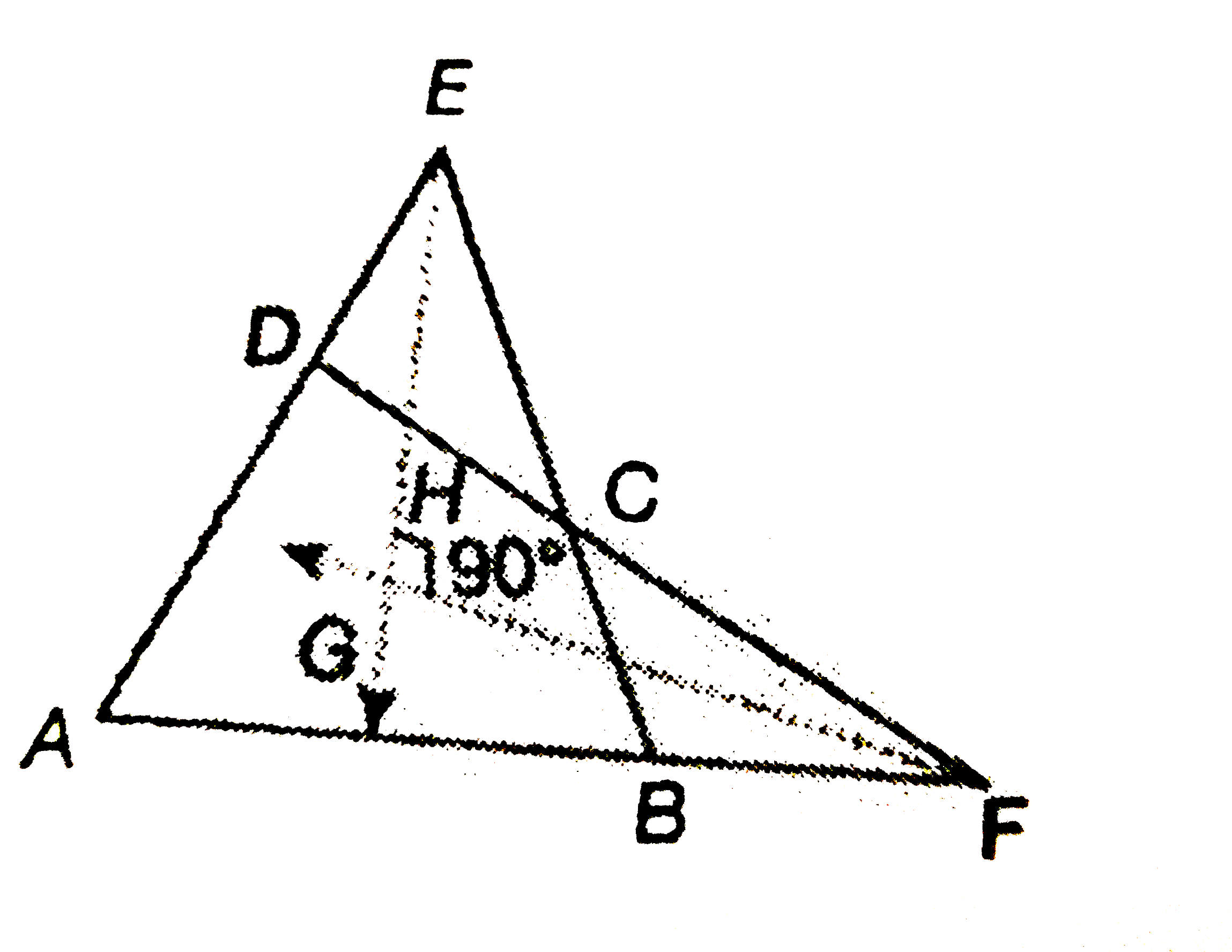
In the given figure, ABCD is a cyclic quadrilateral, ∠DAB=50% and ∠ABC=80∘. ¯¯¯¯¯¯EC and ¯¯¯¯¯¯FG are the angle bisectors of ∠DEC and ∠BFC Find ∠FHG.
A
80∘
B
90∘
C
75∘
D
105∘
Text Solution
∠DAB=50∘⇒∠DCE=50∘ (exterior angle of a cyclic quadrilateral)
∠ABC=80∘⇒∠EDC=80∘(exterior angle of a cyclic quadrilateral)
∴ln △DEC,∠DEC=180∘−(50∘+80∘)=50∘
¯¯¯¯¯¯EG is the bisector of ∠DEC
⇒∠DEH=(50∘2)=25∘
∠DHE=180∘−(∠HDE+∠DEH)=180∘−(80∘+25∘)=75∘
∠FHG=∠DHE (vertically opposite angles) ⇒∠FHG=75∘
|
Topper's Solved these Questions
- GEOMETRYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - TEST YOUR CONCEPTS
29 Videos
- GEOMETRYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - SHORT ANSWER TYPE QUESTIONS
18 Videos
- COORDINATE GEOMETRYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - Level 3
9 Videos
- LINEAR EQUATIONS AND INEQUATIONSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS AND INEQUATIONSEXERCISE - level 3
10 Videos
Similar Questions
Knowledge Check
In the given figure, ABCD is a cyclic quadrilateral and AB is diameter. ∠ADC=140∘, then find ∠BAC :
 A45∘B40∘C50∘Dnone of the above
A45∘B40∘C50∘Dnone of the aboveIf ABCD is a cyclic quadrilateral with ∠A=50∘,∠B=80∘ , then ∠C and ∠D are
A130∘,100∘B115∘,115∘C110∘,120∘D130∘,100∘In a given figure, ABCD is cyclic quadrilateral, in which ∠DAB=80∘, then measures of ∠DCE is
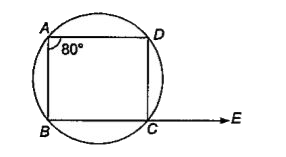 A80∘B90∘C120∘D140∘
A80∘B90∘C120∘D140∘
In the figure, □ABCD is a cyclic quadrilateral. seg AB is a diameter. If ∠ADC=120∘, find the measure of ∠BAC.
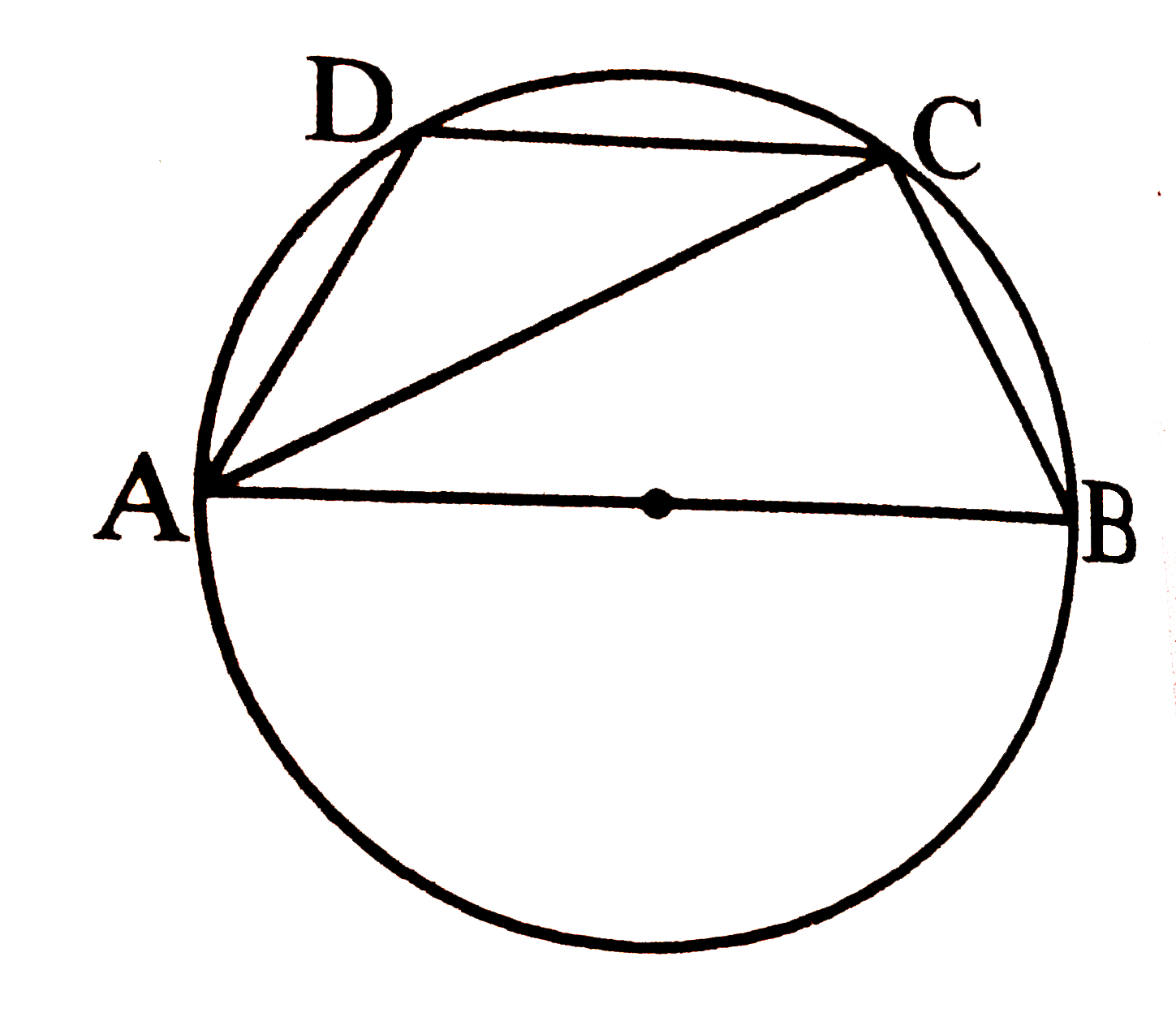
View SolutionIn the following, CDEF is a cyclic quadrilateral. ¯¯¯¯¯¯CG and ¯¯¯¯¯¯¯DH are the angle bisectors of ∠C and ∠D respectively. If ∠E=100∘ and ∠F=110∘, then find ∠CPD.

View SolutionIn the given figure, if PQRS is a cyclic quadrilateral with respective angles. Find , the ratio of x and y.
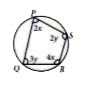
View SolutionIn the given diagram of ΔABC,∠B=80∘,∠C=30∘ BF and CF are the angle bisectors of ∠CBDand∠BCE respectively. Find the value of ∠BFC :
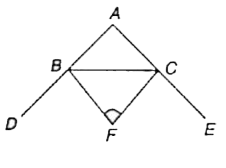
View Solution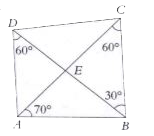
In the given figure ABCD is a quadrilateral ∠ADB=60∘,∠BAC=70∘,∠DBC=30∘,and∠ACB=60∘. Find ∠DAC
View Solution