A wooden crate with a heavy machine weighing 3000 N slides on the ground when pushed by a lever AB of length 100 cm as shown in the figure. The force required to slide the crate acts at a distance of 10 cm from the fulcrum . If the coefficient of friction between the crate and the ground is √2, find the effort required to move the crate. (Take the value of √2=1.4)
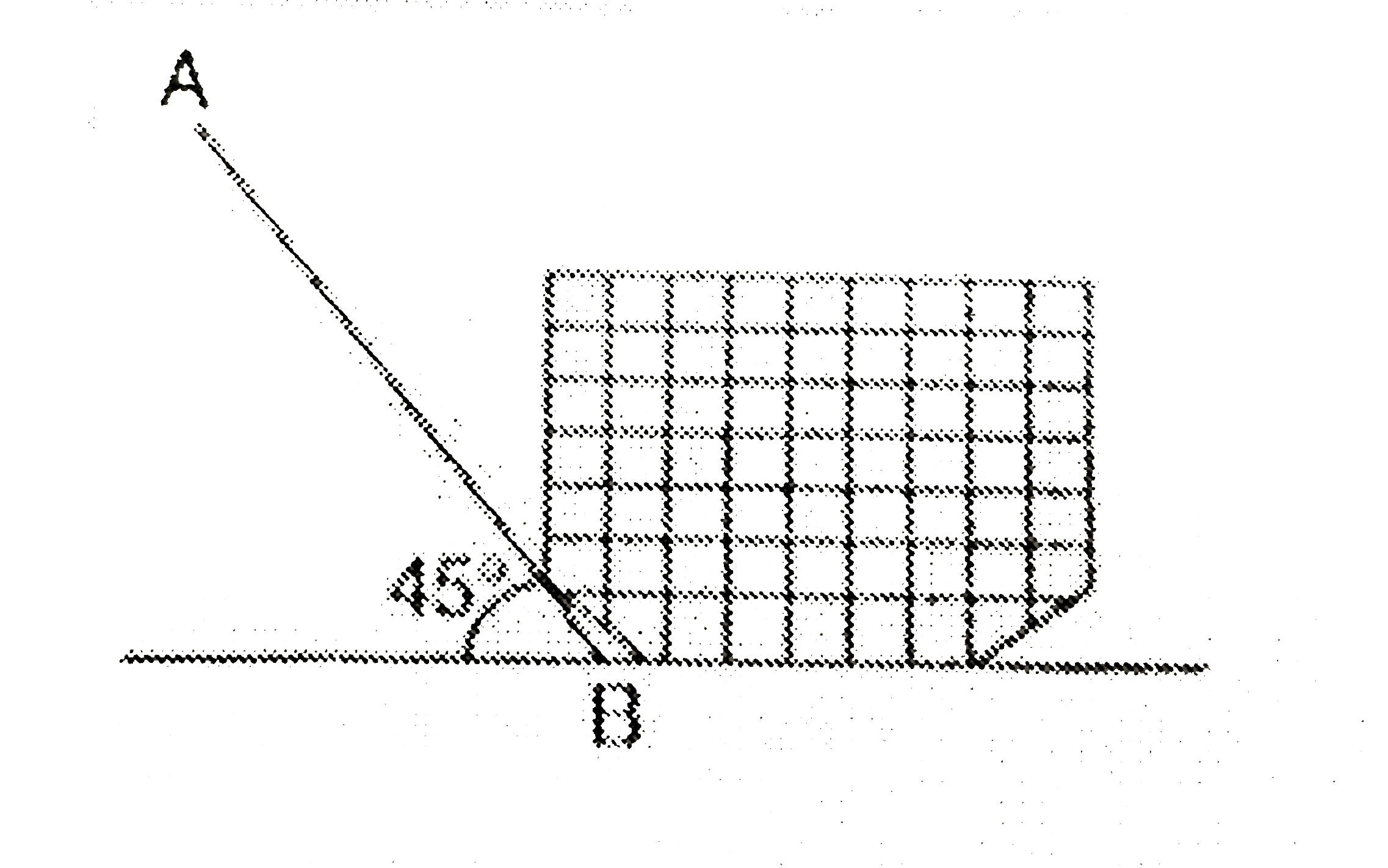
Different forces that act on the crate are shown in the figure
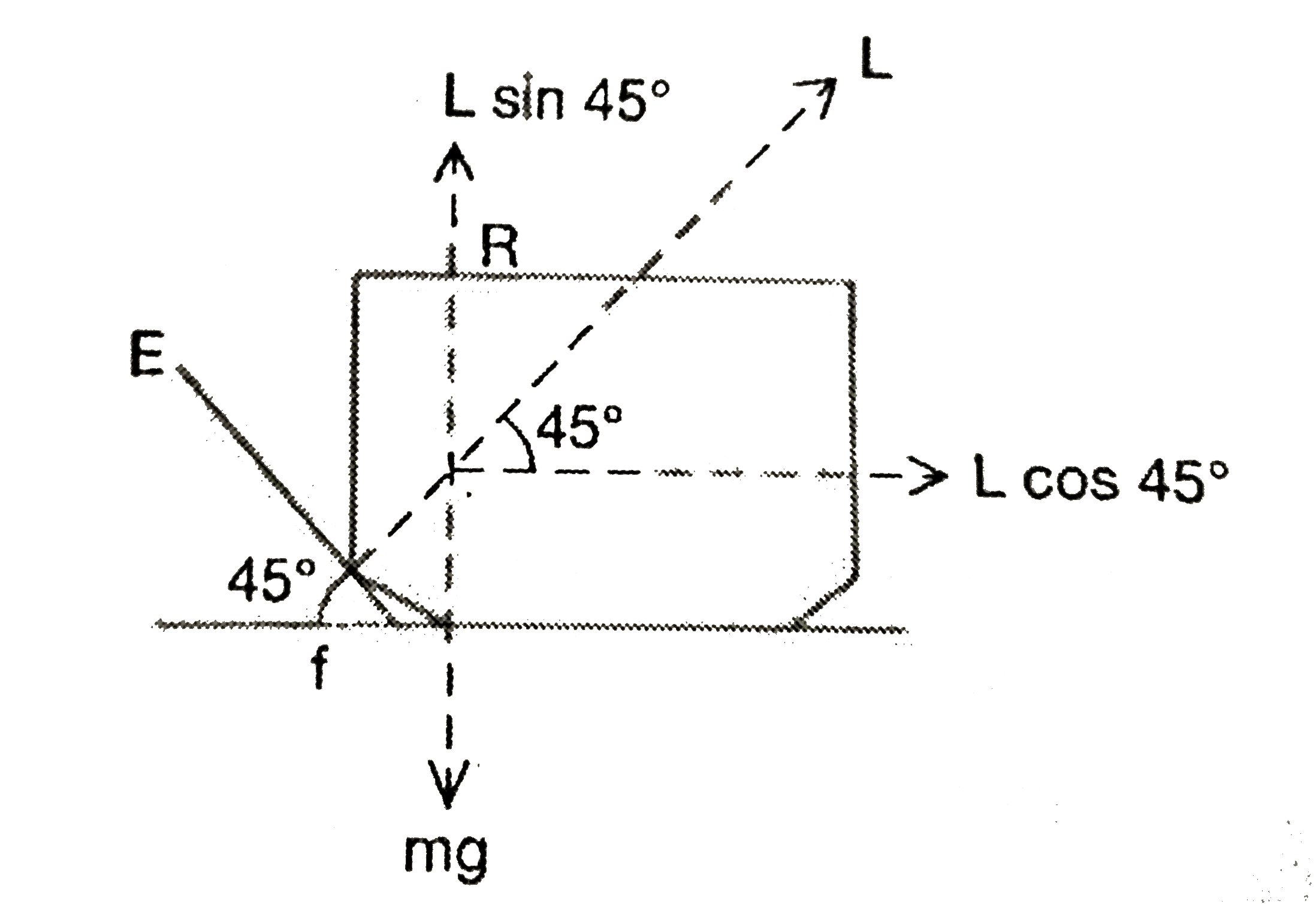
From the figure it is clear that
R + L sin 45 = mg
R=[mg−L√2] and the force that is responsible for the motion of the roller is L cos45∘.
Thus, Lcos45∘=f=μR⇒L√2=μ(mg−L√2)
L√2=μmg.μL√2⇒L√2(μ+1)=μmg
Or L=√2μmgμ+1
Now, (effort) (effort arm) = (load) (load arm)
Effort =L×le=le(√2μmgμ+1)
Given l=100cm,e=100cm,
mg=3000N and μ=sqt(2)
=(10100)(√2×√2×3000√2+1)=250N
Topper's Solved these Questions
- SIMPLE MACHINESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SIMPLE MACHINESEXERCISE - Level- 2
25 Videos
- MODERN PHYSICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MODERN PHYSICSEXERCISE - Level 3
5 Videos
- WAVES MOTION AND SOUND BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - WAVES MOTION AND SOUND EXERCISE - Level 3
10 Videos
Similar Questions
A road roller of 200 kg wt slides on ground when pushed by a lever AB of length 1m, as shown in the figure. The force required to slide the roller acts at a distance of 5cm from the fulcrum. If the coefficient of friction between the roller and the ground is √2 find the effort required to move the roller. (Take g=10ms−2)
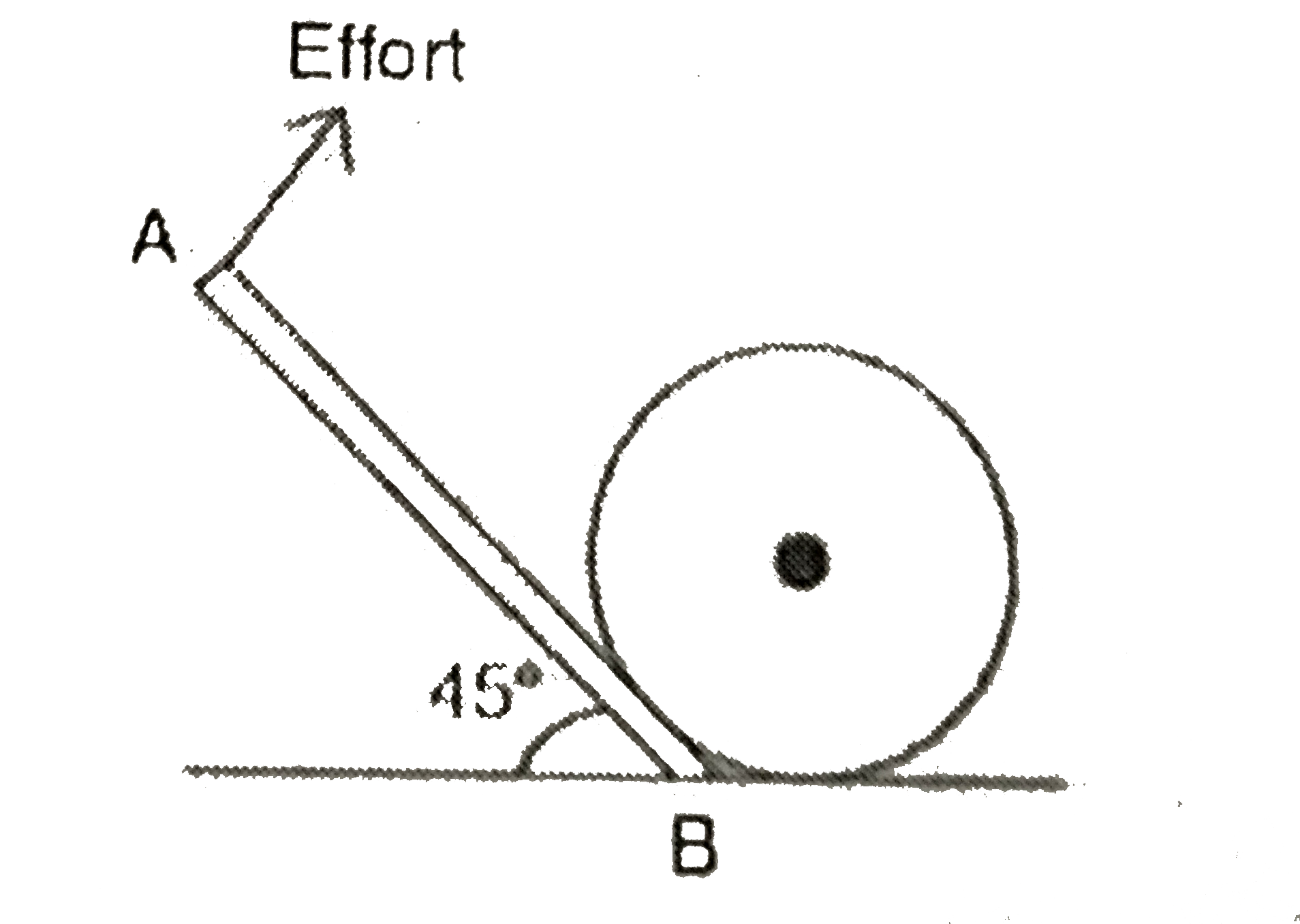
View SolutionA class-three lever has a length of 150 cm and the effort is applied at a distance of 50 cm from the fulcrum. The MA is
View Solution
Knowledge Check
In the above problem, the contact force between the man and the crate is
A2250NB1125NC750ND375NA man drags an m kg crate across a floor by pulling on a rope inclined at angle θ above the horizontal. If the coefficient of static friction between the floor and crate is then the tension required in the rope to start the crate moving is :
Aμsmg(sinθ−μscosθ)Bμsmg(sinθ+μscosθ)Cμsmg(cosθ−μssinθ)Dμsmg(cosθ+μssinθ)If the coefficient of kinetic friction be in above question then the initial acceleration of the crate will be :
A[(Tmg)(cosθ+μksinθ)+μk]gB[(Tmg)(cosθ+μksinθ)−μk]gC[(Tmg)(sinθ+μksinθ)−μk]gD[(Tmg)(sinθ+μksinθ)+μk]g
A crate of mass 20 kg is sliding across a wooden floor. The coefficient of kinetic friction between the crate and the floor is 0.3.
Determine the magnitude of the friction force acting on the crate.
View SolutionA crate of mass m is pulled with a force F along a fixed right angles horizontal trough as in figure. The coefficient of kinetic friction between the crate and the trough is μ. Find the value of force F required to pull it along the trough with constant velocity.
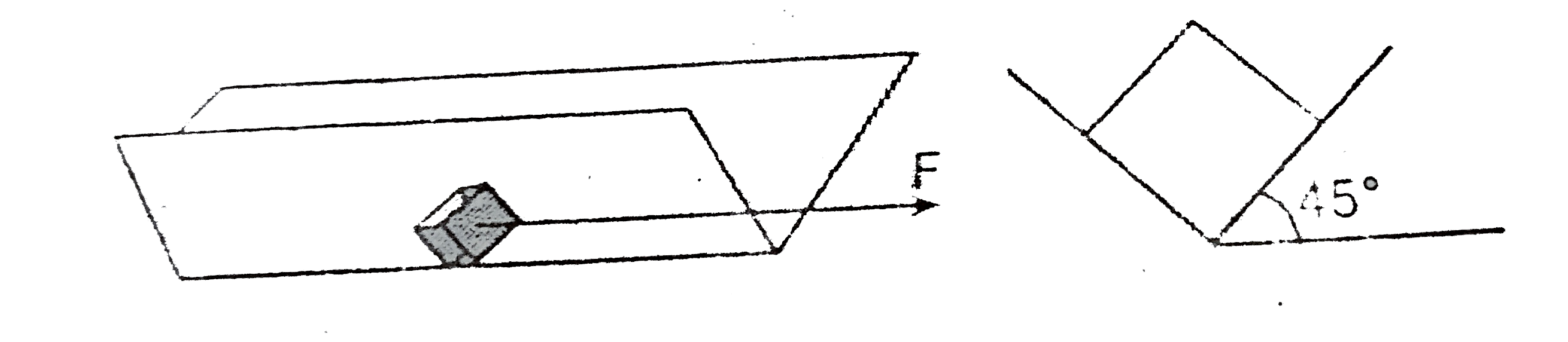
View SolutionA crate of mass m is pulled with a force F along a fixed right angles horizontal trough as in figure. The coefficient of kinetic friction between the crate and the trough is μ. Find the value of force F required to pull it along the trough with constant velocity.

View SolutionA force F is applied on the top of a cube as shown in the figure. The coefficient of friction between the cube and the ground is μ. If F is gradually increased, find the value of μ for which the cube will topple before sliding.
View SolutionA force F is applied on the top of a cube as shown in the figure. The coefficient of friction between the cube and the ground is μ. If F is gradually increased, find the value of μ for which the cube will topple before sliding.

View Solution
